Chapter 10 Vector Algebra EXERCISE 10.1
EXERCISE 10.1
1. Represent graphically a displacement of $40 km, 30^{\circ}$ east of north.
Show Answer
Solution
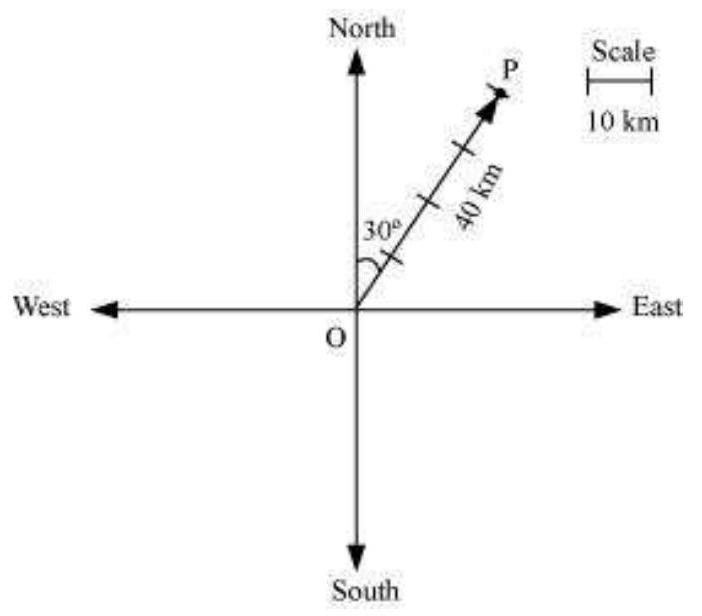
Here, vector $\overrightarrow{{}OP}$ represents the displacement of $40 km, 30^{\circ}$ East of North.
2. Classify the following measures as scalars and vectors.
(i) $10 kg$
(ii) 2 meters north-west
(iii) $40^{\circ}$
(iv) 40 watt
(v) $10^{-19}$ coulomb
(vi) $20 m / s^{2}$
Show Answer
Solution
(i) $10 kg$ is a scalar quantity because it involves only magnitude.
(ii) 2 meters north-west is a vector quantity as it involves both magnitude and direction.
(iii) $40^{\circ}$ is a scalar quantity as it involves only magnitude.
(iv) 40 watts is a scalar quantity as it involves only magnitude.
(v) $10^{-19}$ coulomb is a scalar quantity as it involves only magnitude.
(vi) $20 m / s^{2}$ is a vector quantity as it involves magnitude as well as direction.
3. Classify the following as scalar and vector quantities.
(i) time period
(ii) distance
(iv) velocity
(v) work done
(iii) force
Show Answer
Solution
(i) Time period is a scalar quantity as it involves only magnitude.
(ii) Distance is a scalar quantity as it involves only magnitude.
(iii) Force is a vector quantity as it involves both magnitude and direction.
(iv) Velocity is a vector quantity as it involves both magnitude as well as direction.
(v) Work done is a scalar quantity as it involves only magnitude.
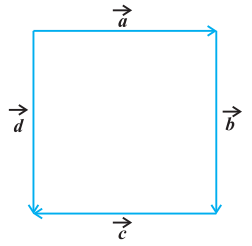
4. In Fig 10.6 (a square), identify the following vectors.
(i) Coinitial
(ii) Equal
(iii) Collinear but not equal
Show Answer
Solution
(i) Vectors $\vec{a}$ and $\vec{d}$ are coinitial because they have the same initial point.
(ii) Vectors $\vec{b}$ and $\vec{d}$ are equal because they have the same magnitude and direction.
(iii) Vectors $\vec{a}$ and $\vec{c}$ are collinear but not equal. This is because although they are parallel, their directions are not the same.
5. Answer the following as true or false.
(i) $\vec{a}$ and $-\vec{a}$ are collinear.
(ii) Two collinear vectors are always equal in magnitude.
(iii) Two vectors having same magnitude are collinear.
Fig 10.6
(iv) Two collinear vectors having the same magnitude are equal.
Show Answer
Solution
(i) True.
Vectors $\vec{a}$ and $-\vec{a}$ are parallel to the same line.
(ii) False.
Collinear vectors are those vectors that are parallel to the same line.
(iii) False.










