Answer :

The given equation of line is

$(k - 3) x - (4 - k^{2}) y+k^{2} $ -$7 k+6=0$

Answer :

Let the intercepts cut by the given lines on the axes be $a$ and $b$.

It is given that

$a+b=1 \ldots(1)$

$a b=- 6$

On solving equations (1) and (2), we obtain

$a=3$ and $b=$ - 2 or $a=-2$ and $b=3$

It is known that the equation of the line whose intercepts on the axes are $a$ and $b$ is

$\dfrac{x}{a}+\dfrac{y}{b}=1$ or $b x+a y-a b=0$

Case I: $a=3$ and $b=$ - 2

In this case, the equation of the line is - $2 x+3 y+6=0$, i.e., $2 x$ - $3 y=6$.

Case II: $a=$ - 2 and $b=3$

In this case, the equation of the line is $3 x $ - $2 y+6=0$, i.e., - $3 x+2 y=6$.

Thus, the required equation of the lines are $2 x $ - $3 y=6$ and $-3 x+2 y=6$.

Answer :

Let $(0, b)$ be the point on the $y$-axis whose distance from line $\dfrac{x}{3}+\dfrac{y}{4}=1$ is 4 units.

The given line can be written as $4 x+3 y$ - $12=0$

On comparing equation (1) to the general equation of line $A x+B y+C=0$, we obtain $A=4, B=3$, and $C= -12$.

It is known that the perpendicular distance $(d)$ of a line $A x+B y+C=0$ from a point $(x_1, y_1)$ is given by

$d=\dfrac{|A x_1+B y_1+C|}{\sqrt{A^{2}+B^{2}}}$.

Therefore, if $(0, b)$ is the point on the $y$-axis whose distance from line $\dfrac{x}{3}+\dfrac{y}{4}=1$ is 4 units, then: $4=\dfrac{|4(0)+3(b)-12|}{\sqrt{4^{2}+3^{2}}}$

$\Rightarrow 4=\dfrac{|3 b-12|}{5}$

$\Rightarrow 20=|3 b-12|$

$\Rightarrow 20= \pm(3 b-12)$

$\Rightarrow 20=(3 b-12)$ or $20=-(3 b-12)$

$\Rightarrow 3 b=20+12$ or $3 b=-20+12$

$\Rightarrow b=\dfrac{32}{3}$ or $b=-\dfrac{8}{3}$

Thus, the required points are $(0, \dfrac{32}{3})$ and $(0,-\dfrac{8}{3})$.

Answer :

The equation of the line joining the points $(\cos \theta, \sin \theta)$ and $(\cos \phi, \sin \phi)$ is given by

$ \begin{aligned} & y-\sin \theta=\dfrac{\sin \phi-\sin \theta}{\cos \phi-\cos \theta}(x-\cos \theta) \\ & y(\cos \phi-\cos \theta)-\sin \theta(\cos \phi-\cos \theta)=x(\sin \phi-\sin \theta)-\cos \theta(\sin \phi-\sin \theta) \\ & x(\sin \theta-\sin \phi)+y(\cos \phi-\cos \theta)+\cos \theta \sin \phi-\cos \theta \sin \theta-\sin \theta \cos \phi+\sin \theta \cos \theta=0 \\ & x(\sin \theta-\sin \phi)+y(\cos \phi-\cos \theta)+\sin (\phi-\theta)=0 \\ & A x+B y+C=0, \text{ where } A=\sin \theta-\sin \phi, B=\cos \phi-\cos \theta, \text{ and } C=\sin (\phi-\theta) \end{aligned} $

It is known that the perpendicular distance $(d)$ of a line $A x+B y+C=0$ from a point $(x_1, y_1)$ is given by $d=\dfrac{|A x_1+B y_1+C|}{\sqrt{A^{2}+B^{2}}}$.

Therefore, the perpendicular distance $(d)$ of the given line from point $(x_1, y_1)=(0,0)$ is

$ \begin{aligned} & d=\dfrac{|(\sin \theta-\sin \phi)(0)+(\cos \phi-\cos \theta)(0)+\sin (\phi-\theta)|}{\sqrt{(\sin \theta-\sin \phi)^{2}+(\cos \phi-\cos \theta)^{2}}} \\ & =\dfrac{|\sin (\phi-\theta)|}{\sqrt{\sin ^{2} \theta+\sin ^{2} \phi-2 \sin \theta \sin \phi+\cos ^{2} \phi+\cos ^{2} \theta-2 \cos \phi \cos \theta}} \\ & =\dfrac{|\sin (\phi-\theta)|}{\sqrt{(\sin ^{2} \theta+\cos ^{2} \theta)+(\sin ^{2} \phi+\cos ^{2} \phi)-2(\sin \theta \sin \phi+\cos \theta \cos \phi)}} \\ & =\dfrac{|\sin (\phi-\theta)|}{\sqrt{1+1-2(\cos (\phi-\theta))}} \\ & =\dfrac{|\sin (\phi-\theta)|}{\sqrt{2(1-\cos (\phi-\theta))}} \\ & =\dfrac{|\sin (\phi-\theta)|}{\sqrt{2(2 \sin ^{2}(\dfrac{\phi-\theta}{2}))}} \\ & =\dfrac{|\sin (\phi-\theta)|}{|2 \sin (\dfrac{\phi-\theta}{2})|} \end{aligned} $

Answer :

The equation of any line parallel to the $y$-axis is of the form

$x=a$

The two given lines are

$x$ - $7 y+5=0$

$3 x+y=0$

On solving equations (2) and (3), we obtain $x=-\dfrac{5}{22}$ and $y=\dfrac{15}{22}$.

Therefore, $(-\dfrac{5}{22}, \dfrac{15}{22})$

is the point of intersection of lines (2) and (3).

Since line $x=a$ passes through point $(-\dfrac{5}{22}, \dfrac{15}{22}), a=-\dfrac{5}{22}$.

Thus, the required equation of the line is $x=-\dfrac{5}{22}$.

Answer :

The equation of the given line is $\dfrac{x}{4}+\dfrac{y}{6}=1$.

This equation can also be written as $3 x+2 y$ - $12=0$

$y=\dfrac{-3}{2} x+6$

, which is of the form $y=m x+c$

$\therefore$ Slope of the given line $=-\dfrac{3}{2}$

$\therefore$ Slope of line perpendicular to the given line

$ =-\dfrac{1}{(-\dfrac{3}{2})}=\dfrac{2}{3} $

Let the given line intersect the $y$-axis at $(0, y)$.

On substituting $x$ with 0 in the equation of the given line, we obtain $\dfrac{y}{6}=1 \Rightarrow y=6$

$\therefore$ The given line intersects the $y$-axis at $(0,6)$.

The equation of the line that has a slope of $\dfrac{2}{3}$ and passes through point $(0,6)$ is

$(y-6)=\dfrac{2}{3}(x-0)$

$3 y-18=2 x$

$2 x-3 y+18=0$

Thus, the required equation of the line is $2 x-3 y+18=0$.

Answer :

The equations of the given lines are

$y$ - $x=0$

$x+y=0$.

$x$ - $k=0$

The point of intersection of lines (1) and (2) is given by

$x=0$ and $y=0$

The point of intersection of lines (2) and (3) is given by

$x=k$ and $y=$ -k

The point of intersection of lines (3) and (1) is given by

$x=k$ and $y=k$

Thus, the vertices of the triangle formed by the three given lines are $(0,0),(k, -k) $, and $(k, k)$.

We know that the area of a triangle whose vertices are $(x_1, y_1),(x_2, y_2)$, and $(x_3, y_3)$ is

$\dfrac{1}{2}|x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2)|$

Therefore, area of the triangle formed by the three given lines

$=\dfrac{1}{2}|0(-k-k)+k(k-0)+k(0+k)|$ square units

$=\dfrac{1}{2}|k^{2}+k^{2}|$ square units

$=\dfrac{1}{2}|2 k^{2}|$ square units

$=k^{2}$ square units

Answer :

The equations of the given lines are

$3 x+y-2=0$

$p x+2 y-3=0$ $2 x-y-3=0$…

On solving equations (1) and (3), we obtain

$x=1$ and $y=-1$

Since these three lines may intersect at one point, the point of intersection of lines (1) and (3) will also satisfy line (2).

$p(1)+2(-1)-3=0$

$p-2-3=0$

$p=5$

Thus, the required value of $p$ is 5 .

Answer :

The equations of the given lines are

$y=m_1 x+c_1 \ldots(1)$

$y=m_2 x+c_2 \ldots$

$y=m_3 x+c_3$.

On subtracting equation (1) from (2), we obtain

$0=(m_2-m_1) x+(c_2-c_1)$

$\Rightarrow(m_1-m_2) x=c_2-c_1$

$\Rightarrow x=\dfrac{c_2-c_1}{m_1-m_2}$

On substituting this value of $x$ in (1), we obtain

$ \begin{aligned} & y=m_1(\dfrac{c_2-c_1}{m_1-m_2})+c_1 \\ & y=\dfrac{m_1 c_2-m_1 c_1}{m_1-m_2}+c_1 \\ & y=\dfrac{m_1 c_2-m_1 c_1+m_1 c_1-m_2 c_1}{m_1-m_2} \\ & y=\dfrac{m_1 c_2-m_2 c_1}{m_1-m_2} \end{aligned} $

$ \therefore(\dfrac{c_2-c_1}{m_1-m_2}, \dfrac{m_1 c_2-m_2 c_1}{m_1-m_2}) \text{ is the point of intersection of lines (1) and (2). } $

It is given that lines (1), (2), and (3) are concurrent. Hence, the point of intersection of lines (1) and (2) will also satisfy equation (3).

$ \begin{aligned} & \dfrac{m_1 c_2-m_2 c_1}{m_1-m_2}=m_3(\dfrac{c_2-c_1}{m_1-m_2})+c_3 \\ & \dfrac{m_1 c_2-m_2 c_1}{m_1-m_2}=\dfrac{m_3 c_2-m_3 c_1+c_3 m_1-c_3 m_2}{m_1-m_2} \\ & m_1 c_2-m_2 c_1-m_3 c_2+m_3 c_1-c_3 m_1+c_3 m_2=0 \\ & m_1(c_2-c_3)+m_2(c_3-c_1)+m_3(c_1-c_2)=0 \\ & \text{ Hence, } m_1(c_2-c_3)+m_2(c_3-c_1)+m_3(c_1-c_2)=0 . \end{aligned} $

Answer :

Let the slope of the required line be $m_1$.

The given line can be written as $y=\dfrac{1}{2} x-\dfrac{3}{2}$, which is of the form $y=m x+c$

$\therefore$ Slope of the given line $=m_2=\dfrac{1}{2}$

It is given that the angle between the required line and line $x - 2 y=3$ is $45^{\circ}$.

We know that if $\theta$ isthe acute angle between lines $I_1$ and $I_2$ with slopes $m_1$ and $m_2$ respectively, then

$\tan \theta=|\dfrac{m_2-m_1}{1+m_1 m_2}|$ $\therefore \tan 45^{\circ}=\dfrac{|m_1-m_2|}{1+m_1 m_2}$

$\Rightarrow I=|\dfrac{\dfrac{1}{2}-m_1}{1+\dfrac{m_1}{2}}|$

$\Rightarrow 1=|\dfrac{(\dfrac{1-2 m_1}{2})}{\dfrac{2+m_1}{2}}|$

$\Rightarrow 1=|\dfrac{1-2 m_1}{2+m_1}|$

$\Rightarrow 1= \pm(\dfrac{1-2 m_1}{2+m_1})$

$\Rightarrow 1=\dfrac{1-2 m_1}{2+m_1}$ or $1=-(\dfrac{1-2 m_1}{2+m_1})$

$\Rightarrow 2+m_1=1-2 m_1$ or $2+m_1=-1+2 m_1$

$\Rightarrow m_1=-\dfrac{1}{3}$ or $m_1=3$

Case I: $m_1=3$

The equation of the line passing through $(3,2)$ and having a slope of 3 is:

$y$ - 2 = $3(x$ - 3 $)$

$y$ - $2=3 x$ - 9

$3 x -y=7$

Case II: $m_1=-\dfrac{1}{3}$

The equation of the line passing through $(3,2)$ and having a slope of $-\dfrac{1}{3}$ is:

$y-2=-\dfrac{1}{3}(x-3)$

$3 y-6=-x+3$

$x+3 y=9$

Thus, the equations of the lines are $3 x$ - $y=7$ and $x+3 y=9$.

Answer :

Let the equation of the line having equal intercepts on the axes be

$\dfrac{x}{a}+\dfrac{y}{a}=1$

Or $x+y=a$

On solving equations $4 x+7 y - 3=0$ and $2 x $ - $3 y+1=0$, we obtain $x=\dfrac{1}{13}$ and $y=\dfrac{5}{13}$.

$\therefore(\dfrac{1}{13}, \dfrac{5}{13})$ is the point of intersection of the two given lines.

Since equation (1) passes through point $(\dfrac{1}{13}, \dfrac{5}{13})$,

$\dfrac{1}{13}+\dfrac{5}{13}=a$

$\Rightarrow a=\dfrac{6}{13}$

$\therefore$ Equation (1) becomes

$ x+y=\dfrac{6}{13} \text{, i.e., } 13 x+13 y=6 $

Thus, the required equation of the line is $13 x+13 y=6$

Answer :

Let the equation of the line passing through the origin be $y=m_1 x$.

If this line makes an angle of $\theta$ with line $y=m x+c$, then angle $\theta$ is given by $\therefore \tan \theta=|\dfrac{m_1-m}{1+m_1 m}|$

$\Rightarrow \tan \theta=|\dfrac{\dfrac{y}{x}-m}{1+\dfrac{y}{x} m}|$

$\Rightarrow \tan \theta= \pm(\dfrac{\dfrac{y}{x}-m}{1+\dfrac{y}{x} m})$

$\Rightarrow \tan \theta=\dfrac{\dfrac{y}{x}-m}{1+\dfrac{y}{x} m}$ or $\tan \theta=-(\dfrac{\dfrac{y}{x}-m}{1+\dfrac{y}{x} m})$

Case I: $\quad \tan \theta=\dfrac{\dfrac{y}{x}-m}{1+\dfrac{y}{x} m}$

$ \tan \theta=\dfrac{\dfrac{y}{x}-m}{1+\dfrac{y}{x} m} $

$\Rightarrow \tan \theta+\dfrac{y}{x} m \tan \theta=\dfrac{y}{x}-m$

$\Rightarrow m+\tan \theta=\dfrac{y}{x}(1-m \tan \theta)$

$\Rightarrow \dfrac{y}{x}=\dfrac{m+\tan \theta}{1-m \tan \theta}$

Case II:

$ \tan \theta=-(\dfrac{\dfrac{y}{x}-m}{1+\dfrac{y}{x} m}) $

$\tan \theta=-(\dfrac{\dfrac{y}{x}-m}{1+\dfrac{y}{x} m})$

$\Rightarrow \tan \theta+\dfrac{y}{x} m \tan \theta=-\dfrac{y}{x}+m$

$\Rightarrow \dfrac{y}{x}(1+m \tan \theta)=m-\tan \theta$

$\Rightarrow \dfrac{y}{x}=\dfrac{m-\tan \theta}{1+m \tan \theta}$

Therefore, the required line is given by $\dfrac{y}{x}=\dfrac{m \pm \tan \theta}{1 \mp m \tan \theta}$

Answer :

The equation of the line joining the points ( $-1,1)$ and $(5,7)$ is given by

$y-1=\dfrac{7-1}{5+1}(x+1)$

$y-1=\dfrac{6}{6}(x+1)$

$x-y+2=0$

The equation of the given line is

$x +y -4 =0$

The point of intersection of lines (1) and (2) is given by

$x=1$ and $y=3$

Let point $(1,3)$ divide the line segment joining $(- 1,1)$ and $(5,7)$ in the ratio $1: k$. Accordingly, by section formula, $(1,3)=(\dfrac{k(-1)+1(5)}{1+k}, \dfrac{k(1)+1(7)}{1+k})$

$\Rightarrow(1,3)=(\dfrac{-k+5}{1+k}, \dfrac{k+7}{1+k})$

$\Rightarrow \dfrac{-k+5}{1+k}=1, \dfrac{k+7}{1+k}=3$

$\therefore \dfrac{-k+5}{1+k}=1$

$\Rightarrow-k+5=1+k$

$\Rightarrow 2 k=4$

$\Rightarrow k=2$

Thus, the line joining the points $( -1,1)$ and $(5,7)$ is divided by line $x+y=4$ in the ratio $1: 2$.

Answer :

The given lines are

$2 x - y=0$…

$4 x+7 y+5=0 \ldots$

A $(1,2)$ is a point on line (1).

Let $B$ be the point of intersection of lines (1) and (2).

On solving equations (1) and (2), we obtain

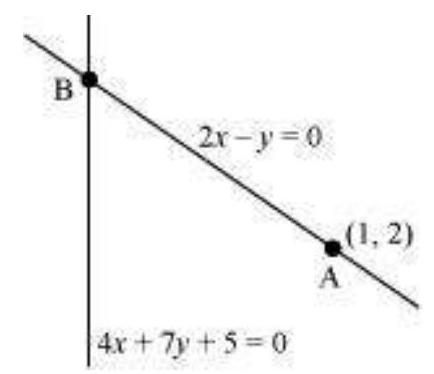
$ x=\dfrac{-5}{18} \text{ and } y=\dfrac{-5}{9} $

$\therefore$ Coordinates of point $B$ are $(\dfrac{-5}{18}, \dfrac{-5}{9})$.

By using distance formula, the distance between points $A$ and $B$ can be obtained as

$AB=\sqrt{(1+\dfrac{5}{18})^{2}+(2+\dfrac{5}{9})^{2}}$ units

$=\sqrt{(\dfrac{23}{18})^{2}+(\dfrac{23}{9})^{2}}$ units

$=\sqrt{(\dfrac{23}{2 \times 9})^{2}+(\dfrac{23}{9})^{2}}$ units

$=\sqrt{(\dfrac{23}{9})^{2}(\dfrac{1}{2})^{2}+(\dfrac{23}{9})^{2}}$ units

$=\sqrt{(\dfrac{23}{9})^{2}(\dfrac{1}{4}+1)}$ units

$=\dfrac{23}{9} \sqrt{\dfrac{5}{4}}$ units

$=\dfrac{23}{9} \times \dfrac{\sqrt{5}}{2}$ units

$=\dfrac{23 \sqrt{5}}{18}$ units

Thus, the required distance is $\dfrac{23 \sqrt{5}}{18}$ units

Answer :

Let $y=m x+c$ be the line through point $($ - 1,2$)$.

Accordingly, $2=m(- 1)+c$.

$\Rightarrow 2=- m+c$

$\Rightarrow c=m+2$

$\therefore y=m x+m+2$

The given line is

$x+y=4$.

On solving equations (1) and (2), we obtain

$ \begin{aligned} & x=\dfrac{2-m}{m+1} \text{ and } y=\dfrac{5 m+2}{m+1} \\ & \therefore(\dfrac{2-m}{m+1}, \dfrac{5 m+2}{m+1}) \text{ is the point of intersection of lines (1) and (2). } \end{aligned} $

Since this point is at a distance of 3 units from point (- 1,2 ), according to distance formula,

$ \begin{aligned} & \sqrt{(\dfrac{2-m}{m+1}+1)^{2}+(\dfrac{5 m+2}{m+1}-2)^{2}}=3 \\ \Rightarrow & (\dfrac{2-m+m+1}{m+1})^{2}+(\dfrac{5 m+2-2 m-2}{m+1})^{2}=3^{2} \\ \Rightarrow & \dfrac{9}{(m+1)^{2}}+\dfrac{9 m^{2}}{(m+1)^{2}}=9 \\ \Rightarrow & \dfrac{1+m^{2}}{(m+1)^{2}}=1 \\ \Rightarrow & 1+m^{2}=m^{2}+1+2 m \\ \Rightarrow & 2 m=0 \\ \Rightarrow & m=0 \end{aligned} $

Thus, the slope of the required line must be zero i.e., the line must be parallel to the $x$-axis.

Answer :

Let $A(1,3)$ and $B(-4,1)$ be the coordinates of the end points of the hypotenuse.

Now, plotting the line segment joining the points $A(1,3)$ and $B(-4,1)$ on the coordinate plane, we will get two right triangles with $A B$ as the hypotenuse. Now from the diagram, it is clear that the point of intersection of the other two legs of the right triangle having $A B$ as the hypotenuse can be either $P$ or $Q$.

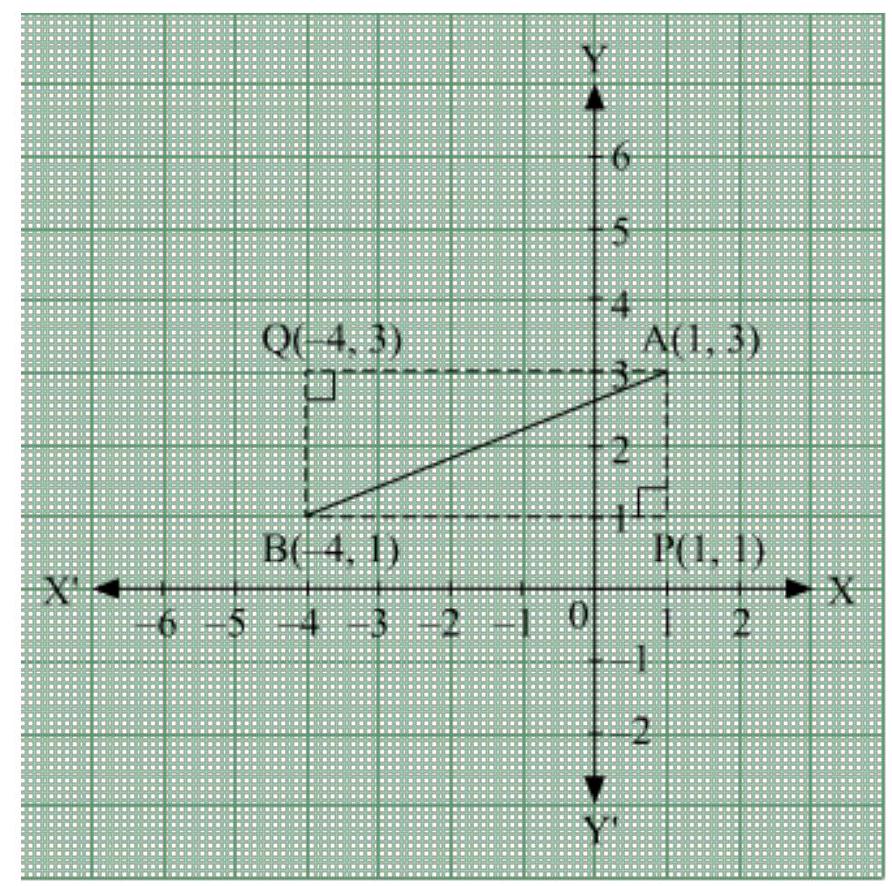
CASE 1: When $\angle $ APB is taken.

The perpendicular sides in $\angle $ APB are AP and PB.

Now, side $P B$ is parallel to $x$-axis and at a distance of 1 units above $x$-axis.

So, equation of $P B$ is, $y=1$ or $y-1=0$.

The side AP is parallel to $y$-axis and at a distance of 1 units on the right of $y$-axis.

So, equation of $A P$ is $x=1$ or $x-1=0$.

CASE 2: When $\angle $ AQB is taken.

The perpendicular sides in $\angle $ AQB are AQ and QB.

Now, side $A Q$ is parallel to $x$-axis and at a distance of 3 units above $x$-axis.

So, equation of $A Q$ is, $y=3$ or $y-3=0$.

The side QB is parallel to $y$-axis and at a distance of 4 units on the left of $y$-axis.

So, equation of $QB$ is $x=-4$ or $x+4=0$.

Hence, the equation of the legs are :

$x=1, y=1$ or $x=-4, y=3$

Answer :

The equation of the given line is

$x+3 y=7$

Let point $B(a, b)$ be the image of point $A(3,8)$.

Accordingly, line (1) is the perpendicular bisector of $A B$.

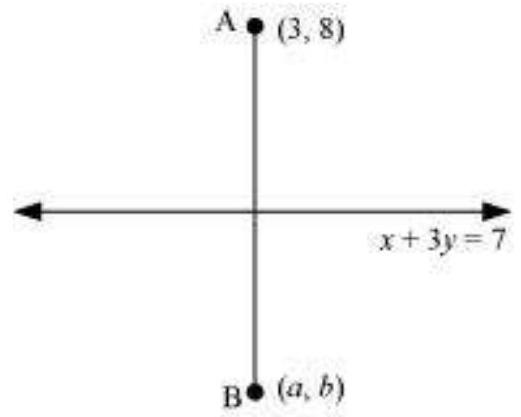
Slope of $AB=\dfrac{b-8}{a-3}$, while the slope of line $(1)=-\dfrac{1}{3}$

Since line (1) is perpendicular to $A B$,

$$ \begin{align*} & (\dfrac{b-8}{a-3}) \times(-\dfrac{1}{3})=-1 \\ & \Rightarrow \dfrac{b-8}{3 a-9}=1 \\ & \Rightarrow b-8=3 a-9 \\ & \Rightarrow 3 a-b=1 \tag{2} \end{align*} $$

Mid-point of $AB=(\dfrac{a+3}{2}, \dfrac{b+8}{2})$

The mid-point of line segment $A B$ will also satisfy line (1).

Hence, from equation (1), we have

$(\dfrac{a+3}{2})+3(\dfrac{b+8}{2})=7$

$\Rightarrow a+3+3 b+24=14$

$\Rightarrow a+3 b=-13$

On solving equations (2) and (3), we obtain $a=$ - 1 and $b=$ -4.

Thus, the image of the given point with respect to the given line is (-1, -4).

Answer :

The equations of the given lines are

$y=3 x+1 \ldots(1)$

$2 y=x+3$ $y=m x+4 \ldots(3)$

Slope of line (1), $m_1=3$

Slope of line (2), $m_2=\dfrac{1}{2}$

Slope of line (3), $m_3=m$

It is given that lines (1) and (2) are equally inclined to line (3). This means that the angle between lines (1) and (3) equals the angle between lines (2) and (3).

$\therefore|\dfrac{m_1-m_3}{1+m_1 m_3}|=|\dfrac{m_2-m_3}{1+m_2 m_3}|$

$\Rightarrow|\dfrac{3-m}{1+3 m}|=|\dfrac{\dfrac{1}{2}-m}{1+\dfrac{1}{2} m}|$

$\Rightarrow|\dfrac{3-m}{1+3 m}|=|\dfrac{1-2 m}{m+2}|$

$\Rightarrow \dfrac{3-m}{1+3 m}= \pm(\dfrac{1-2 m}{m+2})$

$\Rightarrow \dfrac{3-m}{1+3 m}=\dfrac{1-2 m}{m+2}$ or $\dfrac{3-m}{1+3 m}=-(\dfrac{1-2 m}{m+2})$

If $\dfrac{3-m}{1+3 m}=\dfrac{1-2 m}{m+2}$, then

$(3-m)(m+2)=(1-2 m)(1+3 m)$

$\Rightarrow-m^{2}+m+6=1+m-6 m^{2}$

$\Rightarrow 5 m^{2}+5=0$

$\Rightarrow(m^{2}+1)=0$

$\Rightarrow m=\sqrt{-1}$, which is not real

Hence, this case is not posible.

If $\dfrac{3-m}{1+3 m}=-(\dfrac{1-2 m}{m+2})$, then

$\Rightarrow(3-m)(m+2)=-(1-2 m)(1+3 m)$

$\Rightarrow-m^{2}+m+6=-(1+m-6 m^{2})$

$\Rightarrow 7 m^{2}-2 m-7=0$

$\Rightarrow m=\dfrac{2 \pm \sqrt{4-4(7)(-7)}}{2(7)}$

$\Rightarrow m=\dfrac{2 \pm 2 \sqrt{1+49}}{14}$

$\Rightarrow m=\dfrac{1 \pm 5 \sqrt{2}}{7}$

Thus, the required value of $m$ is $\dfrac{1 \pm 5 \sqrt{2}}{7}$.

Answer :

The equations of the given lines are

$x+y$ - $5=0$

$3 x$ - $2 y+7=0$

The perpendicular distances of $P(x, y)$ from lines (1) and (2) are respectively given by

$ d_1=\dfrac{|x+y-5|}{\sqrt{(1)^{2}+(1)^{2}}} \text{ and } d_2=\dfrac{|3 x-2 y+7|}{\sqrt{(3)^{2}+(-2)^{2}}} $

i.e., $d_1=\dfrac{|x+y-5|}{\sqrt{2}}$ and $d_2=\dfrac{|3 x-2 y+7|}{\sqrt{13}}$

It is given that $d_1+d_2=10$ $\therefore \dfrac{|x+y-5|}{\sqrt{2}}+\dfrac{|3 x-2 y+7|}{\sqrt{13}}=10$

$\Rightarrow \sqrt{13}|x+y-5|+\sqrt{2}|3 x-2 y+7|-10 \sqrt{26}=0$

$\Rightarrow \sqrt{13}(x+y-5)+\sqrt{2}(3 x-2 y+7)-10 \sqrt{26}=0$

[Assuming $(x+y-5)$ and $(3 x-2 y+7)$ are positive]

$\Rightarrow \sqrt{13} x+\sqrt{13} y-5 \sqrt{13}+3 \sqrt{2} x-2 \sqrt{2} y+7 \sqrt{2}-10 \sqrt{26}=0$

$\Rightarrow x(\sqrt{13}+3 \sqrt{2})+y(\sqrt{13}-2 \sqrt{2})+(7 \sqrt{2}-5 \sqrt{13}-10 \sqrt{26})=0$

which is the equation of a line.

Similarly, we can obtain the equation of line for any signs of $(x+y-5)$ and $(3 x-2 y+7)$.

Thus, point $P$ must move on a line.

Answer :

The equations of the given lines are

$9 x+6 y - 7=0$

$3 x+2 y+6=0$…

Let $P(h, k)$ be the arbitrary point that is equidistant from lines (1) and (2). The perpendicular distance of $P(h, k)$ from line (1) is given by

$ d_1=\dfrac{|9 h+6 k-7|}{(9)^{2}+(6)^{2}}=\dfrac{|9 h+6 k-7|}{\sqrt{117}}=\dfrac{|9 h+6 k-7|}{3 \sqrt{13}} $

The perpendicular distance of $P(h, k)$ from line (2) is given by

$ d_2=\dfrac{|3 h+2 k+6|}{\sqrt{(3)^{2}+(2)^{2}}}=\dfrac{|3 h+2 k+6|}{\sqrt{13}} $

Since $P(h, k)$ is equidistant from lines (1) and (2), $d_1=d_2$

$$ \begin{aligned} & \therefore \dfrac{|9 h+6 k-7|}{3 \sqrt{13}}=\dfrac{|3 h+2 k+6|}{\sqrt{13}} \\ & \Rightarrow 9 h+6 k-7|=3| 3 h+2 k+6 \mid \\ & \Rightarrow 9 h+6 k-7 \mid= \pm 3(3 h+2 k+6) \\ & \Rightarrow 9 h+6 k-7=3(3 h+2 k+6) \text{ or } 9 h+6 k-7=-3(3 h+2 k+6) \end{aligned} $$

The case $9 h+6 k-7=3(3 h+2 k+6)$ is not possible as

$9 h+6 k-7=3(3 h+2 k+6) \Rightarrow-7=18$ (which is absurd)

$\therefore h+6 k-7=-3(3 h+2 k+6)$

$9h + 6k -7 = -9h-6k-18$

$\Rightarrow 18 h+12 k+11=0$

Thus, the required equation of the line is $18 x+12 y+11=0$.

Answer :

$9h + 6k -7 = -9h-6k-18$

Let the coordinates of point $A$ be $(a, 0)$.

Draw a line (AL) perpendicular to the $x$-axis.

We know that angle of incidence is equal to angle of reflection. Hence, let

$\angle BAL=\angle CAL=\varnothing$

Let $\angle CAX=\theta$

$\therefore \angle OAB=180^{\circ} - (\theta+2 \theta)=180^{\circ} - [\theta+2(90^{\circ} - \theta)]$

$ \begin{aligned} & =180^{\circ} - \theta - 180^{\circ}+2 \theta \\ & =\theta \\ & \therefore \angle B A X=180^{\circ} - \theta \end{aligned} $

Now, slope of line $AC=\dfrac{3-0}{5-a}$

$\Rightarrow \tan \theta=\dfrac{3}{5-a}$

Slope of line $AB=\dfrac{2-0}{1-a}$

$\Rightarrow \tan (180^{\circ}-\theta)=\dfrac{2}{1-a}$

$\Rightarrow-\tan \theta=\dfrac{2}{1-a}$

$\Rightarrow \tan \theta=\dfrac{2}{a-1}$

From equations (1) and (2), we obtain

$ \begin{aligned} & \dfrac{3}{5-a}=\dfrac{2}{a-1} \\ & \Rightarrow 3 a-3=10-2 a \\ & \Rightarrow a=\dfrac{13}{5} \end{aligned} $

Thus, the coordinates of point $A$ are $(\dfrac{13}{5}, 0)$.

Answer :

The equation of the given line is

$\dfrac{x}{a} \cos \theta+\dfrac{y}{b} \sin \theta=1$

Or, $b x \cos \theta+a y \sin \theta-a b=0$

Length of the perpendicular from point $(\sqrt{a^{2}-b^{2}}, 0)$ to line (1) is

$p_1=\dfrac{|b \cos \theta(\sqrt{a^{2}-b^{2}})+a \sin \theta(0)-a b|}{\sqrt{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta}}=\dfrac{|b \cos \theta \sqrt{a^{2}-b^{2}}-a b|}{\sqrt{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta}}$

Length of the perpendicular from point $(-\sqrt{a^{2}-b^{2}}, 0)$ to line (2) is

$p_2=\dfrac{|b \cos \theta(-\sqrt{a^{2}-b^{2}})+a \sin \theta(0)-a b|}{\sqrt{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta}}=\dfrac{|b \cos \theta \sqrt{a^{2}-b^{2}}+a b|}{\sqrt{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta}}$

On multiplying equations (2) and (3), we obtain

$ \begin{aligned} & p_1 p_2=\dfrac{|b \cos \theta \sqrt{a^{2}-b^{2}}-a b|(b \cos \theta \sqrt{a^{2}-b^{2}}+a b) \mid}{(\sqrt{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta})^{2}} \\ & =\dfrac{|(b \cos \theta \sqrt{a^{2}-b^{2}}-a b)(b \cos \theta \sqrt{a^{2}-b^{2}}+a b)|}{(b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta)} \\ & =\dfrac{|(b \cos \theta \sqrt{a^{2}-b^{2}})^{2}-(a b)^{2}|}{(b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta)} \\ & =\dfrac{|b^{2} \cos ^{2} \theta(a^{2}-b^{2})-a^{2} b^{2}|}{(b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta)} \\ & =\dfrac{|a^{2} b^{2} \cos ^{2} \theta-b^{4} \cos ^{2} \theta-a^{2} b^{2}|}{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta} \\ & =\dfrac{b^{2}|a^{2} \cos ^{2} \theta-b^{2} \cos ^{2} \theta-a^{2}|}{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta} \\ & =\dfrac{b^{2}|a^{2} \cos ^{2} \theta-b^{2} \cos ^{2} \theta-a^{2} \sin ^{2} \theta-a^{2} \cos ^{2} \theta|}{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta} \quad[\sin ^{2} \theta+\cos ^{2} \theta=1] \\ & =\dfrac{b^{2}|-(b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta)|}{b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta} \\ & =\dfrac{b^{2}(b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta)}{(b^{2} \cos ^{2} \theta+a^{2} \sin ^{2} \theta)} \\ & =b^{2} \end{aligned} $

Hence, proved.

Answer :

The equations of the given lines are

$2 x$ - $3 y+4=0$

$3 x+4 y-=0$

$6 x$ - $7 y+8=0$

The person is standing at the junction of the paths represented by lines (1) and (2).

On solving equations (1) and (2), we obtain

$ x=-\dfrac{1}{17} \text{ and } y=\dfrac{22}{17} $

Thus, the person is standing at point $(-\dfrac{1}{17}, \dfrac{22}{17})$.

The person can reach path (3) in the least time if he walks along the perpendicular line to (3) from point $(-\dfrac{1}{17}, \dfrac{22}{17})$

Slope of the line $(3)=\dfrac{6}{7}$

$\therefore$ Slope of the line perpendicular to line (3)

$ =-\dfrac{1}{(\dfrac{6}{7})}=-\dfrac{7}{6} $

The equation of the line passing through $(-\dfrac{1}{17}, \dfrac{22}{17})$ and having a slope of $-\dfrac{7}{6}$ is given by

$ \begin{aligned} & (y-\dfrac{22}{17})=-\dfrac{7}{6}(x+\dfrac{1}{17}) \\ & 6(17 y-22)=-7(17 x+1) \\ & 102 y-132=-119 x-7 \\ & 119 x+102 y=125 \end{aligned} $

Hence, the path that the person should follow is $119 x+102 y=125$.