Answer :

The three vertices of a parallelogram $A B C D$ are given as $ A(3, - $ 1, 2 )$, B(1,2,- 4)$, and C (-1, 1, 2). Let the coordinates of the fourth vertex be $D(x, y, z)$.

We know that the diagonals of a parallelogram bisect each other.

Therefore, in parallelogram $A B C D, A C$ and $B D$ bisect each other.

$\therefore$ Mid-point of $A C=$ Mid-point of $B D$

$\Rightarrow(\dfrac{3-1}{2}, \dfrac{-1+1}{2}, \dfrac{2+2}{2})=(\dfrac{x+1}{2}, \dfrac{y+2}{2}, \dfrac{z-4}{2})$

$\Rightarrow(1,0,2)=(\dfrac{x+1}{2}, \dfrac{y+2}{2}, \dfrac{z-4}{2})$

$\Rightarrow \dfrac{x+1}{2}=1, \dfrac{y+2}{2}=0$, and $\dfrac{z-4}{2}=2$

$\Rightarrow x=1, y=- 2$ , and $z=8$

Thus, the coordinates of the fourth vertex are (1, -2, 8).

Answer :

Let $A D, B E$, and $C F$ be the medians of the given triangle $A B C$.

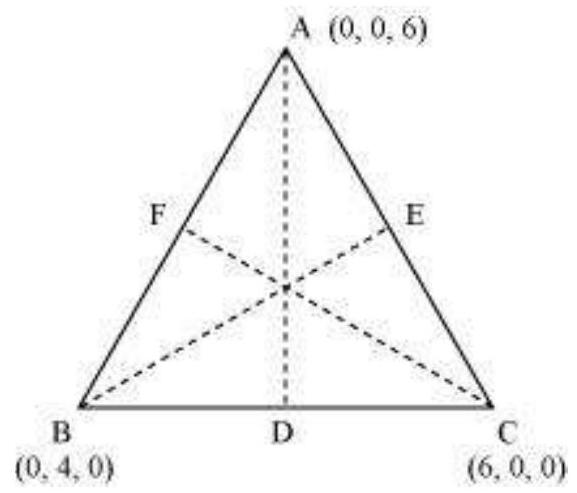
Since $A D$ is the median, $D$ is the mid-point of $B C$.

$\therefore$ Coordinates of point $D=(\dfrac{0+6}{2}, \dfrac{4+0}{2}, \dfrac{0+0}{2})=(3,2,0)$

$AD=\sqrt{(0-3)^{2}+(0-2)^{2}+(6-0)^{2}}=\sqrt{9+4+36}=\sqrt{49}=7$

Since $BE$ is the median, $E$ is the mid-point of $AC$.

$\therefore$ Coordinates of point $E=(\dfrac{0+6}{2}, \dfrac{0+0}{2}, \dfrac{6+0}{2})=(3,0,3)$

$BE=\sqrt{(3-0)^{2}+(0-4)^{2}+(3-0)^{2}}=\sqrt{9+16+9}=\sqrt{34}$

Since $CF$ is the median, $F$ is the mid-point of $AB$.

$\therefore$ Coordinates of point $F=(\dfrac{0+0}{2}, \dfrac{0+4}{2}, \dfrac{6+0}{2})=(0,2,3)$

Length of $C F=\sqrt{(6-0)^{2}+(0-2)^{2}+(0-3)^{2}}=\sqrt{36+4+9}=\sqrt{49}=7$

Thus, the lengths of the medians of $\triangle A B C$ are $7, \sqrt{34}$, and 7

Answer :

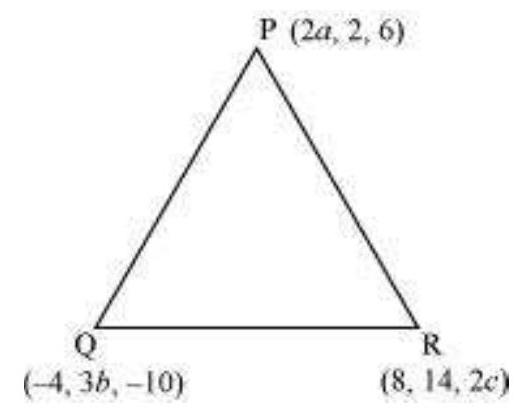
It is known that the coordinates of the centroid of the triangle, whose vertices are $(x_1, y_1, z_1),(x_2, y_2, z_2)$ and $(x_3, y_3, z_3)$, are $(\dfrac{x_1+x_2+x_3}{3}, \dfrac{y_1+y_2+y_3}{3}, \dfrac{z_1+z_2+z_3}{3})$

Therefore, coordinates of the centroid of

$\Delta PQR=(\dfrac{2 a-4+8}{3}, \dfrac{2+3 b+14}{3}, \dfrac{6-10+2 c}{3})=(\dfrac{2 a+4}{3}, \dfrac{3 b+16}{3}, \dfrac{2 c-4}{3})$

It is given that origin is the centroid of $\triangle P Q R$.

$\therefore(0,0,0)=(\dfrac{2 a+4}{3}, \dfrac{3 b+16}{3}, \dfrac{2 c-4}{3})$

$\Rightarrow \dfrac{2 a+4}{3}=0, \dfrac{3 b+16}{3}=0$ and $\dfrac{2 c-4}{3}=0$

$\Rightarrow a=-2, b=-\dfrac{16}{3}$ and $c=2$

Thus, the respective values of $a, b$, and $c$ are

$ -2,-\dfrac{16}{3}, \text{ and } 2 \text{. } $

Answer :

The coordinates of points A and B are given as $(3,4,5)$ and $( -1,3,-7 )$ respectively.

Let the coordinates of point $P$ be $(x, y, z)$.

On using distance formula, we obtain

$ \begin{aligned} PA^{2} & =(x-3)^{2}+(y-4)^{2}+(z-5)^{2} \\ & =x^{2}+9-6 x+y^{2}+16-8 y+z^{2}+25-10 z \\ & =x^{2}-6 x+y^{2}-8 y+z^{2}-10 z+50 \\ PB^{2} & =(x+1)^{2}+(y-3)^{2}+(z+7)^{2} \\ & =x^{2}+2 x+y^{2}-6 y+z^{2}+14 z+59 \end{aligned} $

Now, if $PA^{2}+PB^{2}=k^{2}$, then

$ \begin{aligned} & (x^{2}-6 x+y^{2}-8 y+z^{2}-10 z+50)+(x^{2}+2 x+y^{2}-6 y+z^{2}+14 z+59)=k^{2} \\ & \Rightarrow 2 x^{2}+2 y^{2}+2 z^{2}-4 x-14 y+4 z+109=k^{2} \\ & \Rightarrow 2(x^{2}+y^{2}+z^{2}-2 x-7 y+2 z)=k^{2}-109 \\ & \Rightarrow x^{2}+y^{2}+z^{2}-2 x-7 y+2 z=\dfrac{k^{2}-109}{2} \\ & \qquad x^{2}+y^{2}+z^{2}-2 x-7 y+2 z=\dfrac{k^{2}-109}{2} . \end{aligned} $