Unit 1 The Solid State-Deleted
From our earlier studies, we know that liquids and gases are called fluids because of their ability to flow. The fluidity in both of these states is due to the fact that the molecules are free to move about. On the contrary, the constituent particles in solids have fixed positions and can only oscillate about their mean positions. This explains the rigidity in solids. These properties depend upon the nature of constituent particles and the binding forces operating between them. The correlation between structure and properties helps in the discovery of new solid materials with desired properties. For example, carbon nanotubes are new materials that have potential to provide material that are tougher than steel, lighter than aluminium and have more conductive property than copper. Such materials may play an expanding role in future development of science and society. Some other materials which are expected to play an important role in future are high temperature superconductors, magnetic materials, biodegradable polymers for packaging, biocompliant solids for surgical implants, etc. Thus, the study of this state becomes more important in the present scenario.
In this Unit, we shall discuss different possible arrangements of particles resulting in several types of structures and explore why different arrangements of structural units lend different properties to solids. We will also learn how these properties get modified due to the structural imperfections or by the presence of impurities in minute amounts.
1.1 General Characteristics of Solid State
In Class XI you have learnt that matter can exist in three states namely, solid, liquid and gas. Under a given set of conditions of temperature and pressure, which of these would be the most stable state of a given substance depends upon the net effect of two opposing factors. These are intermolecular forces which tend to keep the molecules (or atoms or ions) closer, and the thermal energy, which tends to keep them apart by making them move faster. At sufficiently low temperature, the thermal energy is low and intermolecular forces bring them so close that they cling to one another and occupy fixed positions. These can still oscillate about their mean positions and the substance exists in solid state.
The following are the characteristic properties of the solid state:
- (i) They have definite mass, volume and shape.
- (ii) Intermolecular distances are short.
- (iii) Intermolecular forces are strong.
- (iv) Their constituent particles (atoms, molecules or ions) have fixed
- positions and can only oscillate about their mean positions.
- (v) They are incompressible and rigid.
1.2 Amorphous and Crystalline Solids
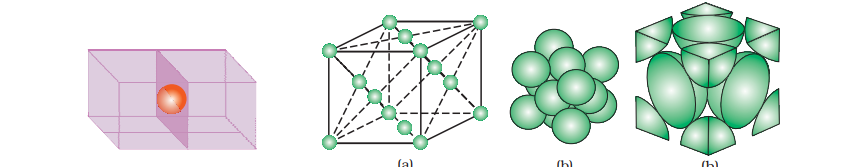
Solids can be classified as crystalline or amorphous on the basis of the nature of order present in the arrangement of their constituent particles. A crystalline solid usually consists of a large number of small crystals, each of them having a definite characteristic geometrical shape. The arrangement of constituent particles (atoms, molecules or ions) in a crystal is ordered and repetitive in three dimensions. If we observe the pattern in one region of the crystal, we can predict accurately the position of particles in any other region of the crystal however far they may be from the place of observation. Thus, crystal has a long range order which means that there is a regular pattern of arrangement of particles which repeats itself periodically over the entire crystal. Sodium chloride and quartz are typical examples of crystalline solids. Glass, rubber and many plastics do not form crystals when their liquids solidify on cooling. These are called amorphous solids. The term amorphous comes from the Greek word amorphos, meaning no form.The arrangement of constituent particles (atoms, molecules or ions) in such a solid has only short range order. In such an arrangement, a regular and periodically repeating pattern is observed over short distances only. Regular patterns are scattered and in between the arrangement is disordered. The structures of quartz (crystalline) and quartz glass (amorphous) are shown in Fig. 1.1 (a) and (b) respectively.
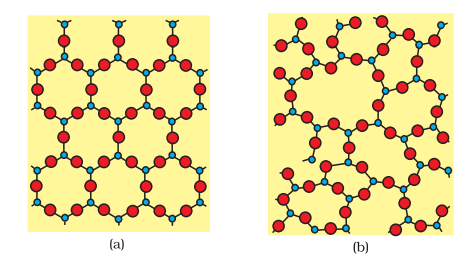
While the two structures are almost identical, yet in the case of amorphous quartz glass there is no long range order. The structure of amorphous solids is similar to that of liquids. Due to the differences in the arrangement of the constituent particles, the two types of solids differ in their properties.
Crystalline solids have a sharp melting point. At a characteristic temperature they melt abruptly and become liquid. On the other hand, amorphous solids soften, melt and start flowing over a range of temperature and can be moulded and blown into various shapes. Amorphous solids have the same structural features as liquids and are conveniently regarded as extremely viscous liquids. They may become crystalline at some temperature. Some glass objects from ancient civilisations are found to become milky in appearance because of some crystallisation. Like liquids, amorphous solids have a tendency to flow, though very slowly. Therefore, sometimes these are called pseudo solids or super cooled liquids.
Amorphous solids are isotropic in nature. Their properties such as mechanical strength, refractive index and electrical conductivity, etc., are same in all directions. It is because there is no long range order in them and arrangement of particles is not definite along all the directions. Hence, the overall arrangement becomes equivalent in all directions. Therefore, value of any physical property would be same along any direction.
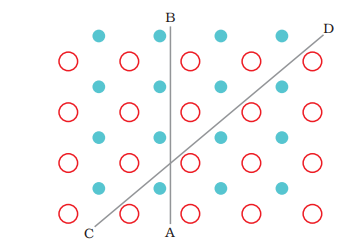
Crystalline solids are anisotropic in nature, that is, some of their physical properties like electrical resistance or refractive index show different values when measured along different directions in the same crystals. This arises from different arrangement of particles in different directions. This is illustrated in Fig. 1.2. This figure shows a simple twodimensional pattern of arrangement of two kinds of atoms. Mechanical property such as resistance to shearing stress might be quite different in two directions indicated in the figure. Deformation in CD direction displaces row which has two different types of atoms while in AB direction rows made of one type of atoms are displaced. The differences between the crystalline solids and amorphous solids are summarised in Table 1.1.

Besides crystalline and amorphous solids, there are some solids which apparently appear amorphous but have microcrystalline structures. These are called polycrystalline solids. Metals often occur in polycrystalline condition. Individual crystals are randomly oriented so a metallic sample may appear to be isotropic even though a single crystal is anisotropic.
Intext Questions
1.1 Why are solids rigid?
Answer The intermolecular forces of attraction that are present in solids are very strong. The constituent particles of solids cannot move from their positions i.e., they have fixed positions. However, they can oscillate about their mean positions. This is the reason solids are rigid.Show Answer
Answer The intermolecular forces of attraction that are present in solids are very strong. The constituent particles of solids have fixed positions i.e., they are rigid. Hence, solids have a definite volume.Show Answer
Answer Amorphous solids Polyurethane, teflon, cellophane, polyvinyl chloride, fibre glass Crystalline solids Naphthalene, benzoic acid, potassium nitrate, copperShow Answer
Show Answer
Answer
An isotropic solid has the same value of physical properties when measured along different directions. Therefore, the given solid, having the same value of refractive index along all directions, is isotropic in nature. Hence, the solid is an amorphous solid.
When an amorphous solid is cut with a sharp edged tool, it cuts into two pieces with irregular surfaces.
1.3 Classification of Crystalline Solids
In Section 1.2, we have learnt about amorphous substances and that they have only short range order. However, most of the solid substances are crystalline in nature. For example, all the metallic elements like iron, copper and silver; non-metallic elements like sulphur, phosphorus and iodine and compounds like sodium chloride, zinc sulphide and naphthalene form crystalline solids.
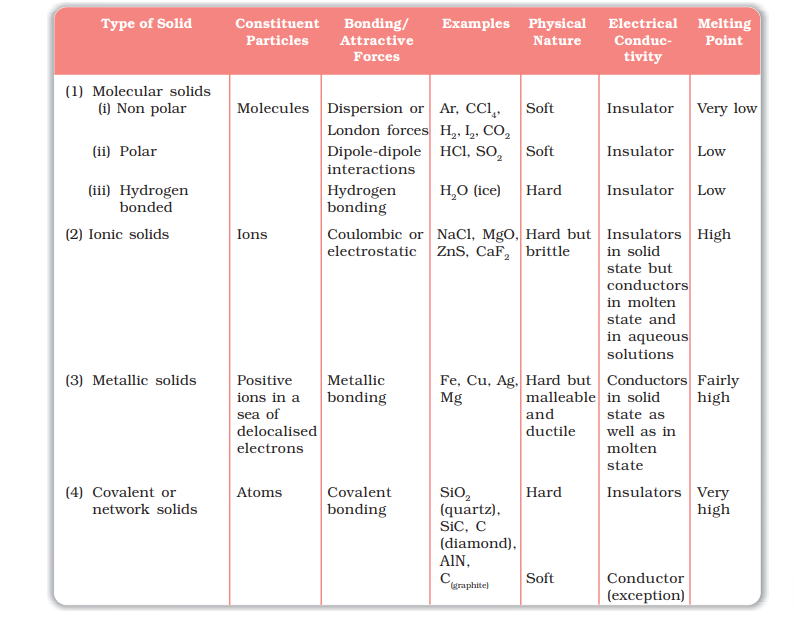
Crystalline solids can be classified in various ways. The method depends on the purpose in hand. Here, we will classify crystalline solids on the basis of nature of intermolecular forces or bonds that hold the constituent particles together. These are — (i) Van der waals forces; (ii) Ionic bonds; (iii) Covalent bonds; and (iv) Metallic bonds. On this basis, crystalline solids are classified into four categories viz., molecular, ionic, metallic and covalent solids. Let us now learn about these categories.
1.3.1 Molecular Solids
Molecules are the constituent particles of molecular solids. These are further sub divided into the following categories:
(i) Non polar Molecular Solids: They comprise either atoms, for example, argon and helium or the molecules formed by non polar covalent bonds, for example, H2, Cl2 and I2. In these solids, the atoms or molecules are held by weak dispersion forces or London forces about which you have learnt in Class XI. These solids are soft and non-conductors of electricity. They have low melting points and are usually in liquid or gaseous state at room temperature and pressure.
(ii) Polar Molecular Solids: The molecules of substances like HCl, SO2, etc. are formed by polar covalent bonds. The molecules in such solids are held together by relatively stronger dipole-dipole interactions. These solids are soft and non-conductors of electricity. Their melting points are higher than those of non polar molecular solids yet most of these are gases or liquids under room temperature and pressure. Solid SO2 and solid NH3 are some examples of such solids.
(iii) Hydrogen Bonded Molecular Solids: The molecules of such solids contain polar covalent bonds between H and F, O or N atoms. Strong hydrogen bonding binds molecules of such solids like H2O (ice). They are non-conductors of electricity. Generally they are volatile liquids or soft solids under room temperature and pressure.
1.3.2 Ionic Solids
Ions are the constituent particles of ionic solids. Such solids are formed by the three dimensional arrangements of cations and anions bound by strong coulombic (electrostatic) forces. These solids are hard and brittle in nature. They have high melting and boiling points. Since the ions are not free to move about, they are electrical insulators in the solid state. However, in the molten state or when dissolved in water, the ions become free to move about and they conduct electricity.
1.3.3 Metallic Solids
Metals are orderly collection of positive ions surrounded by and held together by a sea of free electrons. These electrons are mobile and are evenly spread out throughout the crystal. Each metal atom contributes one or more electrons towards this sea of mobile electrons. These free and mobile electrons are responsible for high electrical and thermal conductivity of metals. When an electric field is applied, these electrons flow through the network of positive ions. Similarly, when heat is supplied to one portion of a metal, the thermal energy is uniformly spread throughout by free electrons. Another important characteristic of metals is their lustre and colour in certain cases. This is also due to the presence of free electrons in them. Metals are highly malleable and ductile.
1.3.4 Covalent or Network Solids

A wide variety of crystalline solids of non-metals result from the formation of covalent bonds between adjacent atoms throughout the crystal. They are also called giant molecules. Covalent bonds are strong and directional in nature, therefore atoms are held very strongly at their positions. Such solids are very hard and brittle. They have extremely high melting points and may even decompose before melting. They are insulators and do not conduct electricity. Diamond (Fig. 1.3) and silicon carbide are typical examples of such solids. Although Graphite (Fig. 1.4) also belongs to this class of crystals, but it is soft and is a conductor of electricity. Its exceptional properties are due to its typical structure. Carbon atoms are arranged in different layers and each atom is covalently bonded to three of its neighbouring atoms in the same layer. The fourth valence electron of each atom is present between different layers and is free to move about. These free electrons make graphite a good conductor of electricity. Different layers can slide one over the other. This makes graphite a soft solid and a good solid lubricant.
Intext Questions
1.5 Classify the following solids in different categories based on the nature of intermolecular forces operating in them: Potassium sulphate, tin, benzene, urea, ammonia, water, zinc sulphide, graphite, rubidium, argon, silicon carbide.
Answer Potassium sulphate $\rightarrow$ Ionic solid Tin $\rightarrow$ Metallic solid Benzene $\rightarrow$ Molecular (non-polar) solid Urea $\rightarrow$ Polar molecular solid Ammonia $\rightarrow$ Polar molecular solid Water $\rightarrow$ Hydrogen bonded molecular solid Zinc sulphide $\rightarrow$ Ionic solid Graphite $\rightarrow$ Covalent or network solid Rubidium $\rightarrow$ Metallic solid Argon $\rightarrow$ Non-polar molecular solid Silicon carbide $\rightarrow$ Covalent or network solidShow Answer
Answer The given properties are the properties of a covalent or network solid. Therefore, the given solid is a covalent or network solid. Examples of such solids include diamond (C) and quartz $\left(\mathrm{SiO}_{2}\right)$.Show Answer
Answer In ionic compounds, electricity is conducted by ions. In solid state, ions are held together by strong electrostatic forces and are not free to move about within the solid. Hence, ionic solids do not conduct electricity in solid state. However, in molten state or in solution form, the ions are free to move and can conduct electricity.Show Answer
Show Answer
Answer
Metallic solids are electrical conductors, malleable, and ductile.
1.4 Crystal Lattices and Unit Cells
You must have noticed that when tiles are placed to cover a floor, a repeated pattern is generated. If after setting tiles on floor we mark a point at same location in all the tiles (e.g. Centre of the tile) and see the marked positions only ignoring the tiles, we obtain a set of points. This set of points is the scaffolding on which pattern has been developed by placing tiles. This scaffolding is a space lattice on which two-dimensional pattern has been developed by placing structural units on its set of points (i.e. tile in this case).

The structural unit is called basis or motif. When motifs are placed on points in space lattice, a pattern is generated. In crystal structure, motif is a molecule, atom or ion. A space lattice, also called a crystal lattice, is the pattern of points representing the locations of these motifs. In other words, space lattice is an abstract scaffolding for crystal structure. When we place motifs in an identical manner on points of space lattice, we get crystal structure. Fig. 1.5 shows a motif, a two-dimensional lattice and a hypothetical two-dimensional crystal structure obtained by placing motifs in the two-dimensional lattice.
Spacial arrangement of lattice points gives rise to different types of lattices. Fig 1.6 shows arrangement of points in two different lattices. In the case of crystalline solids, space lattice is a three-dimensional array of points. The crystal structure is obtained by associating structurral motifs with lattice points. Each repeated basis or motif has same structure and same spacial orientation as other one in a crystal. The environment of each motif is same throughout the crystal except for on surface.
Following are the characteristics of a crystal lattice: (a) Each point in a lattice is called lattice point or lattice site. (b) Each point in a crystal lattice represents one constituent particle which may be an atom, a molecule (group of atoms) or an ion. (c) Lattice points are joined by straight lines to bring out the geometry of the lattice.
We need only a small part of the space lattice of a crystal to spacify crystal completely. This small part is called unit cell. One can choose unit cell in many ways. Normally that cell is chosen which has perpendicular sides of shortest length and one can construct entire crystal by translational displacement of the unit cell in three dimensions. Fig. 1.7 shows movement of unit cell of a two-dimensional lattice to construct the entire crystal structure. Also, unit cells have shapes such that these fill the whole lattice without leaving space between cells.
In two dimensions a parallelogram with side of length ‘a’ and ‘b’ and an angle r between these sides is chosen as unit cell. Possible unit cells in two dimensions are shown in Fig. 1.8.
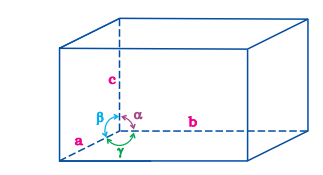
In the three-dimensional crystal structure, unit cell is characterised by:
A portion of three-dimensional crystal lattice and its unit cell is shown in Fig. 1.9.
In the three-dimensional crystal structure, unit cell is characterised by:
(i) its dimensions along the three edges a, b and c. These edges may or may not be mutually perpendicular.
(ii) angles between the edges, a (between b and c), b (between a and c) and g (between a and b). Thus, a unit cell is characterised by six parameters a, b, c, a , b and g. These parameters of a typical unit cell are shown in Fig. 1.6.
1.4.1 Primitive and Centred Unit Cells
Unit cells can be broadly divided into two categories, primitive and centred unit cells.
(a) Primitive Unit Cells When constituent particles are present only on the corner positions of a unit cell, it is called as primitive unit cell.
(b) Centred Unit Cells When a unit cell contains one or more constituent particles present at positions other than corners in addition to those at corners, it is called a centred unit cell. Centred unit cells are of three types:
(i) Body-Centred Unit Cells: Such a unit cell contains one constituent particle (atom, molecule or ion) at its body-centre besides the ones that are at its corners.
(ii) Face-Centred Unit Cells: Such a unit cell contains one constituent particle present at the centre of each face, besides the ones that are at its corners.
(iii) End-Centred Unit Cells: In such a unit cell, one constituent particle is present at the centre of any two opposite faces besides the ones present at its corners.
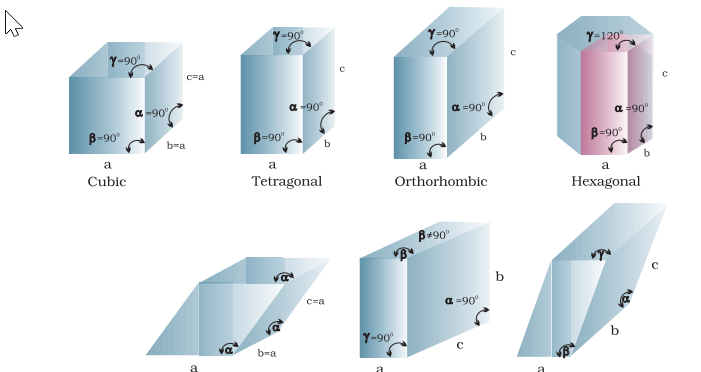
Inspection of a wide variety of crystals leads to the conclusion that all can be regarded as conforming to one of the seven regular figures. These basic regular figures are called seven crystal systems. To which system a given crystal belongs to is determined by measuring the angles between its faces and deciding how many axis are needed to define the principal features of its shape. Fig. 1.11 shows seven crystal systems.
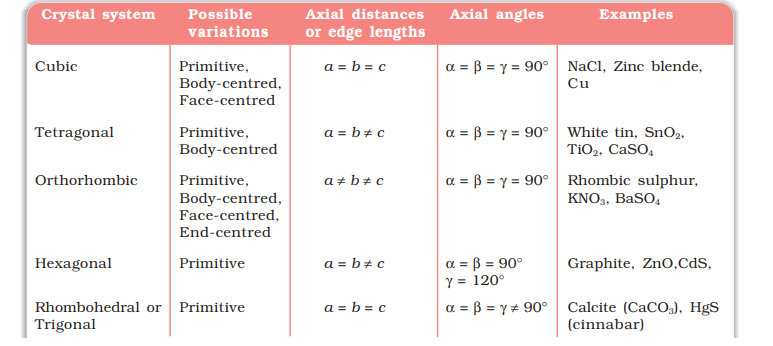
A French mathematician, Bravais, showed that there are only 14 possible three-dimensional lattices. These are called Bravais lattices. Unit cells of these lattices are shown in the following box. The characteristics of their primitive unit cells along with the centred unit cells that they can form have been listed in Table 1.3.
1.5 Number of Atoms in a Unit Cell
We know that any crystal lattice is made up of a very large number of unit cells and every lattice point is occupied by one constituent particle (atom, molecule or ion). Let us now work out what portion of each particle belongs to a particular unit cell.

We shall consider three types of cubic unit cells and for simplicity assume that the constituent particle is an atom.
Primitive cubic unit cell has atoms only at its corner. Each atom at a corner is shared between eight adjacent unit cells as shown in Fig. 1.12, four unit cells in the same layer and four unit cells of the upper (or lower) layer. Therefore, only 1 8 th of an atom (or molecule or ion) actually belongs to a particular unit cell. In Fig. 1.13, a primitive cubic unit cell has been depicted in three different ways. Each small sphere in Fig. 1.13(a) represents only the centre of the particle occupying that position and not its actual size. Such structures are called open structures. The arrangement of particles is easier to follow in open structures. Fig. 1.13 (b) depicts space-filling representation of the unit cell with actual particle size and Fig. 1.13 (c) shows the actual portions of different atoms present in a cubic unit cell.
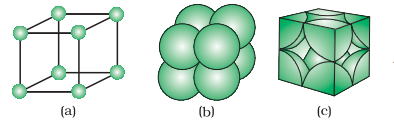
In all, since each cubic unit cell has 8 atoms on its corners, the total number of atoms in one unit cell is 8 × 1/8 = 1 atom.
1.5.2 Body- Centred Cubic Unit Cell
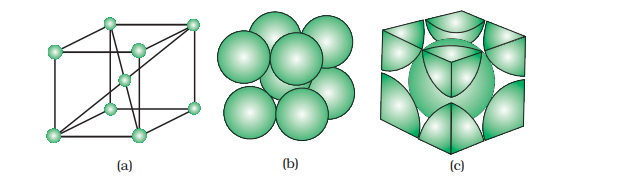
A body-centred cubic (bcc) unit cell has an atom at each of its corners and also one atom at its body centre. Fig. 1.14 depicts (a) open structure (b) space filling model and (c) the unit cell with portions of atoms actually belonging to it. It can be seen that the atom at the body centre wholly belongs to the unit cell in which it is present. Thus in a body-centered cubic (bcc) unit cell:
(i) 8 corners × 1/8 per corner atom = 8 ×1/8 = 1 atom
(ii) 1 body centre atom = 1 × 1 = 1 atom \ Total number of atoms per unit cell = 2 atoms
1.5.3 Face- Centred Cubic Unit Cell
A face-centred cubic (fcc) unit cell contains atoms at all the corners and at the centre of all the faces of the cube. It can be seen in Fig. 1.15 that each atom located at the face-centre is shared between two adjacent unit cells and only 1/2 of each atom belongs to a unit cell. Fig. 1.16 depicts (a) open structure (b) space-filling model and (c) the unit cell with portions of atoms actually belonging to it. Thus, in a face-centred cubic (fcc) unit cell:

(i) 8 corners atoms × 1/8 atom per unit cell = 8 × 1/8 = 1 atom
(ii) 6 face-centred atoms × 1/2 atom per unit cell = 6 × 1/2 = 3 atoms
Total number of atoms per unit cell = 4 atoms
Intext Questions
1.9 Give the significance of a ‘lattice point’.
Answer The significance of a lattice point is that each lattice point represents one constituent particle of a solid which may be an atom, a molecule (group of atom), or an ion.Show Answer
Answer The six parameters that characterise a unit cell are as follows. (i) Its dimensions along the three edges, $a, b$, and $c$ These edges may or may not be equal. (ii) Angles between the edges These are the angle $\propto$ (between edges b and c), (between edges a and c), and (between edges a and b)Show Answer
(i) Hexagonal and monoclinic unit cells
(ii) Face-centred and end-centred unit cells.
Answer (i) Hexagonal unit cell For a hexagonal unit cell, $a=b \neq c$ and $\alpha=\beta=90^{\circ}$ $\gamma=120^{\circ}$ Monoclinic unit cell For a monoclinic cell, $a \neq b \neq c$ and $\alpha=\gamma=90^{\circ}$ $\beta \neq 90^{\circ}$ (ii) Face-centred unit cell In a face-centred unit cell, the constituent particles are present at the corners and one at the centre of each face. End-centred unit cell Anend-centred unit cell contains particles at the corners and one at the centre of any two opposite faces.Show Answer
Show Answer
Answer
(i) An atom located at the corner of a cubic unit cell is shared by eight adjacent unit cells.
Therefore, $\frac{1}{8}$ th portion of the atom is shared by one unit cell.
(ii) An atom located at the body centre of a cubic unit cell is not shared by its neighbouring unit cell. Therefore, the atom belongs only to the unit cell in which it is present i.e., its contribution to the unit cell is 1.
1.6 Close Packed Structures
In solids, the constituent particles are close-packed, leaving the minimum vacant space. Let us consider the constituent particles as identical hard spheres and build up the three-dimensional structure in three steps.
(a) Close Packing in One Dimension There is only one way of arranging spheres in a one-dimensional close packed structure, that is to arrange them in a row and touching each other (Fig. 1.17).
In this arrangement, each sphere is in contact with two of its neighbours. The number of nearest neighbours of a particle is called its coordination number. Thus, in one dimensional close packed arrangement, the coordination number is 2.
(b) Close Packing in Two Dimensions
Two dimensional close packed structure can be generated by stacking (placing) the rows of close packed spheres. This can be done in two different ways.
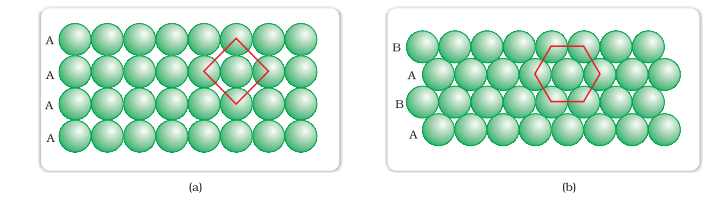
(i) The second row may be placed in contact with the first one such that the spheres of the second row are exactly above those of the first row. The spheres of the two rows are aligned horizontally as well as vertically. If we call the first row as ‘A’ type row, the second row being exactly the same as the first one, is also of ‘A’ type. Similarly, we may place more rows to obtain AAA type of arrangement as shown in Fig. 1.18 (a).
In this arrangement, each sphere is in contact with four of its neighbours. Thus, the two dimensional coordination number is 4. Also, if the centres of these 4 immediate neighbouring spheres are joined, a square is formed. Hence this packing is called square close packing in two dimensions.
(ii) The second row may be placed above the first one in a staggered manner such that its spheres fit in the depressions of the first row. If the arrangement of spheres in the first row is called ‘A’ type, the one in the second row is different and may be called ‘B’ type. When the third row is placed adjacent to the second in staggered manner, its spheres are aligned with those of the first layer. Hence this layer is also of ‘A’ type. The spheres of similarly placed fourth row will be aligned with those of the second row (‘B’ type). Hence this arrangement is of ABAB type. In this arrangement there is less free space and this packing is more efficient than the square close packing. Each sphere is in contact with six of its neighbours and the two dimensional coordination number is 6. The centres of these six spheres are at the corners of a regular hexagon (Fig. 1.18 b) hence this packing is called two dimensional hexagonal closepacking. It can be seen in Figure 1.18 (b) that in this layer there are some voids (empty spaces). These are triangular in shape. The triangular voids are of two different types. In one row, the apex of the triangles are pointing upwards and in the next layer downwards.
(c) Close Packing in Three Dimensions
All real structures are three dimensional structures. They can be obtained by stacking two dimensional layers one above the other. In the last Section, we discussed close packing in two dimensions which can be of two types; square close-packed and hexagonal close-packed. Let us see what types of three dimensional close packing can be obtained from these.

(i) Three-dimensional close packing forms two-dimensional square close-packed layers: While placing the second square close-packed layer above the first we follow the same rule that was followed when one row was placed adjacent to the other. The second layer is placed over the first layer such that the spheres of the upper layer are exactly above those of the first layer. In this arrangement spheres of both the layers are perfectly aligned horizontally as well as vertically as shown in Fig. 1.19. Similarly, we may place more layers one above the other. If the arrangement of spheres in the first layer is called ‘A’ type, all the layers have the same arrangement. Thus this lattice has AAA…. type pattern. The lattice thus generated is the simple cubic lattice, and its unit cell is the primitive cubic unit cell (See Fig. 1.19).
(ii) Three dimensional close packing from two dimensional hexagonal close packed layers: Three dimensional close packed structure can be generated by placing layers one over the other.
(a) Placing second layer over the first layer
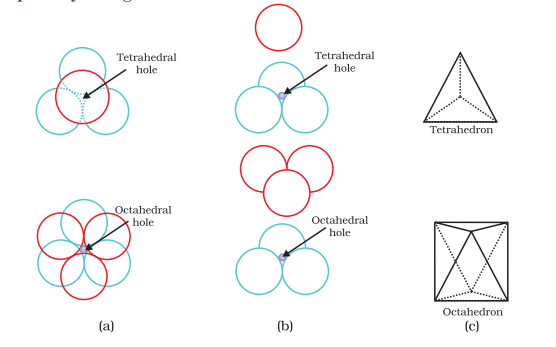
Let us take a two dimensional hexagonal close packed layer ‘A’ and place a similar layer above it such that the spheres of the second layer are placed in the depressions of the first layer. Since the spheres of the two layers are aligned differently, let us call the second layer as B. It can be observed from Fig. 1.20 that all the triangular voids of the first layer are not covered by the spheres of the second layer. This gives rise to different arrangements. Wherever a sphere of the second layer is above the void of the first layer (or vice versa) a tetrahedral void is formed. These voids are called tetrahedral voids because a tetrahedron is formed when the centres of these four spheres are joined. They have been marked as ‘T’ in Fig. 1.20. One such void has been shown separately in Fig. 1.21.

At other places, the triangular voids in the second layer are above the triangular voids in the first layer, and the triangular shapes of these do not overlap. One of them has the apex of the triangle pointing upwards and the other downwards. These voids have been marked as ‘O’ in Fig. 1.20. Such voids are surrounded by six spheres and are called octahedral voids. One such void has been shown separately in Fig. 1.21. The number of these two types of voids depend upon the number of close packed spheres.
Let the number of close packed spheres be N, then: The number of octahedral voids generated = N The number of tetrahedral voids generated = 2N
(b) Placing third layer over the second layer
When third layer is placed over the second, there are two possibilities.
(i) Covering Tetrahedral Voids: Tetrahedral voids of the second layer may be covered by the spheres of the third layer. In this case, the spheres of the third layer are exactly aligned with those of the first layer. Thus, the pattern of spheres is repeated in alternate layers. This pattern is often written as ABAB ……. pattern. This structure is called hexagonal close packed (hcp) structure (Fig. 1.22). This sort of arrangement of atoms is found in many metals like magnesium and zinc.
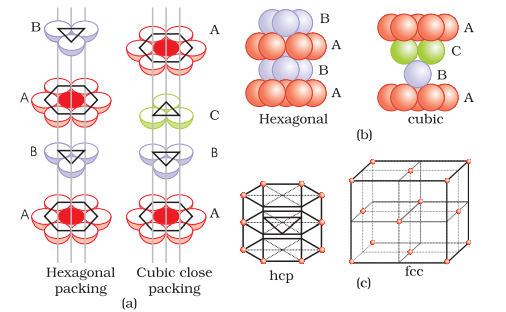
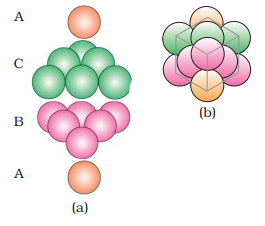
(ii) Covering Octahedral Voids: The third layer may be placed above the second layer in a manner such that its spheres cover the octahedral voids. When placed in this manner, the spheres of the third layer are not aligned with those of either the first or the second layer. This arrangement is called ‘C’ type. Only when fourth layer is placed, its spheres are aligned with those of the first layer as shown in Figs. 1.22 and 1.23. This pattern of layers is often written as ABCABC ……….. This structure is called cubic close packed (ccp) or face-centred cubic (fcc) structure. Metals such as copper and silver crystallise in this structure.
Both these types of close packing are highly efficient and 74% space in the crystal is filled. In either of them, each sphere is in contact with twelve spheres. Thus, the coordination number is 12 in either of these two structures.
1.6.1 Formula of a Compound and Number of Voids Filled
Earlier in the section, we have learnt that when particles are closepacked resulting in either ccp or hcp structure, two types of voids are generated. While the number of octahedral voids present in a lattice is equal to the number of close packed particles, the number of tetrahedral voids generated is twice this number. In ionic solids, the bigger ions (usually anions) form the close packed structure and the smaller ions (usually cations) occupy the voids. If the latter ion is small enough then tetrahedral voids are occupied, if bigger, then octahedral voids. All octahedral or tetrahedral voids are not occupied. In a given compound, the fraction of octahedral or tetrahedral voids that are occupied, depends upon the chemical formula of the compound, as can be seen from the following examples.
Example 1.1
A compound is formed by two elements X and Y. Atoms of the element Y (as anions) make ccp and those of the element X (as cations) occupy all the octahedral voids. What is the formula of the compound?
Solution
The ccp lattice is formed by the element Y. The number of octahedral voids generated would be equal to the number of atoms of Y present in it. Since all the octahedral voids are occupied by the atoms of X, their number would also be equal to that of the element Y. Thus, the atoms of elements X and Y are present in equal numbers or 1:1 ratio. Therefore, the formula of the compound is XY.
Example 1.2
Atoms of element B form hcp lattice and those of the element A occupy 2/3rd of tetrahedral voids. What is the formula of the compound formed by the elements $\mathrm{A}$ and $\mathrm{B}$ ?
Solution
The number of tetrahedral voids formed is equal to twice the number of atoms of element $\mathrm{B}$ and only 2/3rd of these are occupied by the atoms of element $\mathrm{A}$. Hence the ratio of the number of atoms of $\mathrm{A}$ and $\mathrm{B}$ is 2 $\times(2 / 3): 1$ or $4: 3$ and the formula of the compound is $\mathrm{A_4} \mathrm{~B_3}$.
Locating Tetrahedral and Octahedral Voids
We know that close packed structures have both tetrahedral and octahedral voids. Let us take ccp (or fcc) structure and locate these voids in it.
(a) Locating Tetrahedral Voids
Let us consider a unit cell of ccp or fcc lattice [Fig. 1(a)]. The unit cell is divided into eight small cubes.
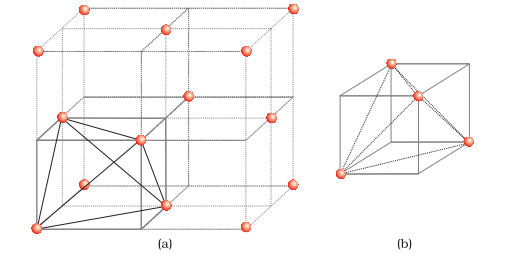
Each small cube has atoms at alternate corners [Fig. 1(a)]. In all, each small cube has 4 atoms. When joined to each other, they make a regular tetrahedron. Thus, there is one tetrahedral void in each small cube and eight tetrahedral voids in total. Each of the eight small cubes have one void in one unit cell of ccp structure. We know that ccp structure has 4 atoms per unit cell. Thus, the number of tetrahedral voids is twice the number of atoms.
(b) Locating Octahedral Voids
Let us again consider a unit cell of ccp or fcc lattice [Fig. 2(a)]. The body centre of the cube, C is not occupied but it is surrounded by six atoms on face centres. If these face centres are joined, an octahedron is generated. Thus, this unit cell has one octahedral void at the body centre of the cube.
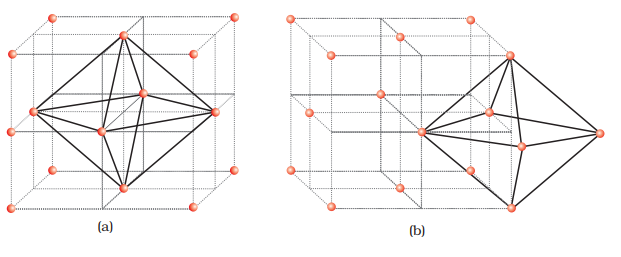
Besides the body centre, there is one octahedral void at the centre of each of the 12 edges [Fig. 2(b)]. It is surrounded by six atoms, four belonging to the same unit cell (2 on the corners and 2 on face centre) and two belonging to two adjacent unit cells. Since each edge of the cube is shared between four adjacent unit cells, so is the octahedral void located on it. Only 1/4 th of each void belongs to a particular unit cell.
Thus in cubic close packed structure: Octahedral void at the body-centre of the cube = 1 12 octahedral voids located at each edge and shared between four unit cells = 12 × 1/4 = 3
Total number of octahedral voids = 4 We know that in ccp structure, each unit cell has 4 atoms. Thus, the number of octahedral voids is equal to this number.
1.7 Packing Efficiency
In whatever way the constituent particles (atoms, molecules or ions) are packed, there is always some free space in the form of voids. Packing efficiency is the percentage of total space filled by the particles. Let us calculate the packing efficiency in different types of structures.
1.7.1 Packing Efficiency in hcp and ccp Structures
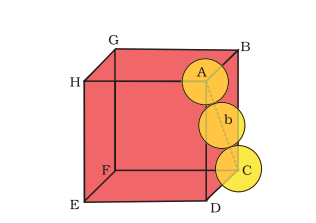
Both types of close packing (hcp and ccp) are equally efficient. Let us calculate the efficiency of packing in ccp structure. In Fig. 1.24 let the unit cell edge length be ‘a’ and face diagonal AC = b.
In $\triangle \mathrm{ABC}$
$\mathrm{AC}^{2}=\mathrm{b}^{2}=\mathrm{BC}^{2}+\mathrm{AB}^{2}$
$=a^{2}+a^{2}=2 a^{2}$ or
$b=\sqrt{2} a$
If $r$ is the radius of the sphere, we find
$b=4 \mathrm{r}=\sqrt{2} a$
or $a=\frac{4 \mathrm{r}}{\sqrt{2}}=2 \sqrt{2} \mathrm{r}$
(we can also write, $\mathrm{r}=\frac{\mathrm{a}}{2 \sqrt{2}}$ )
We know, that each unit cell in ccp structure, has effectively 4 spheres. Total volume of four spheres is equal to $4 \times(4 / 3) \pi r^{3}$ and volume of the cube is $\mathrm{a}^{3}$ or $(2 \sqrt{2} \mathrm{r})^{3}$.
Therefore,
Packing efficiency $=\frac{\text { Volume occupied by four spheres in the unit cell } \times 100}{\text { Total volume of the unit cell }} $%
$$ \begin{aligned} & =\frac{4 \times(4 / 3) \pi r^{3} \times 100}{(2 \sqrt{2} r)^{3}} \% \end{aligned} $$ $$ \begin{aligned} & =\frac{(16 / 3) \pi r^{3} \times 100}{16 \sqrt{2} r^{3}} \% =74 \% \end{aligned} $$
1.7.2 Efficiency of Packing in Body- Centred Cubic Structures
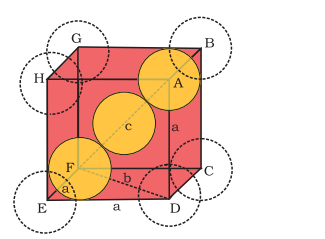
From Fig. 1.25, it is clear that the atom at the centre will be in touch with the other two atoms diagonally arranged.
In $\triangle \mathrm{EFD}$,
$\mathrm{b}^{2}=\mathrm{a}^{2}+\mathrm{a}^{2}=2 \mathrm{a}^{2}$
$\mathrm{b}=\sqrt{2} \mathrm{a}$
Now in $\triangle$ AFD
$$ \begin{aligned} & c^{2}=a^{2}+b^{2}=a^{2}+2 a^{2}=3 a^{2} \\ & c=\sqrt{3} a \end{aligned} $$
The length of the body diagonal $c$ is equal to $4 r$, where $r$ is the radius of the sphere (atom), as all the three spheres along the diagonal touch each other.
Therefore, $$\quad \sqrt{3} \mathrm{a}=4 \mathrm{r}$$ $$ \mathrm{a}=\frac{4 \mathrm{r}}{\sqrt{3}} $$
Also we can write, $r=\frac{\sqrt{3}}{4} \mathrm{a}$
In this type of structure, total number of atoms is 2 and their volume is $2 \times\left(\frac{4}{3}\right) \pi \mathrm{r}^{3}$.
Volume of the cube, $\mathrm{a}^{3}$ will be equal to $\left(\frac{4}{\sqrt{3}} \mathrm{r}\right)^{3}$ or $\mathrm{a}^{3}=\left(\frac{4}{\sqrt{3}} \mathrm{r}\right)^{3}$.
Therefore,
Packing efficiency $=\frac{\text { Volume occupied by two spheres in the unit cell } \times 100}{\text { Total volume of the unit cell }} \%$
$$ \begin{aligned} & =\frac{2 \times(4 / 3) \pi r^{3} \times 100}{[(4 / \sqrt{3}) r]^{3}} \% \\ & =\frac{(8 / 3) \pi r^{3} \times 100}{64 /(3 \sqrt{3}) r^{3}}=68 \% \end{aligned} $$
1.7.3 Packing Efficiency in Simple Cubic Lattice
In a simple cubic lattice the atoms are located only on the corners of the cube. The particles touch each other along the edge (Fig. 1.26).
Thus, the edge length or side of the cube ‘a’, and the radius of each particle, r are related as
$$ \mathrm{a}=2 \mathrm{r} $$
The volume of the cubic unit cell $=a^{3}=(2 r)^{3}=8 r^{3}$
Since a simple cubic unit cell contains only 1 atom
The volume of the occupied space $=\frac{4}{3} \pi \mathrm{r}^{3}$
Packing efficiency
$\therefore$ Packing efficiency $$ \begin{gathered} =\frac{\text { Volume of one atom }}{\text { Volume of cubic unit cell }} \times 100 \% \\ =\frac{\frac{4}{3} \pi \mathrm{r}^3}{8 \mathrm{r}^3} \times 100=\frac{\pi}{6} \times 100 \\ =52.36 \%=52.4 \% \end{gathered} $$
Thus, we may conclude that $c c p$ and hcp structures have maximum packing efficiency.
1.8 Calculations Involving Unit Cell Dimensions
From the unit cell dimensions, it is possible to calculate the volume of the unit cell. Knowing the density of the metal, we can calculate the mass of the atoms in the unit cell. The determination of the mass of a single atom gives an accurate method of determination of Avogadro constant. Suppose, edge length of a unit cell of a cubic crystal determined by X-ray diffraction is a, d the density of the solid substance and M the molar mass. In case of cubic crystal:
Volume of a unit cell $=a^{3}$
Mass of the unit cell
$=$ number of atoms in unit cell $\times$ mass of each atom $=Z \times m$
(Here $z$ is the number of atoms present in one unit cell and $m$ is the mass of a single atom)
Mass of an atom present in the unit cell:
$$ m=\frac{M}{N_{A}}(M \text { is molar mass }) $$
Therefore, density of the unit cell
$$ \begin{aligned} & =\frac{\text { mass of unit cell }}{\text { volume of unit cell }} \\ & =\frac{z \cdot m}{a^{3}}=\frac{z \cdot M}{a^{3} \cdot N_{A}} \text { or } d=\frac{z M}{a^{3} N_{A}} \end{aligned} $$
Remember, the density of the unit cell is the same as the density of the substance. The density of the solid can always be determined by other methods. Out of the five parameters $\left(d, z M, a\right.$ and $\left.N_{A}\right)$, if any four are known, we can determine the fifth.
Example 1.3
An element has a body-centred cubic (bcc) structure with a cell edge of $288 \mathrm{pm}$. The density of the element is $7.2 \mathrm{~g} / \mathrm{cm}^{3}$. How many atoms are present in $208 \mathrm{~g}$ of the element?
Solution
Volume of the unit cell $=(288 \mathrm{pm})^{3}$
$$ \begin{aligned} & =\left(288 \times 10^{-12} \mathrm{~m}\right)^{3}=\left(288 \times 10^{-10} \mathrm{~cm}\right)^{3} \\ & =2.39 \times 10^{-23} \mathrm{~cm}^{3} \end{aligned} $$
Volume of $208 \mathrm{~g}$ of the element
$$ =\frac{\text { mass }}{\text { density }}=\frac{208 \mathrm{~g}}{7.2 \mathrm{~g} \mathrm{~cm}^{-3}}=28.88 \mathrm{~cm}^{3} $$
Number of unit cells in this volume
$$ =\frac{28.88 \mathrm{~cm}^{3}}{2.39 \times 10^{-23} \mathrm{~cm}^{3} / \text { unit cell }}=12.08 \times 10^{23} \text { unit cells } $$
Since each $b c c$ cubic unit cell contains 2 atoms, therefore, the total number of atoms in $208 \mathrm{~g}=2$ (atoms/unit cell) $\times 12.08 \times 10^{23}$ unit cells
$$ =24.16 \times 10^{23} \text { atoms } $$
Example 1.4
X-ray diffraction studies show that copper crystallises in an fcc unit cell with cell edge of 3.608×10-8 cm. In a separate experiment, copper is determined to have a density of 8.92 g/cm3, calculate the atomic mass of copper.
In case of fcc lattice, number of atoms per unit cell, z = 4 atoms Therefore, $M=\frac{\mathrm{dN}_{\mathrm{A}} \mathrm{a}^{3}}{\mathrm{Z}}$
$=63.1 \mathrm{~g} / \mathrm{mol}$
Atomic mass of copper $=63.1 \mathrm{u}$
Example 1.5
Silver forms ccp lattice and X-ray studies of its crystals show that the edge length of its unit cell is 408.6 pm. Calculate the density of silver (Atomic mass = 107.9 u).
Since the lattice is ccp, the number of silver atoms per unit cell = z = 4 Molar mass of silver $=107.9 \mathrm{~g} \mathrm{~mol}^{-1}=107.9 \times 10^{-3} \mathrm{~kg} \mathrm{~mol}^{-1}$
Edge length of unit cell $=\mathrm{a}=408.6 \mathrm{pm}=408.6 \times 10^{-12} \mathrm{~m}$
Density, $d=\frac{z \cdot \mathrm{M}}{\mathrm{a}^{3} \cdot \mathrm{N}_{\mathrm{A}}}$
$$ \begin{aligned} & =\frac{4 \times\left(107.9 \times 10^{-3} \mathrm{~kg} \mathrm{~mol}^{-1}\right)}{\left(408.6 \times 10^{-12} \mathrm{~m}\right)^{3}\left(6.022 \times 10^{23} \mathrm{~mol}^{-1}\right)}=10.5 \times 10^{3} \mathrm{~kg} \mathrm{~m}^{-3} \\ & =10.5 \mathrm{~g} \mathrm{~cm}^{-3} \end{aligned} $$
Intext Questions
1.13 What is the two dimensional coordination number of a molecule in square close-packed layer?
Answer In square close-packed layer, a molecule is in contact with four of its neighbours. Therefore, the two-dimensional coordination number of a molecule in square close-packed layer is 4.Show Answer
Answer Number of close-packed particles $=0.5 \times 6.022 \times 10^{23}=3.011 \times 10^{23}$ Therefore, number of octahedral voids $=3.011 \times 10^{23}$ And, number of tetrahedral voids $=2 \times 3.011 \times 10^{23}=6.022 \times 10^{23}$ Therefore, total number of voids $=3.011 \times 10^{23}+6.022 \times 10^{23}=9.033 \times 10^{23}$Show Answer
Answer The ccplattice is formed by the atoms of the element $\mathrm{N}$. Here, the number of tetrahedral voids generated is equal to twice the number of atoms of the element $\mathrm{N}$. According to the question, the atoms of element M occupy $\frac{1}{3}^{\text {rd }}$ of the tetrahedral voids. Therefore, the number of atoms of $M$ is equal to $2 \times \frac{1}{3}=\frac{2}{3}$ rd of the number of atoms of $\mathrm{N}$. Therefore, ratio of the number of atoms of $\mathrm{M}$ to that of $\mathrm{N}$ is $\mathrm{M}: \mathrm{N}=\frac{2}{3}: 1$
$=2: 3$ Thus, the formula of the compound is $\mathrm{M_2} \mathrm{~N_3}$.Show Answer
Answer Hexagonal close-packed lattice has the highest packing efficiency of $74 \%$. The packing efficiencies of simple cubic and body-centred cubic lattices are $52.4 \%$ and $68 \%$ respectively.Show Answer
Show Answer
Answer
It is given that density of the element, $d=2.7 \times 10^{3} \mathrm{~kg} \mathrm{~m}^{-3}$
Molar mass, $\mathrm{M}=2.7 \times 10^{-2} \mathrm{~kg} \mathrm{~mol}^{-1}$
Edge length, $a=405 \mathrm{pm}=405 \times 10^{-12} \mathrm{~m}$
$=4.05 \times 10^{-10} \mathrm{~m}$
It is known that, Avogadro’s number, $N_{A}=6.022 \times 1023 \mathrm{~mol}^{-1}$
Applying the relation,
$$ \begin{aligned} d & =\frac{z, M}{a^{3} \cdot \mathrm{N_A}} \\ \end{aligned} $$
$$ \begin{aligned} z & =\frac{d \cdot a^{3} \mathrm{~N_A}}{M} \\ & =\frac{2.7 \times 10^{3} \mathrm{~kg} \mathrm{~m}^{-3} \times\left(4.05 \times 10^{-10} \mathrm{~m}\right)^{3} \times 6.022 \times 10^{23} \mathrm{~mol}^{-1}}{2.7 \times 10^{-2} \mathrm{~kg} \mathrm{~mol}^{-1}} \\ & =4.004 \\ & =4 \end{aligned} $$
This implies that four atoms of the element are present per unit cell. Hence, the unit cell is face-centred cubic (fcc) or cubic close-packed ( $c c p$ ).
1.9 Imperfections in Solids
Although crystalline solids have short range as well as long range order in the arrangement of their constituent particles, yet crystals are not perfect. Usually a solid consists of an aggregate of large number of small crystals. These small crystals have defects in them. This happens when crystallisation process occurs at fast or moderate rate. Single crystals are formed when the process of crystallisation occurs at extremely slow rate. Even these crystals are not free of defects. The defects are basically irregularities in the arrangement of constituent particles. Broadly speaking, the defects are of two types, namely, point defects and line defects. Point defects are the irregularities or deviations from ideal arrangement around a point or an atom in a crystalline substance, whereas the line defects are the irregularities or deviations from ideal arrangement in entire rows of lattice points. These irregularities are called crystal defects. We shall confine our discussion to point defects only.
1.9.1 Types of Point Defects
Point defects can be classified into three types : (i) stoichiometric defects (ii) impurity defects and (iii) non-stoichiometric defects.
(a) Stoichiometric Defects These are the point defects that do not disturb the stoichiometry of the solid. They are also called intrinsic or thermodynamic defects. Basically these are of two types, vacancy defects and interstitial defects.
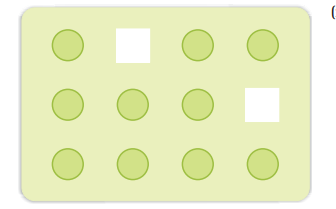
(i) Vacancy Defect: When some of the lattice sites are vacant, the crystal is said to have vacancy defect (Fig. 1.27). This results in decrease in density of the substance. This defect can also develop when a substance is heated.
(ii) Interstitial Defect: When some constituent particles (atoms or molecules) occupy an interstitial site, the crystal is said to have interstitial defect (Fig. 1.28). This defect increases the density of the substance.
Vacancy and interstitial defects as explained above can be shown by non-ionic solids. Ionic solids must always maintain electrical neutrality. Rather than simple vacancy or interstitial defects, they show these defects as Frenkel and Schottky defects.
(iii) Frenkel Defect: This defect is shown by ionic solids. The smaller ion (usually cation) is dislocated from its normal site to an interstitial site (Fig. 1.29). It creates a vacancy defect at its original site and an interstitial defect at its new location.
Frenkel defect is also called dislocation defect. It does not change the density of the solid. Frenkel defect is shown by ionic substance in which there is a large difference in the size of ions, for example, $\mathrm{ZnS}, \mathrm{AgCl}, \mathrm{AgBr}$ and $\mathrm{AgI}$ due to small size of $\mathrm{Zn}^{2+}$ and $\mathrm{Ag}^{+}$ions.

(iv) Schottky Defect: It is basically a vacancy defect in ionic solids. In order to maintain electrical neutrality, the number of missing cations and anions are equal (Fig. 1.30).

Like simple vacancy defect, Schottky defect also decreases the density of the substance. Number of such defects in ionic solids is quite significant. For example, in $\mathrm{NaCl}$ there are approximately $10^{6}$ Schottky pairs per $\mathrm{cm}^{3}$ at room temperature. In $1 \mathrm{~cm}^{3}$ there are about $10^{22}$ ions. Thus, there is one Schottky defect per $10^{16}$ ions. Schottky defect is shown by ionic substances in which the cation and anion are of almost similar sizes. For example, $\mathrm{NaCl}, \mathrm{KCl}, \mathrm{CsCl}$ and $\mathrm{AgBr}$. It may be noted that AgBr shows both, Frenkel as well as Schottky defects.
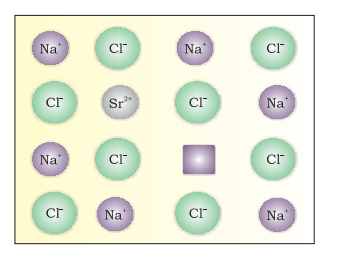
(b) Impurity Defects : If molten $\mathrm{NaCl}$ containing a little amount of $\mathrm{SrCl_2}$ is crystallised, some of the sites of $\mathrm{Na}^{+}$ions are occupied by $\mathrm{Sr}^{2+}$. Each $\mathrm{Sr}^{2+}$ replaces two $\mathrm{Na}^{+}$ions. It occupies the site of one ion and the other site remains vacant. The cationic vacancies thus produced are equal in number to that of $\mathrm{Sr}^{2+}$ ions. Another similar example is the solid solution of $\mathrm{CdCl}_{2}$ and $\mathrm{AgCl}$.
(c) Non-Stoichiometric Defects : The defects discussed so far do not disturb the stoichiometry of the crystalline substance. However, a large number of nonstoichiometric inorganic solids are known which contain the constituent elements in non-stoichiometric ratio due to defects in their crystal structures. These defects are of two types: (i) metal excess defect and (ii) metal deficiency defect.
(i) Metal Excess Defect : A Metal excess defect due to anionic vacancies: Alkali halides like NaCl and KCl show this type of defect. When crystals of NaCl are heated in an atmosphere of sodium vapour, the sodium atoms are deposited on the surface of the crystal. The Cl– ions diffuse to the surface of the crystal and combine with Na atoms to give NaCl. This happens by loss of electron by sodium atoms to form Na+ ions. The released electrons diffuse into the crystal and occupy anionic sites (Fig. 1.32). As a result the crystal now has an excess of sodium. The anionic sites occupied by unpaired electrons are called F-centres (from the German word Farbenzenter for colour centre). They impart yellow colour to the crystals of NaCl. The colour results by excitation of these electrons when they absorb energy from the visible light falling on the crystals. Similarly, excess of lithium makes LiCl crystals pink and excess of potassium makes KCl crystals violet (or lilac).
à Metal excess defect due to the presence of extra cations at interstitial sites: Zinc oxide is white in colour at room temperature. On heating it loses oxygen and turns yellow. $$ \mathrm{ZnO} \xrightarrow{\text { heating }} \mathrm{Zn}^{2+}+\frac{1}{2} \mathrm{O_2}+2 \mathrm{e}^{-} $$
Now there is excess of zinc in the crystal and its formula becomes $\mathrm{Zn_1+\mathrm{x}} \mathrm{O}$. The excess $\mathrm{Zn}^{2+}$ ions move to interstitial sites and the electrons to neighbouring interstitial sites.
(ii) Metal Deficiency Defect There are many solids which are difficult to prepare in the stoichiometric composition and contain less amount of the metal as compared to the stoichiometric proportion. A typical example of this type is $\mathrm{FeO}$ which is mostly found with a composition of $\mathrm{Fe_0.95} \mathrm{O}$. It may actually range from $\mathrm{Fe_0.93} \mathrm{O}$ to $\mathrm{Fe_0.96} \mathrm{O}$. In crystals of $\mathrm{FeO}$ some $\mathrm{Fe}^{2+}$ cations are missing and the loss of positive charge is made up by the presence of required number of $\mathrm{Fe}^{3+}$ ions.
1.10 Electrical Properties
Solids exhibit an amazing range of electrical conductivities, extending over 27 orders of magnitude ranging from $10^{-20}$ to $10^{7} \mathrm{ohm}^{-1} \mathrm{~m}^{-1}$. Solids can be classified into three types on the basis of their conductivities.
(i) Conductors: The solids with conductivities ranging between $10^{4}$ to $10^{7} \mathrm{ohm}^{-1} \mathrm{~m}^{-1}$ are called conductors. Metals have conductivities in the order of $10^{7} \mathrm{ohm}^{-1} \mathrm{~m}^{-1}$ are good conductors.
(ii) Insulators : These are the solids with very low conductivities ranging between $10^{-20}$ to $10^{-10} \mathrm{ohm}^{-1} \mathrm{~m}^{-1}$.
(iii) Semiconductors: These are the solids with conductivities in the intermediate range from $10^{-6}$ to $10^{4} \mathrm{ohm}^{-1} \mathrm{~m}^{-1}$.
1.10.1 Conduction of Electricity in Metals
A conductor may conduct electricity through movement of electrons or ions. Metallic conductors belong to the former category and electrolytes to the latter.
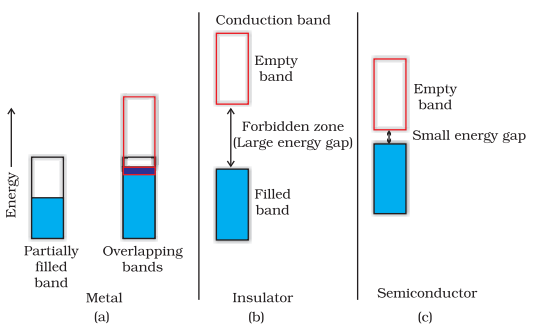
Metals conduct electricity in solid as well as molten state. The conductivity of metals depend upon the number of valence electrons available per atom. The atomic orbitals of metal atoms form molecular orbitals which are so close in energy to each other as to form a band. If this band is partially filled or it overlaps with a higher energy unoccupied conduction band, then electrons can flow easily under an applied electric field and the metal shows conductivity (Fig. 1.33 a).
If the gap between filled valence band and the next higher unoccupied band (conduction band) is large, electrons cannot jump to it and such a substance has very small conductivity and it behaves as an insulator (Fig. 1.33 b).
1.10.2 Conduction of Electricity in Semiconductors
In case of semiconductors, the gap between the valence band and conduction band is small (Fig. 1.33 c). Therefore, some electrons may jump to conduction band and show some conductivity. Electrical conductivity of semiconductors increases with rise in temperature, since more electrons can jump to the conduction band. Substances like silicon and germanium show this type of behaviour and are called intrinsic semiconductors.
The conductivity of these intrinsic semiconductors is too low to be of practical use. Their conductivity is increased by adding an appropriate amount of suitable impurity. This process is called doping. Doping can be done with an impurity which is electron rich or electron deficient as compared to the intrinsic semiconductor silicon or germanium. Such impurities introduce electronic defects in them.
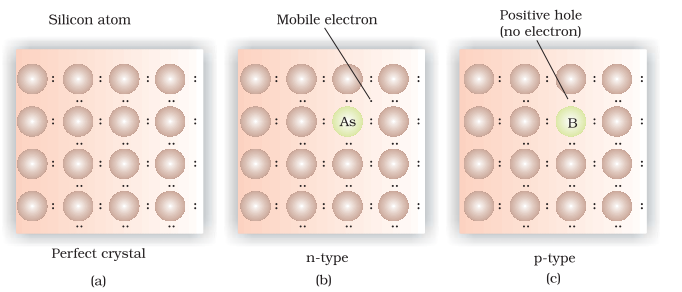
(a) Electron - rich impurities Silicon and germanium belong to group 14 of the periodic table and have four valence electrons each. In their crystals each atom forms four covalent bonds with its neighbours (Fig. 1.34 a). When doped with a group 15 element like P or As, which contains five valence electrons, they occupy some of the lattice sites in silicon or germanium crystal (Fig. 1.34 b). Four out of five electrons are used in the formation of four covalent bonds with the four neighbouring silicon atoms. The fifth electron is extra and becomes delocalised. These delocalised electrons increase the conductivity of doped silicon (or germanium). Here the increase in conductivity is due to the negatively charged electron, hence silicon doped with electron-rich impurity is called n-type semiconductor.
(b) Electron - deficit impurities Silicon or germanium can also be doped with a group 13 element like B, Al or Ga which contains only three valence electrons. The place where the fourth valence electron is missing is called electron hole or electron vacancy (Fig. 1.34 c). An electron from a neighbouring atom can come and fill the electron hole, but in doing so it would leave an electron hole at its original position. If it happens, it would appear as if the electron hole has moved in the direction opposite to that of the electron that filled it. Under the influence of electric field, electrons would move towards the positively charged plate through electronic holes, but it would appear as if electron holes are positively charged and are moving towards negatively charged plate. This type of semi conductors are called p-type semiconductors.
Applications of n-type and p-type semiconductors
Various combinations of n-type and p-type semiconductors are used for making electronic components. Diode is a combination of n-type and p-type semiconductors and is used as a rectifier. Transistors are made by sandwiching a layer of one type of semiconductor between two layers of the other type of semiconductor. npn and pnp type of transistors are used to detect or amplify radio or audio signals. The solar cell is an efficient photo-diode used for conversion of light energy into electrical energy.
Germanium and silicon are group 14 elements and therefore, have a characteristic valence of four and form four bonds as in diamond. A large variety of solid state materials have been prepared by combination of groups 13 and 15 or 12 and 16 to simulate average valence of four as in Ge or Si. Typical compounds of groups $13-15$ are InSb, AlP and GaAs. Gallium arsenide (GaAs) semiconductors have very fast response and have revolutionised the design of semiconductor devices. ZnS, CdS, CdSe and HgTe are examples of groups $12-16$ compounds. In these compounds, the bonds are not perfectly covalent and the ionic character depends on the electronegativities of the two elements.
It is interesting to learn that transition metal oxides show marked differences in electrical properties. TiO, $\mathrm{CrO_2}$ and $\mathrm{ReO_3}$ behave like metals. Rhenium oxide, $\mathrm{ReO_3}$ is like metallic copper in its conductivity and appearance. Certain other oxides like VO, $\mathrm{VO_2}, \mathrm{VO_3}$ and $\mathrm{TiO_3}$ show metallic or insulating properties depending on temperature.
1.11 Magnetic Properties
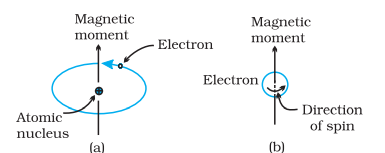
Every substance has some magnetic properties associated with it. The origin of these properties lies in the electrons. Each electron in an atom behaves like a tiny magnet. Its magnetic moment originates from two types of motions (i) its orbital motion around the nucleus and (ii) its spin around its own axis (Fig. 1.35). Electron being a charged particle and undergoing these motions can be considered as a small loop of current which possesses a magnetic moment. Thus, each electron has a permanent spin and an orbital magnetic moment associated with it. Magnitude of this magnetic moment is very small and is measured in the unit called Bohr magneton, μB. It is equal to 9.27 × 10–24A m2.
On the basis of their magnetic properties, substances can be classified into five categories: (i) paramagnetic (ii) diamagnetic (iii) ferromagnetic (iv) antiferromagnetic and (v) ferrimagnetic.
(i) Paramagnetism: Paramagnetic substances are weakly attracted by a magnetic field. They are magnetised in a magnetic field in the same direction. They lose their magnetism in the absence of magnetic field. Paramagnetism is due to presence of one or more unpaired electrons which are attracted by the magnetic field. $\mathrm{O_2}, \mathrm{Cu}^{2+}, \mathrm{Fe}^{3+}, \mathrm{Cr}^{3+}$ are some examples of such substances.
(ii) Diamagnetism: Diamagnetic substances are weakly repelled by a magnetic field. $\mathrm{H_2} \mathrm{O}, \mathrm{NaCl}$ and $\mathrm{C_6} \mathrm{H_6}$ are some examples of such substances. They are weakly magnetised in a magnetic field in opposite direction. Diamagnetism is shown by those substances in which all the electrons are paired and there are no unpaired electrons. Pairing of electrons cancels their magnetic moments and they lose their magnetic character.
(iii) Ferromagnetism: A few substances like iron, cobalt, nickel, gadolinium and CrO2 are attracted very strongly by a magnetic field. Such substances are called ferromagnetic substances. Besides strong attractions, these substances can be permanently magnetised. In solid state, the metal ions of ferromagnetic substances are grouped together into small regions called domains. Thus, each domain acts as a tiny magnet. In an unmagnetised piece of a ferromagnetic substance the domains are randomly oriented and their magnetic moments get cancelled. When the substance is placed in a magnetic field all the domains get oriented in the direction of the magnetic field (Fig. 1.36 a) and a strong magnetic effect is produced. This ordering of domains persist even when the magnetic field is removed and the ferromagnetic substance becomes a permanent magnet.
(iv) Antiferromagnetism: Substances like MnO showing antiferromagnetism have domain structure similar to ferromagnetic substance, but their domains are oppositely oriented and cancel out each other’s magnetic moment (Fig. 1.36 b).
(v) Ferrimagnetism: Ferrimagnetism is observed when the magnetic moments of the domains in the substance are aligned in parallel and anti-parallel directions in unequal numbers (Fig. $1.32 \mathrm{c}$ ). They are weakly attracted by magnetic field as compared to ferromagnetic substances. $\mathrm{Fe_3} \mathrm{O_4}$ (magnetite) and ferrites like $\mathrm{MgFe_2} \mathrm{O_4}$ and $\mathrm{ZnFe_2} \mathrm{O_4}$ are examples of such substances. These substances also lose ferrimagnetism on heating and become paramagnetic.
Intext Questions
1.18 What type of defect can arise when a solid is heated? Which physical property is affected by it and in what way?
Answer When a solid is heated, vacancy defect can arise. A solid crystal is said to have vacancy defect when some of the lattice sites are vacant. Vacancy defect leads to a decrease in the density of the solid.Show Answer
Answer (i) ZnS shows Frenkel defect. (ii) AgBr shows Frenkel defect as well as Schottky defect.Show Answer
Answer When a cation of higher valence is added to an ionic solid as an impurity to it, the cation of higher valence replaces more than one cation of lower valence so as to keep the crystal electrically neutral. As a result, some sites become vacant. For example, when $\mathrm{Sr}^{2+}$ is added to $\mathrm{NaCl}$, each $\mathrm{Sr}^{2+}$ ion replaces two $\mathrm{Na}^{+}$ions. However, one $\mathrm{Sr}^{2+}$ ion occupies the site of one $\mathrm{Na}^{+}$ion and the other site remains vacant. Hence, vacancies are introduced.Show Answer
Answer The colour develops because of the presence of electrons in the anionic sites. These electrons absorb energy from the visible part of radiation and get excited. For example, when crystals of $\mathrm{NaCl}$ are heated in an atmosphere of sodium vapours, the sodium atoms get deposited on the surface of the crystal and the chloride ions from the crystal diffuse to the surface to form $\mathrm{NaCl}$ with the deposited $\mathrm{Na}$ atoms. During this process, the $\mathrm{Na}$ atoms on the surface lose electrons to form $\mathrm{Na}^{+}$ions and the
released electrons diffuse into the crystal to occupy the vacant anionic sites. These electrons get excited by absorbing energy from the visible light and impart yellow colour to the crystals.Show Answer
Answer Ann-type semiconductor conducts because of the presence of extra electrons. Therefore, a group 14 element can be converted to $n$-type semiconductor by doping it with a group 15 element.Show Answer
Show Answer
Answer
Ferromagnetic substances would make better permanent magnets.
In solid state, the metal ions of ferromagnetic substances are grouped together into small regions. These regions are called domains and each domain acts as a tiny magnet. In an unmagnetised piece of a ferromagnetic substance, the domains are randomly oriented. As a result, the magnetic moments of the domains get cancelled. However, when the substance is placed in a magnetic field, all the domains get oriented in the direction of the magnetic field and a strong magnetic effect is produced.
The ordering of the domains persists even after the removal of the magnetic field. Thus, the ferromagnetic substance becomes a permanent magnet.
Summary
Solids have definite mass, volume and shape. This is due to the fixed position of their constituent particles, short distances and strong interactions between them. In amorphous solids, the arrangement of constituent particles has only short range order and consequently they behave like super cooled liquids, do not have sharp melting points and are isotropic in nature. In crystalline solids there is long range order in the arrangement of their constituent particles. They have sharp melting points, are anisotropic in nature and their particles have characteristic shapes. Properties of crystalline solids depend upon the nature of interactions between their constituent particles. On this basis, they can be divided into four categories, namely: molecular, ionic, metallic and covalent solids. They differ widely in their properties.
The constituent particles in crystalline solids are arranged in a regular pattern which extends throughout the crystal. This arrangement is often depicted in the form of a three dimensional array of points which is called crystal lattice. Each lattice point gives the location of one particle in space. In all, fourteen different types of lattices are possible which are called Bravais lattices. Each lattice can be generated by repeating its small characteristic portion called unit cell. A unit cell is characterised by its edge lengths and three angles between these edges. Unit cells can be either primitive which have particles only at their corner positions or centred. The centred unit cells have additional particles at their body centre (bodycentred), at the centre of each face (face-centred) or at the centre of two opposite faces (end-centred). There are seven types of primitive unit cells. Taking centred unit cells also into account, there are fourteen types of unit cells in all, which result in fourteen Bravais lattices.
Close-packing of particles result in two highly efficient lattices, hexagonal close-packed (hcp) and cubic close-packed (ccp). The latter is also called facecentred cubic (fcc) lattice. In both of these packings 74% space is filled. The remaining space is present in the form of two types of voids-octahedral voids and tetrahedral voids. Other types of packing are not close-packings and have less efficient packing of particles. While in body-centred cubic lattice (bcc) 68% space is filled, in simple cubic lattice only 52.4 % space is filled.
Solids are not perfect in structure. There are different types of imperfections or defects in them. Point defects and line defects are common types of defects. Point defects are of three types - stoichiometric defects, impurity defects and non-stoichiometric defects. Vacancy defects and interstitial defects are the two basic types of stoichiometric point defects. In ionic solids, these defects are present as Frenkel and Schottky defects. Impurity defects are caused by the presence of an impurity in the crystal. In ionic solids, when the ionic impurity has a different valence than the main compound, some vacancies are created. Nonstoichiometric defects are of metal excess type and metal deficient type. Sometimes calculated amounts of impurities are introduced by doping in semiconductors that change their electrical properties. Such materials are widely used in electronics industry. Solids show many types of magnetic properties like paramagnetism, diamagnetism, ferromagnetism, antiferromagnetism and ferrimagnetism. These properties are used in audio, video and other recording devices. All these properties can be correlated with their electronic configurations or structures.
Exercises
1.1 Define the term ‘amorphous’. Give a few examples of amorphous solids.
Answer Amorphous solids are the solids whose constituent particles are of irregular shapes and have short range order. These solids are isotropic in nature and melt over a range of temperature. Therefore, amorphous solids are sometimes called pseudo solids or super cooled liquids. They do not have definite heat of fusion. When cut with a sharp-edged tool, they cut into two pieces with irregular surfaces. Examples of amorphous solids include glass, rubber, and plastic.Show Answer
Answer The arrangement of the constituent particles makes glass different from quartz. In glass, the constituent particles have short range order, but in quartz, the constituent particles have both long range and short range orders. Quartz can be converted into glass by heating and then cooling it rapidly.Show Answer
(i) Tetra phosphorus decoxide $\left(\mathrm{P_4} \mathrm{O_10}\right) \quad$
(ii) Ammonium phosphate $\left(\mathrm{NH_4}\right)_{3} \mathrm{PO_4} \quad$
(iii) $\mathrm{SiC}$
(ix) $\mathrm{Rb}$
(iv) $\mathrm{I_2}$
(v) $\mathrm{P_4}$
(vi) Plastic
(vii) Graphite
(viii) Brass
(xi) $\mathrm{Si}$
(x) $\mathrm{LiBr}$
Answer Ionic $\rightarrow$ (ii)Ammonium phosphate $\left(\mathrm{NH_4}\right)_{3} \mathrm{PO_4}$, (x) LiBr Metallic $\rightarrow$ (viii)Brass, (ix)Rb Molecular $\rightarrow$ (i) Tetra phosphorus decoxide $\left(\mathrm{P_4} \mathrm{O_10}\right)$, (iv) $\mathrm{I_2},(\mathrm{v}) \mathrm{P_4}$. Covalent (network) $\rightarrow$ (iii) SiC, (vii)Graphite, (xi) Si Amorphous $\rightarrow$ (vi)PlasticShow Answer
(ii) What is the coordination number of atoms:
(a) in a cubic close-packed structure?
(b) in a body-centred cubic structure?
Answer (i) The number of nearest neighbours of any constituent particle present in the crystal lattice is called its coordination number.
(ii) The coordination number of atoms (a)in a cubic close-packed structure is 12 , and (b) in a body-centred cubic structure is 8Show Answer
Answer By knowing the density of an unknown metal and the dimension of its unit cell, the atomic mass of the metal can be determined. Let ’ $a$ ’ be the edge length of a unit cell of a crystal, ’ $d$ ’ be the density of the metal, ’ $m$ ’ be the mass of one atom of the metal and ’ $z$ ’ be the number of atoms in the unit cell. Now, density of the unit cell $$
\begin{equation*}
=\frac{\text { Mass of the unit cell }}{\text { Volume of the unit cell }}
\end{equation*}
$$ $$\Rightarrow d=\frac{z m}{a^{3}}\tag{i}$$ [Since mass of the unit cell $=$ Number of atoms in the unit cell $\times$ mass of one atom] [Volume of the unit cell $=(\text { Edge length of the cubic unit cell })^{3}$ ] From equation ( $i$ ), we have: $$
\begin{equation*}
m=\frac{d a^{3}}{z} \tag{ii}
\end{equation*}
$$ Now, mass of one atom of metal $(m)$ $$
=\frac{\text { Atomic mass }(\mathrm{M})}{\text { Avogadro’s number }\left(\mathrm{N}_{\mathrm{A}}\right)}
$$ Therefore, $$
\begin{equation*}
\mathrm{M}=\frac{d a^{3} \mathrm{~N}_{\mathrm{A}}}{z} \tag{iii}
\end{equation*}
$$ If the edge lengths are different (say $a, b$ and $c$ ), then equation (ii) becomes: $M=d(a b c) N_{A}$Show Answer
Answer Higher the melting point, greater is the intermolecular force of attraction and greater is the stability. A substance with higher melting point is more stable than a substance with lower melting point. The melting points of the given substances are: Solid water $\rightarrow 273 \mathrm{~K}$ Ethyl alcohol $\rightarrow 158.8 \mathrm{~K}$ Diethyl ether $\rightarrow 156.85 \mathrm{~K}$ Methane $\rightarrow 89.34 \mathrm{~K}$ Now, on observing the values of the melting points, it can be said that among the given substances, the intermolecular force in solid water is the strongest and that in methane is the weakest.Show Answer
(i) Hexagonal close-packing and cubic close-packing?
(ii) Crystal lattice and unit cell?
(iii) Tetrahedral void and octahedral void?
Answer i. A 2-D hexagonal close-packing contains two types of triangular voids ( $a$ and $b$ ) as shown in figure 1. Let us call this 2-D structure as layer A. Now, particles are kept in the voids present in layer A (it can be easily observed from figures 2 and 3 that only one of the voids will be occupied in the process, i.e., either a or b). Let us call the particles or spheres present in the voids of layer A as layer B. Now, two types of voids are present in layer B (c and d). Unlike the voids present in layer A, the two types of voids present in layer B are not similar. Void $c$ is surrounded by 4 spheres and is called the tetrahedral void. Void $\mathrm{d}$ is surrounded by 6 spheres and is called the octahedral void. Now, the next layer can be placed over layer B in 2 ways. Case 1: When the third layer (layer C) is placed over the second one (layer B) in such a manner that the spheres of layer $\mathrm{C}$ occupy the tetrahedral voids $\mathbf{c}$. In this case we get hexagonal close-packing. This is shown in figure 4. In figure 4.1, layer B is present over the voids a and layer $\mathrm{C}$ is present over the voids $\mathrm{C}$. In figure 4.2, layer B is present over the voids b and layer $\mathrm{C}$ is present over the voids $\mathrm{c}$. It can be observed from the figure that in this arrangement, the spheres present in layer $\mathrm{C}$ are present directly above the spheres of layer A. Hence, we can say that the layers in hexagonal close-packing are arranged in an $A B A B . .$. pattern. Case 2: When the third layer (layer C) is placed over layer B in such a manner that the spheres of layer C occupy the octahedral voids $d$. In this case we get cubic close-packing. In figure 5.1, layer B is present over the voids a and layer C is present over the voids $d$. In figure 5.2, layer $B$ is present over the voids $b$ and layer $C$ is present over the voids $d$. It can be observed from the figure that the arrangement of particles in layer $\mathrm{C}$ is completely different from that in layers $\mathrm{A}$ or $\mathrm{B}$. When the fourth layer is kept over the third layer, the arrangement of particles in this layer is similar to that in layer A. Hence, we can say that the layers in cubic close-packing are arranged in an ABCABC….. pattern.Show Answer




(i) Face-centred cubic
(ii) Face-centred tetragonal
(iii) Body-centred
Answer (i) There are 14 (8 from the corners + 6 from the faces) lattice points in face-centred cubic. (ii) There are 14 (8 from the corners +6 from the faces) lattice points in face-centred tetragonal. (iii) There are 9 (1 from the centre +8 from the corners) lattice points in body-centred cubic.Show Answer
(i) The basis of similarities and differences between metallic and ionic crystals.
(ii) Ionic solids are hard and brittle.
Answer (i) The basis of similarities between metallic and ionic crystals is that both these crystal types are held by the electrostatic force of attraction. In metallic crystals, the electrostatic force acts between the positive ions and the electrons. In ionic crystals, it acts between the oppositely-charged ions. Hence, both have high melting points. The basis of differences between metallic and ionic crystals is that in metallic crystals, the electrons are free to move and so, metallic crystals can conduct electricity. However, in ionic crystals, the ions are not free to move. As a result, they cannot conduct electricity. However, in molten state or in aqueous solution, they do conduct electricity. (ii) The constituent particles of ionic crystals are ions. These ions are held together in three-dimensional arrangements by the electrostatic force of attraction. Since the electrostatic force of attraction is very strong, the charged ions are held in fixed positions. This is the reason why ionic crystals are hard and brittle.Show Answer
(i) simple cubic
(ii) body-centred cubic
(iii) face-centred cubic (with the assumptions that atoms are touching each other).
Answer (i) Simple cubic In a simple cubic lattice, the particles are located only at the corners of the cube and touch each other along the edge. Let the edge length of the cube be ‘a’ and the radius of each particle be $r$. So, we can write: $a=2 r$ Now, volume of the cubic unit cell $=a^{3}$ $=(2 r)^{3}$ $=8 r^{3}$ We know that the number of particles per unit cell is 1 . Therefore, volume of the occupied unit cell $=\frac{4}{3} \pi r^{3}$ Hence, packing efficiency $$
=\frac{\text { Volume of one particle }}{\text { Volume of cubic unit cell }} \times 100 \%
$$ $$
\begin{aligned}
& =\frac{\frac{4}{3} \pi r^{3}}{8 r^{3}} \times 100 \% \\
& =\frac{1}{6} \pi \times 100 \% \\
& =\frac{1}{6} \times \frac{22}{7} \times 100 \% \\
& =52.4 \%
\end{aligned}
$$ (ii) Body-centred cubic Itcan be observed from the above figure that the atom at the centre is in contact with the other two atoms diagonally arranged. From $\triangle \mathrm{FED}$, we have: $b^{2}=a^{2}+a^{2}$ $\Rightarrow b^{2}=2 a^{2}$ $\Rightarrow b=\sqrt{2} a$ Again, from $\triangle A F D$, we have: $c^{2}=a^{2}+b^{2}$ $\Rightarrow c^{2}=a^{2}+2 a^{2} \quad\left(\right.$ Since $\left.b^{2}=2 a^{2}\right)$ $\Rightarrow c^{2}=3 a^{2}$ $\Rightarrow c=\sqrt{3} a$ Let the radius of the atom be $r$. Length of the body diagonal, $c=4 \pi$ $\Rightarrow \sqrt{3} a=4 r$ $\Rightarrow a=\frac{4 r}{\sqrt{3}}$ $r=\frac{\sqrt{3} a}{4}$ Volume of the cube, $$
a^{3}=\left(\frac{4 r}{\sqrt{3}}\right)^{3}
$$ A body-centred cubic lattice contains 2 atoms. So, volume of the occupied cubic lattice $=2 \pi \frac{4}{3} r^{3}$ $=\frac{8}{3} \pi r^{3}$ $\therefore$ Packing efficiency $=\frac{\text { Volume occupied by two spheres in the unit cell }}{\text { Total volume of the unit cell }} \times 100 \%$ $=\frac{\frac{8}{3} \pi r^{3}}{\left(\frac{4}{\sqrt{3}} r\right)^{3}} \times 100 \%$ $=\frac{\frac{8}{3} \pi r^{3}}{\frac{64}{3 \sqrt{3}} r^{3}} \times 100 \%$ $=68 \%$ (iii) Face-centred cubic Let the edge length of the unit cell be ’ $a$ ’ and the length of the face diagonal AC be $b$. From $\triangle \mathrm{ABC}$, we have: $\mathrm{AC}^{2}=\mathrm{BC}^{2}+\mathrm{AB}^{2}$ $\Rightarrow b^{2}=a^{2}+a^{2}$ $\Rightarrow b^{2}=2 a^{2}$ $\Rightarrow b=\sqrt{2 a}$Show Answer



Answer It is given that the edge length, $a=4.077 \times 10^{-8} \mathrm{~cm}$ Density, $d=10.5 \mathrm{~g} \mathrm{~cm}^{-3}$ As the lattice is fcc type, the number of atoms per unit cell, $z=4$ We also know that, $\mathrm{N}_{\mathrm{A}}=6.022 \times 10^{23} \mathrm{~mol}^{-1}$ Using the relation: $$
\begin{aligned}
& d=\frac{z \mathrm{M}}{a^{3} \mathrm{~N_\mathrm{A}}} \\
& \begin{aligned}
& \Rightarrow \mathrm{M}=\frac{d a^{3} \mathrm{~N_\mathrm{A}}}{z} \\
&=\frac{10.5 \mathrm{gcm}^{-3} \times\left(4.077 \times 10^{-8} \mathrm{~cm}\right)^{3} \times 6.022 \times 10^{23} \mathrm{~mol}^{-1}}{4} \\
&=107.13 \mathrm{gmol}^{-1}
\end{aligned}
\end{aligned}
$$ Therefore, atomic mass of silver $=107.13 \mathrm{u}$Show Answer
Answer It is given that the atoms of $\mathrm{Q}$ are present at the corners of the cube. Therefore, number of atoms of $\mathrm{Q}$ in one unit cell $$
=8 \times \frac{1}{8}=1
$$ It is also given that the atoms of $\mathrm{P}$ are present at the body-centre. Therefore, number of atoms of $\mathrm{P}$ in one unit cell $=1$ This means that the ratio of the number of $P$ atoms to the number of $Q$ atoms, $P: Q=1: 1$ Hence, the formula of the compound is $\mathrm{PQ}$. The coordination number of both $\mathrm{P}$ and $\mathrm{Q}$ is 8.Show Answer
Answer It is given that the density of niobium, $d=8.55 \mathrm{~g} \mathrm{~cm}^{-3}$ Atomic mass, $\mathrm{M}=93 \mathrm{gmol}^{-1}$ As the lattice is bcc type, the number of atoms per unit cell, $z=2$ We also know that, $\mathrm{N}_{\mathrm{A}}=6.022 \times 10^{23} \mathrm{~mol}^{-1}$ Applying the relation: $$
\begin{aligned}
d= & \frac{z \mathrm{M}}{a^{3} \mathrm{~N_\mathrm{A}}} \\
\Rightarrow a^{3} & =\frac{z \mathrm{M}}{d \mathrm{~N_\mathrm{A}}} \\
& =\frac{2 \times 93 \mathrm{gmol}^{-1}}{8.55 \mathrm{gcm}^{-3} \times 6.022 \times 10^{23} \mathrm{~mol}^{-1}}
\end{aligned}
$$ $=3.612 \times 10^{-23} \mathrm{~cm}^{3}$ So, $a=3.306 \times 10^{-8} \mathrm{~cm}$ For body-centred cubic unit cell: $
\begin{aligned}
r & =\frac{\sqrt{3}}{4} a \\
& =\frac{\sqrt{3}}{4} \times 3.306 \times 10^{-8} \mathrm{~cm}
\end{aligned}
$ $=1.432 \times 10^{-8} \mathrm{~cm}$ $=14.32 \times 10^{-9} \mathrm{~cm}$ $=14.32 \mathrm{~nm}$Show Answer
Answer A sphere with centre $\mathrm{O}$, is fitted into the octahedral void as shown in the above figure. It can be observed from the figure that $\triangle \mathrm{POQ}$ is right-angled $\angle \mathrm{POQ}=90^{\circ}$ Now, applying Pythagoras theorem, we can write: $
\begin{aligned}
& \mathrm{PQ}^{2}=\mathrm{PO}^{2}+\mathrm{OQ}^{2} \\
& \Rightarrow(2 \mathrm{R})^{2}=(\mathrm{R}+\mathrm{r})^{2}+(\mathrm{R}+\mathrm{r})^{2} \\
& \Rightarrow(2 \mathrm{R})^{2}=2(\mathrm{R}+\mathrm{r})^{2} \\
& \Rightarrow 2 \mathrm{R}^{2}=(\mathrm{R}+\mathrm{r})^{2} \\
& \Rightarrow \sqrt{2} \mathrm{R}=\mathrm{R}+\mathrm{r} \\
& \Rightarrow \mathrm{r}=\sqrt{2} \mathrm{R}-\mathrm{R} \\
& \Rightarrow \mathrm{r}=(\sqrt{2}-1) \mathrm{R} \\
& \Rightarrow \mathrm{r}=0.414 \mathrm{R}
\end{aligned}
$Show Answer

Answer Edge length, $a=3.61 \times 10^{-8} \mathrm{~cm}$ As the lattice is fcc type, the number of atoms per unit cell, $z=4$ Atomic mass, $\mathrm{M}=63.5 \mathrm{~g} \mathrm{~mol}^{-1}$ We also know that, $\mathrm{N}_{\mathrm{A}}=6.022 \times 10^{23} \mathrm{~mol}^{-1}$ Applying the relation: $
\begin{aligned}
d & =\frac{z \mathrm{M}}{a^{3} \mathrm{~N}_{\mathrm{A}}} \\
& =\frac{4 \times 63.5 \mathrm{~g} \mathrm{~mol}^{-1}}{\left(3.61 \times 10^{-8} \mathrm{~cm}\right)^{3} \times 6.022 \times 10^{23} \mathrm{~mol}^{-1}}
\end{aligned}
$ $=8.97 \mathrm{~g} \mathrm{~cm}^{-3}$ The measured value of density is given as $8.92 \mathrm{~g} \mathrm{~cm}^{-3}$. Hence, the calculated density $8.97 \mathrm{~g} \mathrm{~cm}^{-3}$ is in agreement with its measured value.Show Answer
Answer The formula of nickel oxide is $\mathrm{Ni_{0.98}} \mathrm{O_{1.00}}$. Therefore, the ratio of the number of $\mathrm{Ni}$ atoms to the number of $\mathrm{O}$ atoms, $\mathrm{Ni}: \mathrm{O}=0.98: 1.00=98: 100$ Now, total charge on $100 \mathrm{O}^{2}$-ions $=100 \times(-2)$ $=-200$ Let the number of $\mathrm{Ni}^{2+}$ ions be $x$. So, the number of $\mathrm{Ni}^{3+}$ ions is $98-x$. Now, total charge on $\mathrm{Ni}^{2+}$ ions $=x(+2)$ $=+2 x$ And, total charge on $\mathrm{Ni}^{3+}$ ions $=(98-x)(+3)$ $=294-3 x$ Since, the compound is neutral, we can write: $2 x+(294-3 x)+(-200)=0$ $\Rightarrow-x+94=0$ $\Rightarrow x=94$ Therefore, number of $\mathrm{Ni}^{2+}$ ions $=94$ And, number of $\mathrm{Ni}^{3+}$ ions $=98-94=4$ Hence, fraction of nickel that exists as $\mathrm{Ni}^{2+}=\frac{94}{98}$ $=0.959$ And, fraction of nickel that exists as $\mathrm{Ni}^{3+}=\frac{4}{98}$
$=0.041$ Alternatively, fraction of nickel that exists as $\mathrm{Ni}^{3+}=1-0.959$ $=0.041$Show Answer
Answer Semiconductors are substances having conductance in the intermediate range of $10^{-6} \mathrm{to} 10^{4} \mathrm{ohm}^{-1} \mathrm{~m}^{-1}$. The two main types of semiconductors are: (i) $n$-type semiconductor (ii) p-type semiconductor n-type semiconductor: The semiconductor whose increased conductivity is a result of negatively-charged electrons is called an $n$-type semiconductor. When the crystal of a group 14 element such as $\mathrm{Si}$ or $\mathrm{Ge}$ is doped with a group 15 element such as $\mathrm{P}$ or As, an $n$-type semiconductor is generated. $\mathrm{Si}$ and $\mathrm{Ge}$ have four valence electrons each. In their crystals, each atom forms four covalent bonds. On the other hand, $\mathrm{P}$ and As contain five valence electrons each. When Si or Ge is doped with P or As, the latter occupies some of the lattice sites in the crystal. Four out of five electrons are used in the formation of four covalent bonds with four neighbouring $\mathrm{Si}$ or $\mathrm{Ge}$ atoms. The remaining fifth electron becomes delocalised and increases the conductivity of the doped Si or Ge. Perfect crystal $n$ - type p-type semiconductor: The semiconductor whose increased in conductivity is a result of electron hole is called a $p$ type semiconductor. When a crystal of group 14 elements such as $\mathrm{Si}$ or $\mathrm{Ge}$ is doped with a group 13 element such as $\mathrm{B}, \mathrm{Al}$, or $\mathrm{Ga}$ (which contains only three valence electrons), a p-type of semiconductor is generated. When a crystal of $\mathrm{Si}$ is doped with $\mathrm{B}$, the three electrons of $\mathrm{B}$ are used in the formation of three covalent bonds and an electron hole is created. An electron from the neighbouring atom can come and fill this electron hole, but in doing so, it would leave an electron hole at its original position. The process appears as if the electron hole has moved in the direction opposite to that of the electron that filled it. Therefore, when an electric field is applied, electrons will move toward the positively-charged plate through electron holes. However, it will appear as if the electron holes are positively-charged and are moving toward the negatively- charged plate. Perfect crystal $p$ - typeShow Answer




Answer In the cuprous oxide $\left(\mathrm{Cu}_{2} \mathrm{O}\right)$ prepared in the laboratory, copper to oxygen ratio is slightly less than 2:1. This means that the number of Cu+ions is slightly less than twice the number of $\mathrm{O}^{2}$ ions. This is because some Cu+ions have been replaced by $\mathrm{Cu}^{2+}$ ions. Every $\mathrm{Cu}^{2+}$ ion replaces two $\mathrm{Cu}^{+}$ions, thereby creating holes. As a result, the substance conducts electricity with the help of these positive holes. Hence, the substance is a p-type semiconductor.Show Answer
Answer Let the number of oxide $\left(\mathrm{O}^{2 \cdot}\right)$ ions be $x$. So, number of octahedral voids $=x$ It is given that two out of every three octahedral holes are occupied by ferric ions. So, number of ferric $\left(\mathrm{Fe}^{3+}\right)$ ions $=\frac{2}{3} x$ Therefore, ratio of the number of $\mathrm{Fe}^{3+}$ ions to the number of $\mathrm{O}^{2}$ ions, $\mathrm{Fe}^{3+}: \mathrm{O}^{2-}=\frac{2}{3} x: x$ $=\frac{2}{3}: 1$ $=2: 3$ Hence, the formula of the ferric oxide is $\mathrm{Fe} _{2} \mathrm{O} _{3}$.Show Answer
(i) Ge doped with In
(ii) Si doped with B.
Show Answer
Answer
(i) Ge (a group 14 element) is doped with In (a group 13 element). Therefore, a hole will be created and the semiconductor generated will be a $p$-type semiconductor.
(ii) B (a group 13 element) is doped with Si (a group 14 element). Thus, a hole will be created and the semiconductor generated will be a $p$-type semiconductor.










