Chapter 05 Heating And Chemical Effects Of Current
Introduction
Heating and chemical effects are the most important effects of electric current. Heating effect has many day-to-day applications for us. We use electric iron to press our clothes, room heater to get rid of cold, electrical lamps to lit our rooms, etc. We access these facilities due to heating effect of current. In these phenomena, electrical energy is converted into heat energy.
The other effect of electric current is to produce the chemical change. This effect is called the chemical effects of electric current. This chapter is based on the two effects of electric current.
ELECTRIC POWER
If a battery is used to establish an electric current in a conductor, these is a continuous transformation of chemical energy stored in the buttery to kinetic energy of the change carriers. This kinetic energy is quickly lost as a result of collisions between the charge carriers and the lattice ions, resulting in an increase in the temperature of the conductor. Therefore, we see that the chemical energy stored in the battery is continuously transformed into heat energy.
Consider a simple circuit vconsisting of a battery whose terminals are connected to a resistor R, as shown in figure 5.1.
The positive terminal of the battery (the longer plate) is at the higher potential, while the negative terminal (the shorter plate) is at the lower potential. Now imagine a positive quantity of charge
The rate at which the charge
where
P = IV ………………… (1)
In this case, the power is supplied to a resistor by a battery. However, eq. (1) can be used to determine the power transferred to any device carrying a current
Using eq. (1) and the fact that
When
Watt is a small unit of power, its other bigger units are Kilowatt, Megawatt and Horsepower.
If
The value of electricity consumed in houses is decided on the basis of the total electric energy used. Electric power tells us about the electric energy used per second not the total electric energy.
The total energy used in a circuit
If the power is in watt and time is in second, then the electric energy will be in joule or watt
As the watt
1 Kilowatt hour
The electricity department uses this unit in the electric bills. If the 100 watt bulb or any other electric appliance is used for an hour then the total electric energy consumed is 1 kWh or 1 unit.
CHECK POINT:
The wattage marked on a light bulb is not an inherent property of the bulb; rather, it depends on the voltage to which it is connected, usually 110 or 120V. How many amperes flow through a 60-W bulb connected in a 120-V elrcult?
Show Answer
SOLUTION
Using the formula,
Watt
or,
Thus, a 0.5 A current flows through a 60-W bulb connected in a 120-V circuit
ILLUSTRATION-5.1
An immersion rod of 1500 W is used everyday for 3 hours to boil water. If the rate of 1 unit of electric energy is
Show Answer
SOLUTION:
Electric energy used in a day
Electric energy used in 30 days
Total energy in
Hence the total cost of the electricity
ILLUSTRATION-5.2
An electric heater is rated
Show Answer
SOLUTION:
ILLUSTRATION-5.3
Calculate the monthly bill for a heater of resistance
Show Answer
SOLUTION:
Power of heater
ILLUSTRATION-5.4
Rearrange the equation
Current
to express resistance in terms of current and voltage. Then solve the following: A certain device in a 120-V circuit has a current rating of
Show Answer
SOLUTION:
Current
Rearranging, we get
Current
or, Resistance
Now, Resistance
Therefore, the resistance of the device is 6 ohms.
HEAT PRODUCED DUE TO ELECTRICITY
When a current passes through a conductor, heat is produced in it due to the collisions between the free electrons and the atoms of the conductor. The heat produced
1. Resistance of conductor : More the resistance of conductor more is the number of collisions between free electrons and atoms. Hence if a conductor offers more resistance
PRACTICAL APPLICATION OF HEATING EFFECT
The heat generated when current passes through a resistive material is used in many common devices. The material through which the current passes is surrounded by an insulating substance in order to prevent the current from flowing through the cook to the earth when he or she touches the pan. A material that is a good conductor of heat surrounds the insulator
Hair dryer, in which a fan blows air past heating coils. In this case the warm air can be used to dry hair, but on a broader scale this same principle is used to dry clothes and to heat buildings.
A final example of a household uses the heating effect of electric current is the steam iron. A heating coil warms the bottom of the iron and simultaneously turns water to steam, which is sprayed from jets located in the bottom of the iron.
Other practical application includes heater, toaster, electric kettle.
CHEMICAL EFFECT OF CURRENT
Electric current can produce chemical change. This ability of current is called chemical effect. This effect is shown by D.C. only. A.C. current can’t produce chemical effect.
FARADAY’S LAWS OF ELECTROLYSIS
(i) 1st law
The mass of the substance liberated or deposited at an electrode during electrolysis is dircetly proportional to the quantity of charge passed through the electrolyte i.e.
mass or m = Zq = Z It, where Z = electrochemical equivalent (E.C.E.) of substance.
(ii) 2nd law
When the same amount of charge is passed through different electrolytes, the masses of the substance liberated or deposited at the various electrodes are proportional to their chemical equivalents i.e:
FARADAY CONSTANT
(i) Faraday constant is equal to the amount of charge required to liberate the mass of a substance at an clectrode during electrolysis, equal to its chemical equivalent in gram (i.e. one gram equivalent)
(ii) One faraday = 1F = 96500 C/gram equivalent.
ELECTROCHEMICAL CELL
An electrochemical cell is a device which by converting chemical energy into electrical energy maintains the flow of charge in a circuit. It usually consists of two electrodes of different materials and an electrolyte. The electrode at higher potential is called anode and the one at lower potential is cathode
PRIMARY CELLS
The cells which cannot be recharged electrically are called primary cells. Here the original state of cell cannot be brought back by passing electrical energy through cell from external source after cell is discharged.
e.g. Voltaic cell, Daniel cell, Leclanche cell, Manganese-alkaline cell, Mercury button cell etc.
SECONDARY CELLS
The cells in which chemical process is reversible are called secondary cells. Here original chemical state of cell can be brought back by passing electrical energy through cell from external source.
e.g. Lead acid accumulator, alkali cells etc.
ELECTROMOTIVE FORCE (EMF)
The emf of a cell is defined as work done by cell in moving a unit positive charge in the whole circuit including the cell once emf
Its SI unit is joule/coulomb or volt.
Emf is the maximum potential difference between the two electrodes of the cell when no current is drawn from the cell. It is the characteristic property of cell and depends on the nature of electrodes and electrolyte used in cell, and is independent of quantity of electrolyte, size of electrodes and distance between the electrodes.
INTERNAL RESISTANCE OF CELL
The opposition offered by the electrolyte of the cell to the flow of electric current through it is called the internal resistance of the cell. The internal resistance of cell depends on.
Distance between electrodes
Conductivity or nature of electrolyte
Concentration of electrolyte
Nature and area of electrodes dipped in electrolyte
TERMINAL POTENTIAL DIFFERENCE
The potential difference between the two electrodes of a cell in a closed circuit i.e., when current is being drawn from the cell is called terminal potential difference.
(a) When cell is discharging
When cell is discharging current inside the cell is from anode to cathode .
Current
When current is drawn from the cell, potential difference is less than emf of cell. Greater is the current drawn from the cell smaller is the terminal voltage. When a large current is drawn from a cell, its terminal voltage is reduced.
(b) When cell is charging
When cell is charging current inside the cell is from anode to cathode.
Fig. 5.4
Current
During charging terminal potential difference is greater than emf of cell.
(c) When cell is in open circuit
In open circuit
In open circuit terminal potential difference is equal to emf and is the maximum potential difference which a cell can provide.
(d) When cell is short circuited
In short circuit
In short circuit current from cell is maximum and terminal potential difference is zero.
(e) Power transferred to load by cell
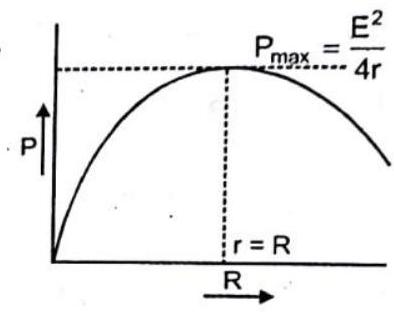
Fig.5.5
Power transferred by cell to load is maximum when
DIFFERENCE BETWEEN EMF AND POTENTIAL DIFFERENCE
| EMFOFACELL | POTENTIAL DIFFERENCE | ||
|---|---|---|---|
| 1. | The emf of a cell is the maximum potential difference between the two electrodes of a cell when the cell is in the open circuit. | 1. | The potential difference between the two points is the difference of potential between these two points in a closed circuit. |
| 2. | Emf is independent of resistance of circuit and depends upon the nature of electrodes and electrolyte. | 2. | This depends upon the resistance between two points of the circuit and current flowing through the circuit. |
| 3. | The term emf is used for source of current. | 3. | Potential difference can be measured between any two points of circuit. |
| 4. | This is a cause. | 4. | It is an effect. |
ILLUSTRATION-5.5
A dry cell of e.m.f.
When the circuit is switched on, the ammeter reading settles to a steady value of 2.0 A, What is the steady
(i) rate of chemical energy consumption of the cell.
(ii) rate of energy dissipation inside the cell.
(iii) rate of energy dissipation inside the resistor and
(iv) power output of the source?
Show Answer
SOLUTION:
Let
(i) The rate of consumption of chemical energy of the cell
(ii) The rate of energy dissipation inside the internal resistor
(iii) The rate of energy dissipation inside the external resistor
(iv) The power output of the source
Rate of energy dissipation inside the cell
ILLUSTRATION-5.6
Does more current flow out of a battery than into it? Does more current flow into a light bub than out of it? Explain.
Show Answer
SOLUTION:
The current that flow out of a battery is always equal to the current that flow into it.
Similarly, the current that flows into a light bulb is always equal to the current that flows out of it. Although there will be a voltage drop due to the resistance provided to the flow of current due to both the battery and the resistance, but the amount of current won’t be affected.
GROUPING OF CELLS
(i) Series Combination
The cells are said to be connected in series if negative terminal of first is connected to positive of second whose negative terminal is connected to positive of third cell. The external resistance is connected between free terminals of first and last cells.
Let
If
If
Maximum current can be drawn from series combination of cells if external resistance is very large as compared to equivalent internal resistance.
If in series combination of
If unidentical cells are connected in series then
(ii) Parallel Combination
The cells are said to be connected in parallel if positive terminals of all the cells are connected tagether at one point and their negative terminals at another point. The external resistor is connected between these two points.
Let
If
If
Thus maximum current can be drawn from parallel combination of cells if external resistance is small as compared to net internal resistance of cells.
(iii) Mixed Combination
If the
and the electrical power delivered to the load is
which is maximum under the condition of total internal and external resistances being equal, i.e.,
ILLUSTRATION-5.7
Four identical cells, each of emf
Show Answer
SOLUTION:
The complete circuit diagram, consisting of the 4 identical cells and the two external resistances in parallel, is shown. The total emf in the circuit is
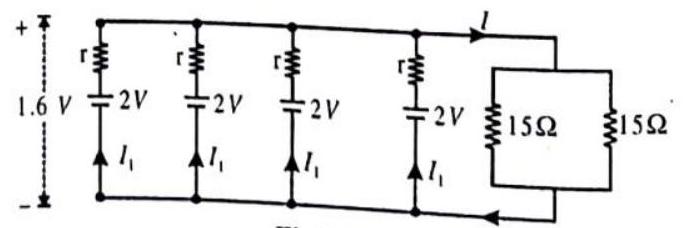
Fig.5.11
and the terminal voltage across the cells is
ILLUSTRATION-5.8
If 64 cells of emf
Show Answer
SOLUTION:
The current through the external resistance
where
DOMESTIC ELECTRIC CIRCUITS
Electricity from power stations is transmitted to homes through high voltage power lines. Electric potentials as high as 220000 V
Fuses and Circuit Breakers
A fuse is a safety device that acts as a switch. Fuses are connected into the circuit close to the source. As a result all the current flowing in the circuit passes through the fuse. When too much current flows, the fuse heats up sufficiently to bum out. This creates an air gap and no more current flows. The construction of a plug fuse is shown in Figure. Other kinds of fuses are cartridge fuses and knife blade fuses. The essential part is the fuse wire or a blade with a low melting point.
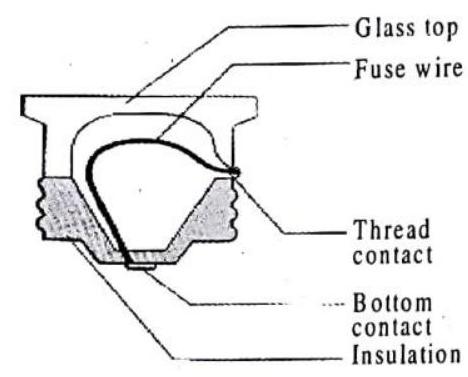
Fig. 5.12 : A plug fuse
Most homes built in recent years use circuit breakers instead of fuses in their electrical panels. A circuit breaker is connected in the same place as a fuse would be, and it performs the same function. Unlike a fuse, however, it does not bum out. When too much current flows, the circuit breaker simply opens like a switch. Then, when the problem that caused the excess current has been corrected, you can close the breaker again.
The Circuit Breaker Panel
At the home, the electricity first passes through an electric meter. The electric meter records the amount of electric energy used in the home. Then the electricity enters a circuit breaker panel. (In some older homes it may enter a fuse panel.) In this panel there is a master circuit breaker. The master circuit breaker can be operated manually to cut off the electricity to the entire home. It opens automatically if the current exceeds the -rated value. In homes that have a
There are three strips of conducting metal called bus bars in the breaker panel. The red wire is connected to one, the black wire to another, and the white wire to the third. As a result, two of the bus bars are live and one is neutral.
Electric Circuits in the Home
All the electric circuits within the home originate from the bus. Figure shows a
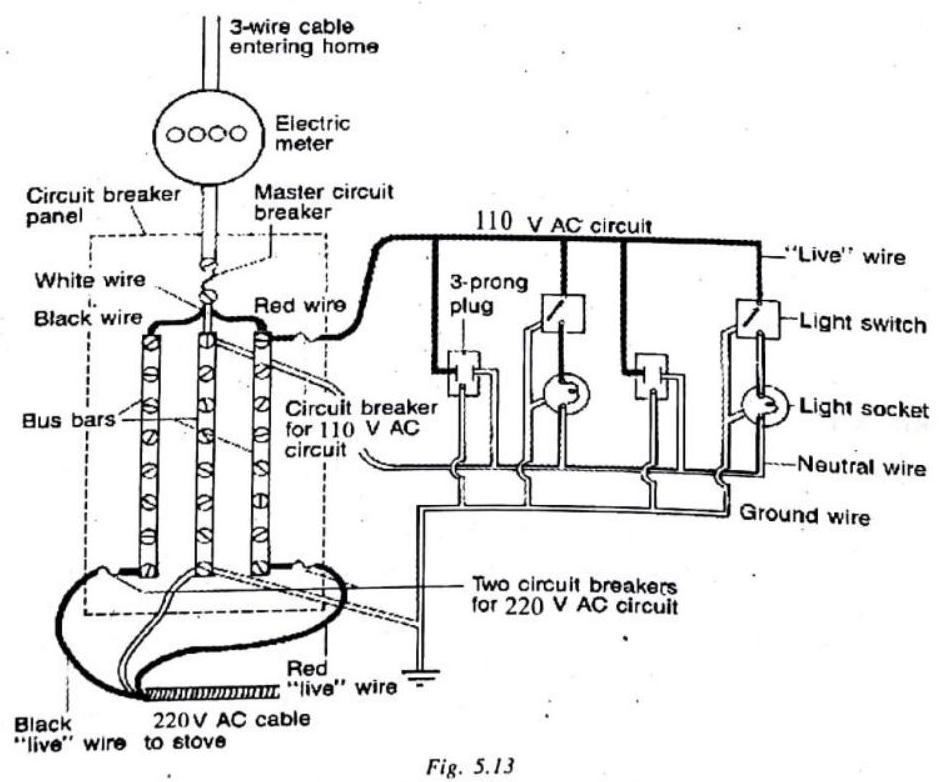
A
Loads in the same circuit, such as wall outlets and lights, are always connected in parallel. If one load bums out the others still operate. Also, as more and more loads are turned on, the electrical potential remains the same. However, the electric current increases. But one load does not affect another unless the circuit becomes overloaded.
Causes of Overloaded Circuits
There are two main causes of overloaded circuits. First, an appliance or a conducting wire can develop a short circuit. Now the current is no longer limited by the load. In this case, current passes from the live wire to the neutral wire or from the live wire to the ground wire without passing through the load. The current becomes very large very fast. This heats up the circuit. Second, too many appliances can be plugged into the same circuit. Each appliance draws a certain current. The combination can draw too much current and the circuit again heats up.
A circuit breaker is designed to prevent overloading. It trips and opens the circuit as soon as the circuit becomes too hot.
CHECK POINT:
Why are devices in household circuits almost never connected in series?
Show Answer
SOLUTION:
The devices in household circuits are never connected in series because if they are connected so they can’t be turned on of separately as required. If one of the device will be turned off then all of them will also be turned off. Moreover, if the devices are lighting one, their brightness will decrease if more devices will be added.
ILLUSTRATION-5.9
An immersion heater having
Show Answer
SOLUTION:
Current passing through the heater
Heat produced
Heat produced
Since
ILLUSTRATION-5.10
An electric refrigerator rated
Show Answer
SOLUTION:
The total energy consumed by the refrigerator in 30 days would be
Thus the cost of energy to operate the refrigerator for 30 days is
ILLUSTRATION-5.11
What is the operating resistance of a 100-watt household light bulb ? The operating line voltage of household electricity is
Show Answer
SOLUTION:
Given :
From
ILLUSTRATION-5.12
On a
Show Answer
SOLUTION:
The resistance of the
The resistance of the
When the bulbs are connected in parallel, their equivalent resistance is
The power of the parallel combination is
When the bulbs are connected in series, their equivalent resistance is
The power is
ILLUSTRATION-5.13
An electric kettle has coils A and B. When coil A is switched on, the water boils in 10 minutes, and when coil
Show Answer
SOLUTION:
Let
where
Here
(a) When the coils are connected in series, the equivalent resistance is
If
(b) When the coils are connected in parallel, the equivalent resistance is
ILLUSTRATION-5.14
Two bulbs A and B are rated
Show Answer
SOLUTION:
Resistance of bulb A (rating 100W, 120V) is
Resistance of bulb B (rating 10W, 120V) is
When they are connected in series,
Total resistance,
Current in the circuit
Same current passes through each bulb.
Power consumed in the bulb
Power consumed in the bulb B is
Since,
Since,
ILLUSTRATION-5.15
There are 5 rooms in a house. Each room has a
Show Answer
SOLUTION:
Energy used every day in 5 bulbs
Energy used everyday in 5 tube lights
Total energy used everyday by bulb and tube light
Total energy used in 30 day
Cost of the total energy used
ILLUSTRATION-5.16
Compute the heat generated while transferring 96000 coulomb of charge in one hour through a potential difference of
Show Answer
SOLUTION:
Here,
Current,
Resistance,
Heat produced,
ILLUSTRATION-5.17
When a
Show Answer
SOLUTION:
Potential difference,
Current,
We know that,
ILLUSTRATION-5.18
Two lamps, one rated
Show Answer
SOLUTION:
We know that,
Resistance of 1st lamp,
Resistance of 2nd lamp,
Since, two lamps are connected in parallel, so its equivalent resistance is given by
Current drawn from the line,
ILLUSTRATION-5.19
A current of
Show Answer
SOLUTION:
If
ILLUSTRATION-5.20
Calculate the current flowing through a nichrome wire, immersed in
Show Answer
SOLUTION:
Energy produced in wire
Energy absorbed by oil
ILLUSTRATION-5.21
Two coils of resistance
Show Answer
SOLUTION:
The circuit is shown in figure.
Total resistance of the circuit
Current in the circuit
Since the resistance are in series, same current flows in each resistance.
Electrical energy spent in
Electrical energy spent in
MISCELLANEOUS
SOLVED EXAMPLES
1. A bulb has voltage rating of
Show Answer
Solution:
Here
Resistance of the bulb,
Current in the circuit,
Potential difference across the bulb,
As per question,
On solving, we get
2. If two bulbs of wattage 25 and
Show Answer
Solution: Resistance of
Resistance of
When bulbs are connected in series, effective resistance is
When supply voltage is
As
3. Fig. shows three similar lamps
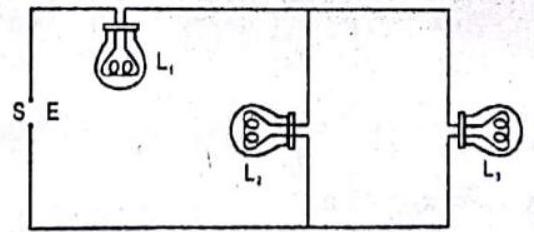
If the lamp
Show Answer
Solution: Let
Current flowing through
When
Thus,
So, current through
4. 1kW heat is to be used with
Assume that heat loses due to radiation are negligible. Latent heat of steam
Show Answer
Solution: (i)
(ii)
(iii)
(iv)
(v) Let
5. A fuse wire with a circular cross section and having diameter of 0.4 mm blows with a current of 3 amp. The value of the current for which another fuse wire made of the same material but having circular cross-section with diameter of 0.06 mm will blow is
Show Answer
Solution: For a fuse wire
where
or
6. An accumulator is connected first to an external resistance
Show Answer
Solution: When an accumulator of emf
According to given problem :
And as
7. How will you connect 24 cells each of internal resistance
Show Answer
Solution: For maximum power output
and
So
or
(a) If
so
(b) If
so
So to get maximum output power cells must be arranged in two rows having 12 cells in each row.
8. If electrochemical equivalent of hydrogen is
Show Answer
Solution:
We know that,
9. Find the mass of silver liberated in a silver voltameter carrying a current of
Show Answer
Solution: Here,
and
Using
mass of silver liberated is
10. In a water voltameter, the act of passing a certain amount of current for a certain time produces of
Show Answer
Solution: Since the same current flows through both the electrodes of a water voltameter, so the amount of oxygen and hydrogen liberated (for the same charge) will be in direct proportional to their respective equivalent weights by Faraday’s second law of electrolysis,
i.e.,
1 EXERCISE
Fill in the Blanks
DIRECTIONS : Complete the following statements with an appropriate word / term to be filled in the blank space(s).
1. Kilowatt is the unit of electrical …………… but kilowatt-hour is the unit of electrical ……………
Show Answer
Answer: power, energy2. Energy spent in kilowatt-hour
Show Answer
Answer: ampere, hour3. A fuse is a short piece of wire of high …………… and low ……………
Show Answer
Answer: resistance, melting point4. Fuse wire has a …………… melting point and is made of an alloy of …………… and …………… If the current in a circuit rises too high, the fuse wire ……………
Show Answer
Answer: low, lead, tin, melts5. A fuse is connected in …………… to the …………… wire.
Show Answer
Answer: series, live6. Electric energy is produced by the …………… of charges.
Show Answer
Answer: separation7. Energy converted per unit charge is measured with an instrument called a (
Show Answer
Answer: voltmeter8. The electrinal energy dissipated in a resistor is given by
Show Answer
Answer:9. The unit of power is ……………
Show Answer
Answer: watt (W)10. One watt of power is consumed when
Show Answer
Answer: 1V11. 1 kWh = ……………
Show Answer
Answer: 3,600,000 J12. The alloy which is used for making the filament of bulbs is ……………
Show Answer
Answer: Tungsten13. Power transmission is carried out at high …………… and low ……………
Show Answer
Answer: voltage, current14. Rate at which electric work is done is called ……………
Show Answer
Answer: electric power15. The process of depositing a thin layer of desired metal over another metal by passing an electric current through some electrolyte is called ……………
Show Answer
Answer: electroplating16. For a given cell, its terminal voltage depends on …………… and ……………
Show Answer
Answer: external resistance, internal resistanceTrue / False
DIRECTIONS : Read the following statements and write your answer as true or false.
1. The filament resistance of glowing bulb is greater, to its resistance when it is not glowing?
Show Answer
Answer: True2. The commercial unit of electrical energy is kilowatt-hour (kWh).
Show Answer
Answer: True3. Pure tungsten has high resistivity and a high melting point (nearly
Show Answer
Answer: True4. When a metallic conductor is heated the atoms in the metal vibrate with greater amplitude and frequency.
Show Answer
Answer: True5. The used up chemicals in a dry cell can be reobtained by charging it.
Show Answer
Answer: False6. In an electrolyte, the movement of ions is responsible for electric current.
Show Answer
Answer: True7. Electrotyping is an application of electrolysis.
Show Answer
Answer: True8. One kilowatt is equal to 10 horse power.
Show Answer
Answer: False9. The mass of the element deposited at the electrode when one coulomb of charge is passed through the electrolyte is called electrochemical equivalent of the element.
Show Answer
Answer: True10. The terminal voltage of a cell in open circuit condition is less than its emf
Show Answer
Answer: False11. Fuse should be connected to phase wire of the circuit.
Show Answer
Answer: True12. Fuse is a thin wire which melts and breaks the electric circuit due to only high voltage.
Show Answer
Answer: False13. The difference between EMF (E) of the cell and terminal voltage
Show Answer
Answer: True14. The filament of bulb offers high resistance to electric current.
Show Answer
Answer: TrueMatch the Following
DIRECTIONS : Each question contains statements given in two columns which have to be matched. Statements (A, B, C, D) in column I have to be matched with statements (p, q, r, s) in column II.
1. Column II gives name of material use for device given in column I
| Column I | Column II | |||
|---|---|---|---|---|
| (A) | Resistance of resistance box | (p) | tungsten | |
| (B) | Fuse wire | (q) | maganin | |
| (C) | Bulb | (r) | tin-lead alloy | |
| (D) | Insulator | (s) | glass |
Show Answer
Answer:2. For the circuit shown in the adjoining figure, match the entries of column I with the entries of column II.
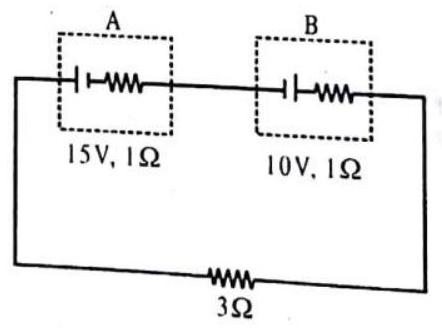
| Column I | Column II | |||
|---|---|---|---|---|
| (A) | Potential difference across battery A | (p) | A | |
| (B) | Potential difference across battery B | (q) | B | |
| (C) | Power is supplied by battery | (r) | 14 V | |
| (D) | Power is consumed by battery | (s) | None |
Show Answer
Answer:3. For a potentiometer circuit match the column
| Column I | Column II | |||
|---|---|---|---|---|
| (A) | Sensitivity | (p) | increases with length of wire | |
| (B) | Accuracy | (q) | decreases with length of wire | |
| (C) | Range | (r) | increases with emf of primary cell | |
| (D) | Potential gradient | (s) | maximum if internal resistance & rheostat resistance is zero. |
Show Answer
Answer:Very Short Answer Questions:
DIRECTIONS: Give answer in one word or one sentence.
1. What is a fuse and specify its uses?
Show Answer
Answer: Fuse is a thin wire which melts and breaks the electric circuit due to (i) overloading (ii) short circuit (iii) fluctuation in power supply.2. Find the total internal resistance of the cells when they are connected in
Show Answer
Answer: (a) In series connection, total internal resistance (
(b) In parallel connection, total internal resistance is equal to the sum of the reciprocals of individual internal resistances.
3. What is the use of kilowatt-hour meter?
Show Answer
Answer: Gives the reading of energy consumed.4. What is the value of mechanical equivalent of heat (J)?
Show Answer
Answer:5. Define terminal voltage, internal resistance and lost voltage.
Show Answer
Answer: (i) The P.D. between two terminals of the cell, when current is drawn from it, i.e., the cell is in closed circuit, is called its terminal voltage (V).
(ii) The electrolyte offers certain resistance to the flow of current. This is called the internal resistance of cell
(iii) The difference between
6. What is watt-hour?
Show Answer
Answer: Watt-hour is a unit in which the amount of electric energy consumed by a device is measured.7. Define electric energy or work.
Show Answer
Answer: The energy spent or work done an moving on electric charge through conductor is called electric energy or electric work8. Define electric power and give its units in S.I.
Show Answer
Answer: The rate at which electric work is done is called electric power. In S.I., power9. What is electro plating?
Show Answer
Answer: The process of depositing a thin layer of desired metal over another metal by passing an electric current through some electrolyte is called electro plating.10. How is the electric current passing though a metallic wire produces heat?
Show Answer
Answer: Colliding electrons lose their K.E. as heat.11. What is the operating resistance of a 100-watt household light bulb? The operating line voltage of household electricity is 120V.
Show Answer
Answer: Given :
From
12. Why are coils of electric toasters and electric irons made of an alloy rather than a pure metal ?
Show Answer
Answer: Coils of electric toasters and electric irons are made of an alloy rather than a pure metal because (i) the resistivity of an alloy is much higher than that of pure metal, and (ii) an alloy does not undergo oxidation easily even at high temperature.13. Why does the cord of an electric heater not glow while the heating element does ?
Show Answer
Answer: The cord of the electric heater made of copper does not glow because negligible heat is produced in it by passing current. It is due to its extremely low resistance.14. How does the amount of current in a household circuit differ from the amount of current in a reading lamp?
Show Answer
Answer: The amount of current in a household circuit is more than the amount of current in a reading lamp as the current of the circuit is divided among all the appliances in a parallel household circuit.15. Would you expect to find DC or AC in the filament of a light bulb in your home? How about in the headlight of an automobile?
Show Answer
Answer: There is16. A 220 volt 100 watts bulb is connected to 110 volts source, calculate the power consumed by the bulb.
Show Answer
Answer: Resistance of the bulb
Power consumed when connected to 110 volt source.
17. A current of 0.5 A is passing through a
Show Answer
Answer: If
18. An electric bulb is connected to a 220V generator. The current is 0.50V. What is the power of the bulb?
Show Answer
Answer:19. What uses more energy, a 250 W TV set in 1 hr. or a 1200W toaster in 10 minutes?
Show Answer
Answer:20. Name two devices which use the heating effect of current.
21. Why is the filament of an electric bulb not made of carbon?
22. 60W-220V is written on a bulb. What does it mean?
23. Name the term used to represent the values of the voltage and wattage (power) of an electrical appliance taken together.
Short Answer Questions
DIRECTIONS: Give answer in 2-3 sentences.
1. Obtain an expression for the (i) electrical energy (ii) electrical power, spent in flow of current through a conductor.
2. A 60W auto lamp allows 5 amps to pass through it. Find
(i) The p.d. across its terminal.
(ii) the resistance of the filament of the lamp
(iii) energy consumed in 2 hours
Show Answer
Answer: (i) Power, P = V
(ii) Resistance
(iii) Energy consumed in 2 hours
3. What is the electric power? Derive a formula for it?
4. An electric iron consumes energy at a rate of 840W when heating is at the maximum rate and 360W when the heating is at the minimum. The voltage is 220V. What are the current and the resistance in each case?
Show Answer
Answer: We know that the power input is,
Thus the current
(a) When heating is at the maximum rate,
and the resistance of the electric iron is
(b) When heating is at the minimum rate,
and the resistance of the electric iron is
5. A geyser is rated 1500W, 250V. This geyser is connected to 250V mains. Calculate: (i) the current drawn, (ii) the energy consumed in 50 hours. (iii) the cost of energy consumed at ‘220 per kWh.
Show Answer
Answer:6. An electric iron of resistance
Show Answer
Answer: Here,
Heat developed
7. Compare the power used in the
(i) a 6V battery in series with
Show Answer
Answer: (i) Equivalent resistance of
Potential difference,
Current in series circuit is same.
Power in
(ii) Potential difference across
Power,
8. An electric heater of resistance
Show Answer
Answer: Here,
Heat developed,
The rate at which heat is developed is power.
9. 100J of heat are produced each second in a
Show Answer
Answer:
We have the current through the resistor as
Thus the potential difference across the resistor
10. In the home electrical energy is converted into other energy forms. What is the energy conversion when
(a) an electric bell is used?
(b) an electric toaster is used ?
(c) a fluorescent tube is switched on ?
(d) an electric fan is used?
Show Answer
Answer: (a) electrical
(b) electrical sound
(c) electrical
(d) electrical
11. An immersion heater having
Show Answer
Answer: Current passing through the heater
Heat produced
Heat produced
Since,
12. Two bulbs are marked
Show Answer
Answer:13. A toaster produces more heat than a light bulb when connected in a parallel to the
Show Answer
Answer: Since,14. An electric heater is used everyday for 120 minutes. The electricity bill for 30 days is 60 units. Calculate the power of the electric heater.
Show Answer
Answer: Energy consumed in 30 days
(i.e),
15. In a household 5 tubelights of
Show Answer
Answer: Energy consumed by five tubelights of
Energy consumed by an electric press of
Energy consumed in 1 day
16. A
(i) The energy consumed for 60 days and
(ii) Cost of electricity consumed at the rate of ’
Show Answer
Answer: (i) Energy consumed in days
(ii) Cost of electricity
17. What is meant by rating of electrical appliance?
Show Answer
Answer: It is the product of the voltage and the current which can flow through the device.18. What are the factors on which heat dissipated by a conductor depends?
Show Answer
Answer: Heat dissipated depends upon (i) the square of current through the conductor (ii) the resistance of the conductor and (iii) the time for which current is passed.19. Why is heat produced when a current is passed through a conductor?
Show Answer
Answer: During their motion the electrons collide with one another and hence lose some kinetic energy. This loss in kinetic energy is dissipated as heat across the conductor.20. Define one watt.
Show Answer
Answer: The power expended by a source is said to be I watt if one ampere of current flows through it under a potential difference of 1 volt.21. Why should the melting point of a fuse wire be low?
Show Answer
Answer: So that it melts and breaks the circuit as soon as the safe limit of current is exceeded.22. The filament of electric bulb is made of tungsten. Why?
Show Answer
Answer: Because it has a high melting point and a high resistivity and does not easily react with air.Long Answer Questions :
DIRECTIONS: Give answer in four to five sentences.
1. What are the safety measures to be taken while dealing with household electricity?
Show Answer
Answer: It goes beyond doubt that electricity plays a very important role in our lives. It is one of the most important and convenient sources of energy at homes and industries. However, we daily come across reports regarding hazards of electricity. Use of electricity can prove to be very dangerous if certain precautions and safety measures are not observed in the design of electrical devices and in handling them. The various safety measures to be taken are:
(i) Use wires of high quality, proper amperage and good insulating material.
(ii) Cover all naked wires and joints with insulating tape.
(iii) All connections at plugs, switches, sockets must be tight.
(iv) Replace any defective plugs, switches and sockets.
(v) Never touch any part of the circuit without putting on rubber shoes or rubber gloves.
(vi) Use fuse (now a days MCB) of proper rating and material. (vii) All electrical appliances must be properly earthed.
(viii) Connect switches and fuse to live wires.
(ix) Put out the main switch in case of short circuiting and fire.
(x) Do not use water as fire extinguishers in case of fire due to electricity.
2. (i) What do you understand by earthing?
(ii) What are the advantages of earthing in a household electric circuit?
(iii) Explain how it is done.
Show Answer
Answer: (i) By earthing we mean that the metallic body of an electric appliance is connected to thick, copper wire, which is buried deep in the earth and at its end is a copper plate surrounded by a mixture of charcoal and common salt.
(ii) It is a kind of safety device which saves us from an electric shock, in case when the metal casing of the appliance happens to touch the live wire or due to short circuiting or leakage of electric current.
Whenever an appliance which is earthed, gets short circuited, the current from the metal casing of the appliance flows into the earth which acts as an electric sink, i.e., its potential always remains zero. Due to the flow of heavy current the fuse in that circuit melts and disconnects the appliance from the circuit. So the user who happens to touch the appliance is protected from receiving any electric shock. Another advantage is that due to overheating the house wiring system is saved from being damaged and same time from being burnt out.
(iii) A three core, cord having three wires coated with insulation of red, brown and green colour, in used for connecting the appliance to the mains for drawing current from the mains. At one end of the cord, red is connected to the pin marked L (live), the brown to the pin marked
3. Three 250 watt heaters are connected in parallel to a 100 volt supply. Calculate :
(i) the total current taken from the supply,
(ii) the resistance of each heater.
(iii) the energy supplied in
Show Answer
Answer: Given, power of one heater (P) = 250 watt, potential (V) = 100 volt, time (t) = 5 hours.
The three 250 watt heaters are connected in parallel then total current,
= 2.5 + 2.5 + 2.5 = 7.5 A
(ii) The resistance of each heater is
(iii) The energy supplied to the first heater is
Then three heaters in connection = 3
= 3.75 kWh energy is used in the circuit.
4. A heating coil is immersed in a calorimeter of heat capacity
Find (i) the resistance of the coil (ii) the potential difference across the coil. State the assumption used in your calculations.
Show Answer
Answer: (i) 2.085. State Joule’s law. Explain an experimental method to determine the value of
Show Answer
Answer: The same work done in different forms produces the same quantity of heat in all cases.
W = JQ
6. Sate Farady’s first law and second law of electrolysis and verify it experimentally.
Show Answer
Answer: 1st Law The mass of the substance deposited at the cathode is directly proportional to the quantity of electricity.
2nd Law
The same quantity of electricity passes through different electrolytes, the masses of ions liberated at the respective electrodes are proportional to the chemical equivalents.
7. Explain the working of the following
(i) Filament lamp
(ii) Flourescent lamp
(iii) Discharge lamp
(iv) Electric are lamp
(v) Drycells
Show Answer
Answer: (i) Filament offers high resistance to electric current.
Electric energy is converted into light and heat energy.
(ii) Discharge lamp is coated with fluorescent material from inside.
High voltages are applied to coils, heat them which results in emission of electrons. Collision of electrons
(iii) Glass mercury vapours produces lights.
high voltage is two electrodes fused to its. When ionized. Accelerated ions collide with neutral gas atoms that produce glow.
(iv) Produces high intensity light.
Carbon rods having pointed tips at one end and separated by small gap.
When
(v) Form of a primary cell.
Consists of zinc container (cathode) at the centre of
which a carbon rod (anode) is fixed. Dry paste of
Electrolyte is mixed with powdered coke.
Here, chemical energy is converted in electrical energy.
2 EXERCISE
Multiple Choice Questions:
DIRECTIONS : This section contains multiple choice questions. Each question has 4 choices (a), (b), (c) and (d) out of which ONLY ONE is correct.
1. Two electric lamps each of 100 watts
(a) 100 watts
(b) 200 watts
(c) 25 watts
(d) 50 watts
Show Answer
Answer: (d)2. An electric bulb is rated
(a)
(b)
(c)
(d)
Show Answer
Answer: (d)3. If it takes 8 minutes to boil a quantity of water electrically, how long will it take to boil the same quantity of water using the same heating coil but with the current doubled
(a) 32 minutes
(b) 16 minutes
(c) 4 minutes
(d) 2 minutes
Show Answer
Answer: (d)4. Kilowatt-hour is the unit of
(a) potential difference
(b) electric power
(c) electrical energy
(d) charge
Show Answer
Answer: (c)5. An electric bulb is filled with
(a) hydrogen
(b) oxygen and hydrogen
(c) ammonia
(d) nitrogen and argon
Show Answer
Answer: (d)6. When current is passed thought and electric bulb, its filament glows, but the wire leading current to the bulb does not glow because
(a) less current flows in the leading wire as compared to that in the filament
(b) the leading wire has more resistance than the filament
(c) the leading wire has less resistance than the filament
(d) filament has coating of fluorescent material over it
Show Answer
Answer: (c)7. From a power station, the power is transmitted at a very high voltage because -
(a) it is generated only at high voltage
(b) it is cheaper to produce electricity at high voltage
(c) electricity at high voltage is less dangerous
(d) there is less loss of energy in transmission at high voltage
Show Answer
Answer: (b)8. When a fuse is rated
(a) it will not work if current is less than 8A
(b) it has a resistance of 8 ohm
(c) it will work only if current is 8A
(d) it will burn if current exceeds 8A
Show Answer
Answer: (a)9. Fuse wire is made of
(a) platinum
(c) aluminium
(b) copper
(d) alloy in tin and lead
Show Answer
Answer: (c)10. Which is not a device based on the heating effect of electricity
(a) heater
(b) toaster
(c) refrigerator
(d) press
Show Answer
Answer: (b)11. Which of the following terms does not represent electrical power in a circuit?
(a)
(b)
(c)
(d)
Show Answer
Answer: (d)12. Two conducting wires of the same material and of equal lengths and equal diameters are first connected in series and then parallel in a circuit across the same potential difference. The ratio of heat produced in series and parallel combinations would be
(a)
(b)
(c)
(d)
Show Answer
Answer: (d)13. On which one of the following the emf of a cell does not depend
(a) The nature of the metal of electrodes
(b) The size of the plates
(c) Nature of the electrolyte
(d) The nature of electrodes
Show Answer
Answer: (b)14. The primary cell which is used in daily life is -
(a) Leclanche cell
(b) Dry cell
(c) Daniel cell
(d) Simple voitaic cell
Show Answer
Answer: (c)15. Which one of the following primary cells has emf 1.08 volts and which remains fairly constant?
(a) Daniel cell
(b) Simple voltaic cell
(c) Leclanche cell
(d) Dry cell
Show Answer
Answer: (d)16. Primary cell are connected in parallel to
(a) Increase voltage
(b) decrease capacity
(c) decrease internal resistance
(d) make electric current constant
Show Answer
Answer: (d)17. In a closed circuit drawing current from cell, the emf of a cell is always
(a) Less than potential difference
(b) More than potential difference
(c) Half of the potential difference
(d) Double of the potential difference
Show Answer
Answer: (b)18. The filament of an electric bulb is of tungsten because
(a) Its resistance is negligible
(b) It is cheaper
(c) Its melting point is high
(d) Filament is easily made
Show Answer
Answer: (c)19. When the current passes through the filament, it gets heated to incandescence and give light while the connecting wires are not heated because
(a) The connecting wires are good conductor of heat while the filament is bad conductor
(b) The connecting wires are of low resistance while the filament is of high resistance
(c) The density of connecting wires is less than that of the filament
(d) The connecting wires are bad conductor of heat while the filament is good conductor
Show Answer
Answer: (d)20. The bulbs which emit a bluish light, are
(a) Filled with argon
(b) Filled with nitrogen
(c) vacuum bulbs
(d) coated from inside with a light blue colour
Show Answer
Answer: (a)21. Which one of the following heater element is used in electric press
(a) copper wire
(b) nichrome wire
(c) lead wire
(d) iron wire
Show Answer
Answer: (a)22. If 10 cells each of 1.4 volts are connected in parallel, their equivalent potential difference will be
(a) 1.4 volts
(b) 0.14 volts
(c) 14 volts
(d) 10/1.4 volts
Show Answer
Answer: (b)23. What should be the characteristic of fuse wire?
(a) High melting point, high specific resistance
(b) Low melting point, low specific resistance
(c) High melting point, low specific resistance
(d) Low melting point, high specific resistance
Show Answer
Answer: (d) Fuse wire should be such that it melts immediatley when strong current flows through the circuit. The same is possible if its melting point is low and resistivity is high.24. The heating element of an electric heater should be made with a material, which should have
(a) high specific resistance and high melting point
(b) high specific resistance and low melting point
(c) low specific resistance and low melting point
(d) low specific resistance and high melting point
Show Answer
Answer: (a) A heating wire should be such that it produces more heat when current is passed through it and also does not melt. It will be so if it has high specific resistance and high melting point.25. Resistance of conductor is doubled keeping the potential difference across it constant. The rate of generation of heat will
(a) become one fourth
(b) be halved
(c) be doubled
(d) become four times
Show Answer
Answer: (b) The rate of generation of heat, for a given potential difference is,26. A current I passes through a wire of length
(a)
(b)
(c)
(d) none of these
Show Answer
Answer: (b) The rate of heat generation27. The resistance
(a)
(b)
(c)
(d)
Show Answer
Answer: (a) Heat produced,28. Two electric bulbs rated
(a)
(b)
(c)
(d)
Show Answer
Answer: (a) In parallel combination, total power29. First electro chemical cell was designed by
(a) Leclanche
(b) Faraday
(c) Galvanic
(d) Some one other than those mentioned above
Show Answer
Answer: (d) First electro-chemical cell was designed by Allexandro de-Volta.30. The electric cell is a device to obtain
(a) electric charge
(b) electric force
(c) electrons
(d) electric energy from chemical energy
Show Answer
Answer: (d)31. In which of the following cells polarisation is the major defect?
(a) Voltaic cell
(c) Leclanche cell
(b) Daniel cell
(d) Fuse cell
Show Answer
Answer: (a)32. Which of the following is NOT the name of a secondary cell?
(a) Storage cell
(b) Fuel cell
(c) Alkali cell
(d) Acid cell
Show Answer
Answer: (b)33. The current capacity of the charged secondary cell does not depend on
(a) rate of charging
(b) rate of discharging
(c) temperature
(d) amount of active material
Show Answer
Answer: (c)34. Which of the following is not the storage cell?
(a) Acid cell
(b) NIFE cell
(c) Edison cell
(d) Dry cell
Show Answer
Answer: (d) Dry cell being primary cell, cannot be recharged.35. In charging a battery of motor car, the following effect of electric current is used
(a) magnetic
(b) heating
(c) chemical
(d) induction
Show Answer
Answer: (c) Chemical effect of current is used in charging a car battery.36. Same current is being passed through a copper voltameter and silver voltameter. The rate of increase in weight of the cathodes in the two voltameters will be proportional to
(a) relative densities
(b) atomic masses
(c) atomic numbers
(d) none of the above
Show Answer
Answer: (d) The masses of the copper and silver deposited on cathodes of copper voltameter and silver voltameter are in the ratio of their chemical equivalent of copper and silver respectively.37. If
(a) farad
(b) newton
(c) coulomb
(d) faraday
Show Answer
Answer: (d)38. A silver and a zinc voltameter are connected in series and a current
(a) 1.7 W grams
(b) 2.4 W grams
(c) 3.5 W grams
(d) 1.2 W grams
Show Answer
Answer: (c)
or
39. What determines the e.m.f. between the two metals placed in an electrolyte?
(a) relative position of metals in the electro chemical series
(b) distance between them
(c) strength of electrolyte
(d) nature of electrolyte
Show Answer
Answer: (d)40. For electroplating a spoon, it is placed in the voltameter at
(a) the position of anode
(b) the position of cathode
(c) exactly in the middle of anode and cathode
(d) anywhere in the electrolyte
Show Answer
Answer: (b) In electroplating, the metallic ions are positive, w/hich are deposited on cathode.41. Electroplating does not help in
(a) fine finish to the surface
(b) shining appearance
(c) metals to become hard
(d) protect metals against corrosion
Show Answer
Answer: (c) Electroplating does not help in making the metals become hard.42. Faraday’s laws are consequence of conservation of
(a) energy
(b) energy and magnetic field
(c) charge
(d) magnetic field
Show Answer
Answer: (a) Faraday’s laws are based on the conversion of electrical energy into mechanical energy; which is in accordance with the law of conservation of energy.43. According to Faraday’s law of electrolysis, the amount of decomposition is proportional to
(a) 1/time for which current passes
(b) electrochemical equivalent of the substance
(c) 1/current
(d) 1/electrochemical equivalent
Show Answer
Answer: (b) The amount of decomposition (i.e., mass of the substance liberated during electrolysis) is proportional to electro chemical equivalent of the substance.44. The current inside a copper voltameter
(a) is half the outside value
(b) is the same as the outside value
(c) is twice the outside value
(d) depends on the concentration of
Show Answer
Answer: (b) Current inside a copper voltameter is same as that of outside.45. In electrolysis, the amount of mass deposited or liberated at an electrode is directly proportional to
(a) amount of charge
(b) square of current
(c) concentration of electrolyte
(d) square of electric charge
Show Answer
Answer: (a)46. Of the following, the one that does not make use of chemical effect of electric current is
(a) electroplating
(b) electrotyping
(c) thermoelectric refrigerator
(d) voltaic cells
Show Answer
Answer: (c)More Than One Correct
DIRECTIONS: This section contains multiple choice questions, Each question has 4 choices (a), (b), (c) and (d) out of which ONE OR MORE may be correct.
1. A constant voltage is applied between the two ends of a uniform metallic wire. If both the length and radius of wire are doubled, then:
(a) the heat developed in the wire will be doubled
(b) the electric field in the wire will be doubled
(c) the heat developed will remain the same
(d) the electric field in the wire will be halved
Show Answer
Answer: (a,d)2. The emf of a cell is:
(a) the potential difference across its terminals
(b) the potential difference across its terminals when no current is passing through it
(c) the heat produced when the cell is connected across a one ohm resistance
(d) the total work done per coulomb of electricity taken in a circuit in which the cell is connected
Show Answer
Answer: (b,d)3. In the circuit shown in the figure:
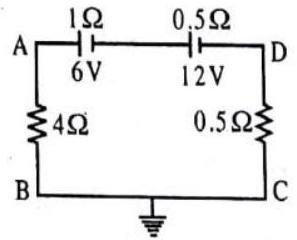
(a) the voltage at the point
(b) the voltage at the point
(c) if a voltmeter is connected across the
(d) if the voltmeter is connected across a
Show Answer
Answer: (b,c)4. When the terminals of a cell of e.m.f.
Which of the following statements is/are correct?
(a) The cell is non-ideal
(b) If a
(c) If a
(d) If a voltmeter of resistance
Show Answer
Answer: (a,c,d)
Potential difference across the accumulator
5. Which of the following statements is/are correct?
(a) Potential difference between terminals of a non-ideal battery can never be greater than its e.m.f.
(b) If a non-ideal battery is short circuited by a wire, heat generater in the wire is less than electric energy developed in the battery.
(c) e.m.f. of an ideal battery is first measured by a potentiometer and then by a voltmeter. Both the measurements are equally correct.
(d) Ncne of the above.
Show Answer
Answer: (a, b, c, d)
In case of non-ideal battery, an amount of energy is dissipated in the form of heat due to its internal resistance. If the battery is ideal then there is no internal drop hence reading of potentiometer and voltmeter will be equally correct.
6. Two cells of unequal e.m.fs.
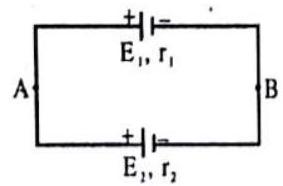
(a) one cell will continuously supply energy to the other
(b) the potential difference across both the cells will be equal
(c) the potential difference across one cell will be greater than its e.m.f.
(d)
Show Answer
Answer: (a, b, c)
Let
Current in the circuit
Hence,
Current flows in
7. Which of the following statement(s) is/are correct?
(a) If
(b) If
(c) If
(d) None of the above.
Show Answer
Answer: (a, b, d)
In series,
In parallel,
After shorting by a wire having a constant resistance
8. For the battery shown in the figure:
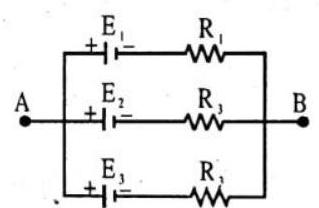
(a) equivalent internal resistance
(b) if
(c) equivalent e.m.f. of the battery is equal to
(d) equivalent e.m.f. of the battery not only depends upon values of
Show Answer
Answer:
Equivalent e.m.f. of the battery is obtained by applying Kirchhoff’s 2nd law to the two loops.
9. A cell of e.m.f.
(a) a single resistor of
(b) two
(c) two
(d) two
Show Answer
Answer: (a,d)10. Two heaters designed for the same voltage
(a) if they are in series,
(b) if they are in series,
(c) if they are in parallel,
(d) if they are in parallel,
Show Answer
Answer: (a,c)
Let
When used in series,
When used in parallel,
11. In a household electric circuit:
(a) all electric appliances drawing power are joined in parallel
(b) a switch may be either in series or in parallel with the appliance which it controls
(c) if a switch is in parallel with an appliance, it will draw power when the switch in the off position (open)
(d) if switch is in parallel with an appliance, the fuse will blow (burn out) when the switch is put on (closed)
Show Answer
Answer: (a, c, d)12. A cell drives a current through a circuit. The e.m.f. of the cell is equal to the work done in moving unit charge:
(a) from the positive to negative plate of the cell
(b) from the positive plate back to the positive plate
(c) from the negative plate back to the negative plate
(d) from any point in the circuit back to the same point
Show Answer
Answer: (b, c, d)13. A cell of e.m.f.
(a) the cell supplies
(b) heat is produced in
(c) heat is produced in
(d) heat is produced in the cell at the rate
Show Answer
Answer: (a,c,d)14. In the circuit shown in the fiugre:
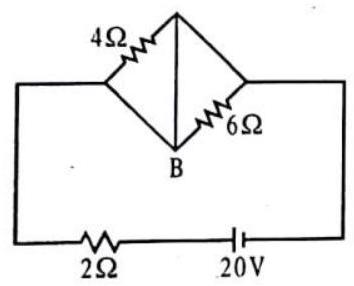
(a) power supplied by the battery is 200 watt
(b) current flowing in the circuit is
(c) potential difference across
(d) current in wire
Show Answer
Answer: (a, c)
This is also the current passing in wire
Power supplied by the battery.
Potential difference across
Fill in the Passage:
DIRECTIONS : Complete the following passage(s) with an appropriate word/term to be filled in the blank spaces.
melt
zero
Different electric circuits can withstand certain maximum amount of current without getting heated up and damaging themselves. For example, for a lighting circuit this limit is
Fuse should be connected in ………3………. of circuit. If it is connected to neutral wire, the fuse will melt when current ………4………. its rating, but the appliance will still be connected to high potential of supply through live wire. If a person touches the appliance, his body provides a path for current to flow to earth which is at ………5………. potential. Thus, person may get severe shock which may prove fatal. Hence, fuse is connected to live wire so that if it melts, the appliance is no longer connected to the high potential of the supply and possibility of electric shock is ………6……….
Show Answer
Answer: 1. melt
2. fuse
3. live wire
4. exceeds
5. zero
- eliminated
insulation
conducting
When the ………1………. of live wire in a circuit inside a electric appliance melts due to some reasons, the live wire may touch the metal casing of the appliance. Thus, metal casing is connected to high potential. This metal casing of appliances such as mixer, fan, refrigerator, toaster etc., is continuously ………2………. by us by our bare hands. Hence, we may get a severe shock as current will flow through our ………3………. to ………4………. which is considered as ………5………. potential. To prevent this, metal casings of all appliances are connected to earth by a ………6………. wire. This is called earthing.
Thus, ………7………. involves connecting metal body of electric appliance to thick copper wire which is connected to a copper plate buried deep in the earth. The copper plate is surrounded by a mixuture of charcoal and common salt.
Show Answer
Answer:
1. insulation
-
touched
-
body
-
earth
-
zero
-
conducting
-
earthing
conductor
lower potential
temperature
When a potential difference is applied between the ends of a ………1………, current begins to flow through it. The current flows as conductor contains large number of ………2……… which begin to drift from the end at ………3……… to the end at ………4……… In the process they constantly keep on ………5……… with atoms of conductor. The atoms gain energy and beging to ………6……… more vigorously about their mean position. The average ………7……… of atoms of conductor increases.
The results in a rise in the ………8……… of the conductor and we say that the conductor has been heated. Thus, flow of current has a heating effect on the conductor.
Show Answer
Answer:
1. conductor
-
free electrons
-
lower potential
-
higher potential
-
colliding
-
vibrate
-
kinetic energy
8 . temperature.
Passage Based Questions:
DIRECTIONS : Study the given paragraph(s) and answer the following questions.
Passage-I
Two tungston lamps with resistances
1. Which lamp will glow more brightly when they are connected in parallel?
(a) Bulb having lower resistance
(b) Bulb having higher resistance
(c) Both the bulbs
(d) None of the two bulbs
Show Answer
Answer: (a) When the lamps are connected in parallel, then potential difference
Hence, illumination produced by 2 nd bulb will be higher than produced by lst bulb, i.e., bulb having lower resistance will shine more brightly.
2. If the lamp of resistance
(a) Net illumination will increase
(b) Net illumination will decrease
(c) Net illumination will remain same
(d) Net illumination will reduced to zero
Show Answer
Answer: (b) When
i.e., net power consumed initially. In other words, net illumination will now decrease.
3. Which lamp will glow more brightly when they are connected in series?
(a) Bulb having lower resistance
(b) Bulb having higher resistance
(c) Both the bulbs
(d) None of the two bulbs
Show Answer
Answer: (b) When two lamps are connected in series, the potential difference across each lamp will be different but current
Hence.
i.e., illumination produced by Ist lamp will be higher as compared to that produced by 2nd lamp, i.e.. lamp having higher resistance will glow more brightly.
4. If the lampe of resistance
(a) Illumination will remain same
(b) Illumination will increase
(c) Illumination will increase
(d) Illumination will decrease
Show Answer
Answer: (b) When lamp of resistance
or
If
When both the lamps were present then total power consumed was given by:
i.e., illumination gets increased when only one bulb is used.
5. Would physically bending a supply wire cause any change in the illumination?
(a) Illumination will remain same
(b) Illumination will increase
(c) Illumination will decrease
(d) It is not possible to predict from the given data
Show Answer
Answer: (a) If a water pipe is given bend at some points, then it definitely reduces the flow of water in the pipe but this is not true in case of an electric current flowing in a conductor because electric current is established in a conductor due to drift motion of electrons in it along the line of the potential gradient. Hence, illumination is not affected due to bending along the length of supply wires.Passage-II
Figure shows the circuit of a potentiometer. The length of the potentiometer wire
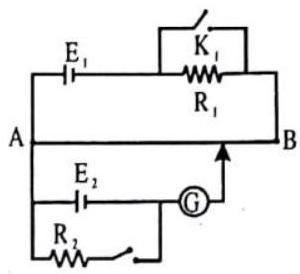
1. The emf of the cell
(a) 1 volt
(b) 2 volt
(c) 3 volt
(d) 4 volt
Show Answer
Answer: (a) Potential gradient.
2. The internal resistance of the cell
(a)
(b)
(c)
(d)
Show Answer
Answer: (d) When both the keys are closed: In the situation.
3. The balance length when key
(a) 10.5 cm
(b) 11.5 cm
(c) 12.5 cm
(d) 13.5 cm
Show Answer
Answer: (c) When key
i.e.,
4. The blance length when key
(a) 10.5 cm
(b) 11.5 cm
(c) 12.5 cm
(d) 13.5 cm
Show Answer
Answer: (c) When key
i.e.,
or
or
Passage-III
A 12 volt battery is connected to two light bulbs, as shown in figure. Light bulb 1 has resistance 3 ohm, while light bulb 2 has resistance 6 ohm. The battery has essentially no internal resistance and all the wires are essentially resistanceless too. When a light bulb is unscrewed, no current flows through that branch of the circuit. For instance, if light bulb 2 is unscrewed, current flows only around the lower loop of the circuit, which consists of the battery and light bulb 1 . When two resistances are joined in series, their equivalent resistances

1. When bulb 1 is screwed in, but bulb 2 is unscrewed, the power generated in bulb 1 is:
(a) 4 watt
(b) 12 watt
(c) 36 watt
(d) 48 watt
Show Answer
Answer: (d) Since, the power generated in the bulb equals the voltage across the bulb times the current through the bulb
flows through that bulb. Therefore, the bulb generates power,
2. Which of the following statements is false?
(a) Some of the energy produced by the light bulb takes the form of heat
(b) The battery is the source of all the electrons flowing around the circuit
(c) The current entering the light bulb equals the current leaving the light bulb
(d) The potential in the wire to the left of the light bulb differs from the potential in the wire to the right of that bulb
Show Answer
Answer: (b) Most of the charges flowing around the circuit are valence electrons stripped off the metal atoms in the wire and light bulbs. A battery does not supply all of the charges. It merely pushes around charges already present in the circuit.
Statements (c) and (d) are both true. All charges flowing into the light bulb also flow back out; no curent gest used up. But inside the bulb, those charges lose energy. This lost electrical energy converts into light and heat. So, the current has lower potential after flowing through the bulb.
3. Bulb 2 is now screwed in, as a result, bulb 1
(a) turns off
(b) becomes dimmer
(c) stays about the same brightness
(d) becomes brighter
Show Answer
Answer: (c) If the battery has internal resistance, bulb 1 would dim. But here, screwing in bulb 2 does not prevent bulb 1 from feeling the full 12 volts produced by the battery. Both bulbs get a full does of that voltage. So, the bulb 2 turns on without lessening the brightness ofbulb 1. If you picked (b), you probably thought that the battery can supply only a certain amount of current. According to this reasoning, bulb 2 draws current at the expense of bulb 1 . But the battery does not supply all of the electrons flowing around the circuit. It merely pushes around electrons already present in the metal wires and bulb filaments, as mentioned in earlier question. Screwing in bulb 2 allows the valence electrons in that branch of the circuit to join the current.4. When both light bulbs are screwed in, the current through the battery is
(a) 1.2 ampere
(b) 2 ampere
(c) 4 ampere
(d) 6 ampere
Show Answer
Answer: (d) The total current through the battery is simply,
where,
and hence,
So,
Intuitively, the equivalent resistance of two in-parallel resistors is less than either individual resistance because the current can split itself between two paths.
5. With only light bulb 1 screwed in, a never quit 12 volt battery goes dead in 24 days. With both light bulbs screwed in a never quit 12 volt battery goes dead in:
(a) 12 days
(b) 14 days
(c) 16 days
(d) 18 days
Show Answer
Answer: (c)Assertion & Reason :
DIRECTIONS : Each of these questions contains an Assertion followed by reason. Read them carefully and answer the question on the basis of following options. You have to select the one that best describes the two statements.
(a) If both Assertion and Reason are correct and Reason is the correct explanation of Assertion.
(b) If both Assertion and Reason are correct, but Reason is not the correct explanation of Assertion.
(c) If Assertion is correct but Reason is incorrect.
(d) If Assertion is incorrect but Reason is correct.
1. Assertion : When current through a bulb decreases by
Reason : Glow (Power) which is directly proportional to square of current.
Show Answer
Answer: (b)
2. Assertion : Long distance power transmission is done at high voltage.
Reason : At high voltage supply power losses are less.
Show Answer
Answer: (b) Power loss
3. Assertion : Resistance of
Reason : Resistance of bulb is inversely proportional to rated power.
Show Answer
Answer: (b)4. Assertion :
Reason : Light produced is same from same power.
Show Answer
Answer: (d) In tube light majority portion of radiation comes under visible region while bulb radiation consists of visible, ultraviolet, infrared radiation giving less visible part.5. Assertion : A resistor of resistance
Reason : The power dissipated in the circuit is directly proportional to the resistance of the circuit.
Show Answer
Answer: (c) Here,6. Assertion : A voltmeter and ammeter can be used together to measure resistance and power.
Reason : Resistance and power both are proportional to voltage and current.
Show Answer
Answer: (c) As7. Assertion : A torch bulb give light if operated on
Reason : Heating effect is common to both
Show Answer
Answer: (a)8. Assertion : A tube light emits white light.
Reason : Emission of light in a tube takes place at a very high temperature.
Multiple Matching Questions :
DIRECTIONS : Following question has four statements (A, B, C and D) given in Column I and four statements (p, q, r and s) in Column II. Any given Assertion Column I can have correct matching with one or more statement(s) given in Column II. Match the entries in column I with entries in column II.
1. For the circuit shown in the adjoining figure, match the entries of column I with the entries of column II.
| Column I | Column II | ||
|---|---|---|---|
| (a) | (p) | Current drawn from the battery is maximum | |
| (b) | (q) | Current drawn from the battery is the least | |
| (c) | (r) | Bulbs will lit the brightest | |
| (d) | (s) | Bulbs will lit with brightness lying between maximum and minimum value |
Show Answer
Answer: (A)2. In the shown circuit diagram, all the electric bulbs are identical. Then, match the entries of column I with the entries of column II
| Column I | Column II | ||
|---|---|---|---|
| (A) | Current drawn by |
(p) | maximum |
| (B) | Intensity of |
(q) | minimum |
| (C) | Intensity of |
(r) | same |
| (D) | Intensity of |
(s) | different |
Show Answer
Answer: (A)3. Six batteries of increasing e.m.f. and increasing internal resistance are connected in a circuit as shown in the given figure. Match the entries of column I with the entries of column II.
| Column I | Column II | |||
|---|---|---|---|---|
| (A) | Potential of point |
(p) | Zero | |
| (B) | Potential of point |
(q) | ||
| (C) | Potential of point |
(r) | ||
| (D) | Potential of point |
(s) | ||
| (t) | None |
Show Answer
Answer: (A)HOTS Subjective Questions :
DIRECTIONS : Answer the following questions.
1. How is a fuse put in an electric circuit? State the purpose of using a fuse in a circuit.
Show Answer
Answer: A fuse is safety device having a short length of a thin wire of tin-lead alloy, that melts and breaks the circuit if the current exceeds a safe value.2. A
Show Answer
Answer: The resistance of bulb and heater we respectively
current becomes
3. Explain why an electric bulb becomes dim when an electric heater in parallel circuit is made on. Why dimness decrease after sometime?
Show Answer
Answer: The resistance of a heater coil is less than that of electric bulb filament. When heater is switched on in parallel, more current start flowing through the heater coil and current through the bulb filament decreases making it dim.
After sometime, when heater coil becomes hot its resistance increases. As a result current through the heater coil decreases and the current through the bulb filament increases and thus dimness of the bulb decreases.
4. Why is tungsten metal selected for making filaments of incandescent lamp bulbs?
Show Answer
Answer: Tungsten metal has high resistivity and high melting point.5. A heater joined in parallel with a
Show Answer
Answer: Resistance of6. Nichrome and copper.wires of same length and same radius are connected in series. Current 1 is passed through then. Why does the nichrome wire get heated first?
Show Answer
Answer: Nichrome has greater resistivity.7. Two bulbs whose resistances are in the ratio
Show Answer
Answer: Since,8. In a factory, an electric bulb of 500 W is used for 2 hours and electric bulb of
Show Answer
Answer: Electrical energy consumed in 30 days
Cost of energy
9. An electric lamp is marked
(i) its resistance while glowing
(ii) energy consumed in
Show Answer
Answer: (i)
(ii) Energy consumed per day
10. A torch bulb is rated
Show Answer
Answer: Given :
From Ohm’s law
11. A heater coil is rated
Show Answer
Answer: Resistance of heater coil
Resistance of each cut part
When connected in parallel, the net resistance will be
Energy liberated per second
12. A
Show Answer
Answer: Power of lamp
Light liberated
13.
Refrigerator,
loudspeaker,
Choose only from the above electrical appliances those that make use of the
(a) chemical effect of an electric current.
(b) heating effect of an electric current.
(c) magnetic effect of an electric current.
Show Answer
Answer: (a) Battery charger, electroplating-vat
(b) electric chip-pan
(c) refrigerator, air conditioner, electric drill, loudspeaker
14. This calculation is aimed at finding out how many joules there are in 1 kilowatt hour :
1 watt second = 1 joule
1 kilowatt second = _______________joule
1 kilowatt minute = _______________
1 kilowatt hour = _______________
1 kilowatt hour = _______________joules
Show Answer
Answer: (a) 1 kilowatt second
1 kilowatt minute
1 kilowatt hour
15. A man sits in a room for an hour watching T.V. There are four
Number of kilowatt hours for
light bulbs = ___________________
T.V. = ___________________
air conditioner = ___________________
Total kilowatt hours = ___________________
Total cost is ___________________
Show Answer
Answer: Light bulbs = 1000
T.V. = 0.15 kWh
air conditioner = 0.45 kWh
Total = 1.0 kWh = 25e
16. What does it mean to say that your car has a 12-volt tautery?
Show Answer
Answer: It means that one of the battery terminals is17. A balloon may easily be charged to several thousind volts Does that mean it has several thousand joules of energy? Explain.
Show Answer
Answer: No, it doesn’t mean that the balloon has several thousand joules of energy always. It can have several thousand joules of energy only if the balloon acquires high charge.18. Calculate the cost of electric bill of a house for the month of March. The following appliances were used in the house for the duration shown respectively. The cost of electrical energy is 50 paise per unit.
Show Answer
Answer: Energy consumed by different appliances is
No. of units consumed per day
Total consumption for 31 days
Total cost of the bill










