Chapter 07 Motion Exercise
Exercises
1. An athlete completes one round of a circular track of diameter $200 m$ in $40 s$. What will be the distance covered and the displacement at the end of 2 minutes $20 s$ ?
Show Answer
Answer
Diameter of circular track (D) $=200 m$
Radius of circular track $(r)=200 / 2=100 m$
Time taken by the athlete for one round $(t)=40 s$
Distance covered by athlete in one round $(s)=2 \pi r$
$=2 \times(22 / 7) \times 100$
Speed of the athlete $(v)=$ Distance $/$ Time
$=(2 \times 2200) /(7 \times 40)$
$=4400 / 7 \times 40$
Therefore, Distance covered in $140 s=$ Speed $(s) \times$ Time $(t)$
$=4400 /(7 \times 40) \times(2 \times 60+20)$
$=4400 /(7 \times 40) \times 140$ $=4400 \times 140 / 7 \times 40$
$=2200 m$
Number of round in $40 s=1$ round
Number of round in $140 s=140 / 40$
$=3^{1 / 2}$
After taking start from position $X$, the athlete will be at postion $Y$ after $3 \frac{1}{2}$ rounds as shown in figure
Hence, Displacement of the athlete with respect to initial position at $x=$ xy $=$ Diameter of circular track
$=200 m$
2. Joseph jogs from one end A to the other end B of a straight $300 m$ road in 2 minutes 30 seconds and then turns around and jogs $100 m$ back to point $C$ in another 1 minute. What are Joseph’s average speeds and velocities in jogging (a) from $A$ to $B$ and (b) from $A$ to $C$ ?
Show Answer
Answer
Total Distance covered from $A B=300 m$
Total time taken $=2 \times 60+30 s$
$=150 s$
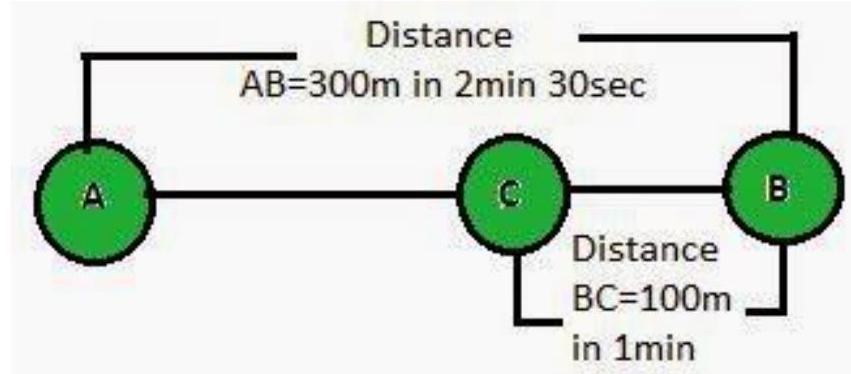
Therefore, Average Speed from AB = Total Distance / Total Time $=300 / 150 m s^{-1}$
$=2 m s^{-1}$
Therefore, Velocity from AB =Displacement AB $/$ Time $=300 / 150 m s^{-1}$
$=2 m s^{-1}$
Total Distance covered from $A C=A B+B C$
$=300+200 m$
Total time taken from A to C = Time taken for AB + Time taken for BC $=(2 \times 60+30)+60 s$
$=210 s$
Therefore, Average Speed from AC = Total Distance /Total Time
$=400 / 210 m s^{-1}$
$=1.904 m s^{-1}$
Displacement (S) from $A$ to $C=A B-B C$
$=300-100 m$
$=200 m$
Time (t) taken for displacement from AC $=210 s$
Therefore, Velocity from AC = Displacement (s) / Time (t)
$=200 / 210 m s^{-1}$
$=0.952 m s^{-1}$
3. Abdul, while driving to school, computes the average speed for his trip to be $20 km h^{-1}$. On his return trip along the same route, there is less traffic and the average speed is $30 km h^{-1}$. What is the average speed for Abdul’s trip?
Show Answer
Answer
The distance Abdul commutes while driving from Home to School $=S$ Let us assume time taken by Abdul to commutes this distance $=t_1$ Distance Abdul commutes while driving from School to Home $=S$ Let us assume time taken by Abdul to commutes this distance $=t_2$ Average speed from home to school $v _{1 av}=20 km h _{\text{- }}$ Average speed from school to home $v _{2 a v}=30 km h^{-1}$ Also we know Time taken form Home to School $t_1=S / v _{1 av}$ Similarly Time taken form School to Home $t_2=S / V _{2 a v}$ Total distance from home to school and backward $=2 S$ Total time taken from home to school and backward $(T)=S / 20+S / 30$ Therefore, Average speed $(V _{av})$ for covering total distance $(2 S)=$ Total Dostance/Total Time
$=2 S /(S / 20+S / 30)$
$=2 S /[(30 S+20 S) / 600]$
$=1200 S / 50 S$
$=24 kmh^{-1}$
4. A motorboat starting from rest on a lake accelerates in a straight line at a constant rate of $3.0 m s^{-2}$ for $8.0 s$. How far does the boat travel during this time?
Show Answer
Answer
Given Initial velocity of motorboat, $u=0$
Acceleration of motorboat, $a=3.0 m s^{-2}$
Time under consideration, $t=8.0 s$
We know that Distance, $s=u t+(1 / 2) a t^{2}$
Therefore, The distance travel by motorboat $=0 \times 8+(1 / 2) 3.0 \times 82$
$=(1 / 2) \times 3 \times 8 \times 8 m$
$=96 m$
5. A driver of a car travelling at $52 km h^{-1}$ applies the brakes Shade the area on the graph that represents the distance travelled by the car during the period. (b) Which part of the graph represents uniform motion of the car?
Show Answer
missing6. Fig 7.10 shows the distance-time graph of three objects A,B and $C$. Study the graph and answer the following questions:
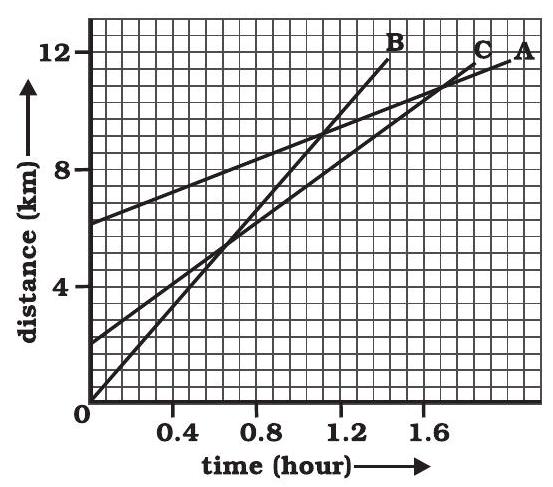
Fig. 7.10
(a) Which of the three is travelling the fastest?
(b) Are all three ever at the same point on the road?
(c) How far has C travelled when B passes A?
(d) How far has B travelled by the time it passes C?
Show Answer
Answer
(a) Object B
(b) No
(c) $5.71 km$
(d) $5.14 km$
(a) Speed $=\frac{\text{ Distance }}{\text{ Time }}$
Slope of graph $=\frac{y \text{-axis }}{x-\text{ axis }}=\frac{\text{ Distance }}{\text{ Time }}$
Therefore, Speed = slope of the graph
Since slope of object B is greater than objects A and C, it is travelling the fastest.
(b) All three objects A, B and C never meet at a single point. Thus, they were never at the same point on road.
(c)
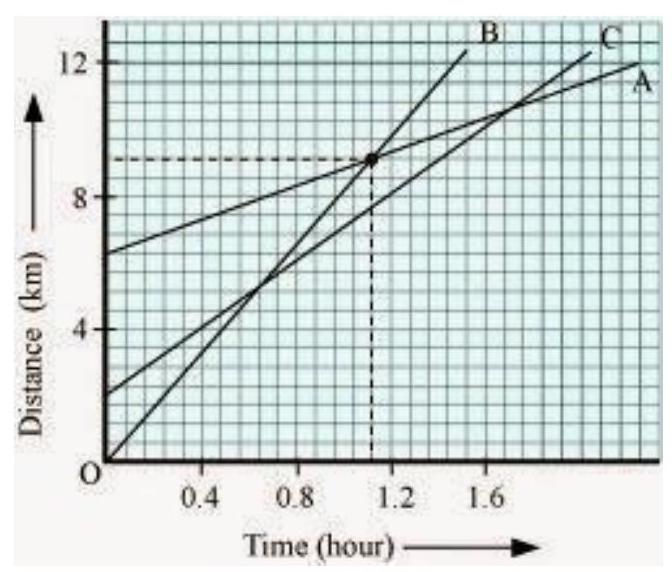
Since there are 7 unit areas of the graph between 0 and 4 on the Y axis, 1 graph unit equals $4/7 km$.
Since the initial point of an object C is 4 graph units away from the origin, Its initial distance from the origin is $4\times(4/7)km = 16/7 km$
When B passes A, the distance between the origin and C is $8km$
Therefore, total distance travelled by C in this time = $8 – (16/7) km = 5.71 km$
(d) The distance that object B has covered at the point where it passes C is equal to 9 graph units. Therefore, total distance travelled by B when it crosses C = $9\times(4/7) = 5.14 km$
7. A ball is gently dropped from a height of $20 m$. If its velocity increases uniformly at the rate of $10 m s^{-2}$, with what velocity will it strike the ground? After what time will it strike the ground?
Show Answer
Answer
Let us assume, the final velocity with which ball will strike the ground be ’ $v$ ’ and time it takes to strike the ground be ’ $t$ ’ Initial Velocity of ball, $u=0$
Distance or height of fall, $s=20 m$
Downward acceleration, $a=10 m s^{-2}$
As we know, $2 a s=v^{2}-u^{2}$
$v^{2}=2 as+u^{2}$
$=2 \times 10 \times 20+0$
$=400$
$\therefore$ Final velocity of ball, $v=20 ms^{-1}$
$t=(v-u) / a$
$\therefore$ Time taken by the ball to strike $=(20-0) / 10$
$=20 / 10$
$=2$ seconds
8. The speed-time graph for a car is shown is Fig. 7.11.
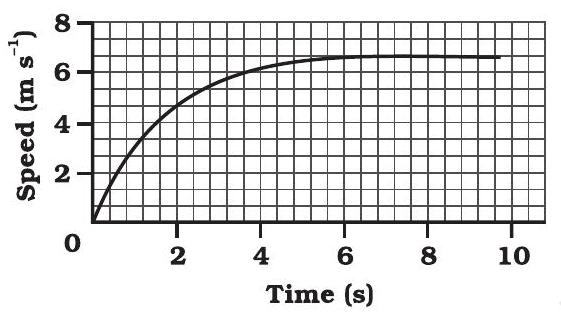
Fig. 7.11
(a) Find how far does the car travel in the first 4 seconds. Shade the area on the graph that represents the distance travelled by the car during the period.
(b) Which part of the graph represents uniform motion of the car?
Show Answer
Answer
(a)
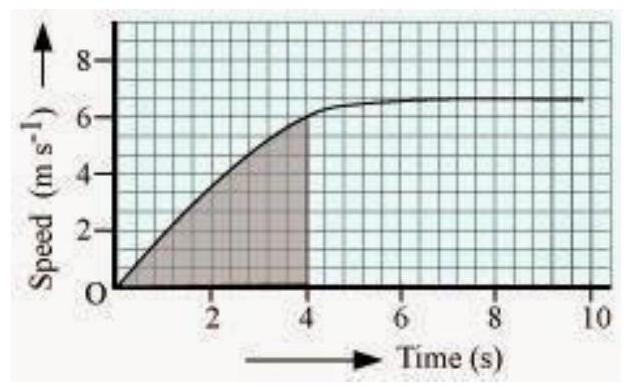
The shaded area which is equal to $1 / 2 \times 4 \times 6=12 m$ represents the distance travelled by the car in the first $4 s$.
(b)
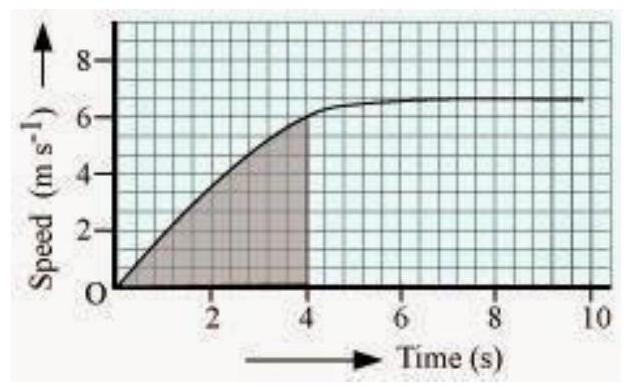
The part of the graph in red colour between time $6 s$ to $10 s$ represents uniform motion of the car.
9. State which of the following situations are possible and give an example for each of these:
(a) an object with a constant acceleration but with zero velocity
(b) an object moving with an acceleration but with uniform speed.
(c) an object moving in a certain direction with an acceleration in the perpendicular direction.
Show Answer
Answer
(a) It is possible; an object thrown up into the air has a constant acceleration due to gravity acting on it. However, when it reaches its maximum height, its velocity is zero.
(b) It is possible; acceleration implies an increase or decrease in speed, and uniform speed implies that the speed does not change over time
Circular motion is an example of an object moving with acceleration but with uniform speed.
An object moving in a circular path with uniform speed is still under acceleration because the velocity changes due to continuous changes in the direction of motion.
(c) It is possible; for an object accelerating in a circular trajectory, the acceleration is perpendicular to the direction followed by the object.
10. An artificial satellite is moving in a circular orbit of radius $42250 km$. Calculate its speed if it takes 24 hours to revolve around the earth.
Show Answer
Answer
Radius of the circular orbit, $r=42250 km$
Time taken to revolve around the earth, $t=24 h$
Speed of a circular moving object, $v=(2 \pi r) / t$
$=[2 \times(22 / 7) \times 42250 \times 1000] /(24 \times 60 \times 60)$
$=(2 \times 22 \times 42250 \times 1000) /(7 \times 24 \times 60 \times 60) m s^{-1}$
$=3073.74 m s^{-1}$
