Chapter 11 Surface Areas and Volumes Exercise-03
EXERCISE 11.3
Assume $\pi=\frac{22}{7}$, unless stated otherwise.
1. Find the volume of the right circular cone with
(i) radius $6 \mathrm{~cm}$, height $7 \mathrm{~cm}$
(ii) radius $3.5 \mathrm{~cm}$, height $12 \mathrm{~cm}$
Show Answer
Solution
(i) Radius ( $r$ ) of cone $=6 cm$
Height (h) of cone $=7 cm$
Volume of cone
$ =\frac{1}{3} \pi r^{2} h $
$=[\frac{1}{3} \times \frac{22}{7} \times(6)^{2} \times 7] cm^{3}$
$=(12 \times 22) cm^{3}$
$=264 cm^{3}$
Therefore, the volume of the cone is $264 cm^{3}$.
(ii) Radius ( $r$ ) of cone $=3.5 cm$
Height (h) of cone $=12 cm$
Volume of cone
$ =\frac{1}{3} \pi r^{2} h $
$=[\frac{1}{3} \times \frac{22}{7} \times(3.5)^{2} \times 12] cm^{3}$
$=(\frac{1}{3} \times 22 \times \frac{1}{2} \times 3.5 \times 12) cm^{3}$
$=154 cm^{3}$
Therefore, the volume of the cone is $154 cm^{3}$.
2. Find the capacity in litres of a conical vessel with
(i) radius $7 \mathrm{~cm}$, slant height $25 \mathrm{~cm}$
(ii) height $12 \mathrm{~cm}$, slant height $13 \mathrm{~cm}$
Show Answer
Solution
(i) Radius ( $r$ ) of cone $=7 cm$
Slant height (I) of cone $=25 cm$
Height (h) of cone $=\sqrt{l^{2}-r^{2}}$
$=(\sqrt{25^{2}-7^{2}}) cm$
$=24 cm$
Volume of cone $=\frac{1}{3} \pi r^{2} h$
$=(\frac{1}{3} \times \frac{22}{7} \times(7)^{2} \times 24) cm^{3}$
$=(154 \times 8) cm^{3}$
$=1232 cm^{3}$
Therefore, capacity of the conical vessel
$=(\frac{1232}{1000})$ litres $(1.$ litre $.=1000 cm^{3})$
$=1.232$ litres
(ii) Height (h) of cone $=12 cm$
Slant height $(I)$ of cone $=13 cm$
Radius $(r.$ ) of cone $=\sqrt{l^{2}-h^{2}}$
$=(\sqrt{13^{2}-12^{2}}) cm$
$=5 cm$
Volume of cone $=\frac{1}{3} \pi r^{2} h$
$ \begin{aligned} & =[\frac{1}{3} \times \frac{22}{7} \times(5)^{2} \times 12] cm^{3} \\ & =(4 \times \frac{22}{7} \times 25) cm^{3} \\ & =(\frac{2200}{7}) cm^{3} \end{aligned} $
Therefore, capacity of the conical vessel
$ \begin{aligned} &(\frac{2200}{7000}) \text{ litres }(1 \text{ litre }=1000 cm^{3}) \\ &= \frac{11}{35} \text{ litres } \\ & \text{ Question 3: } \end{aligned} $
3. The height of a cone is $15 \mathrm{~cm}$. If its volume is $1570 \mathrm{~cm}^{3}$, find the radius of the base. (Use $\pi=3.14$ )
Show Answer
Solution
Height $(h)$ of cone $=15 cm$
Let the radius of the cone be $r$. Volume of cone
$=1570 cm^{3}$
$\frac{1}{3} \pi r^{2} h=1570 cm^{3}$
$\Rightarrow(\frac{1}{3} \times 3.14 \times r^{2} \times 15) cm=1570 cm^{3}$
$\Rightarrow r^{2}=100 cm^{2}$
$\Rightarrow r=10 cm$
Therefore, the radius of the base of cone is $10 cm$.
4. If the volume of a right circular cone of height $9 \mathrm{~cm}$ is $48 \pi \mathrm{cm}^{3}$, find the diameter of its base.
Show Answer
Solution
Height (h) of cone $=9 cm$
Let the radius of the cone be $r$.
Volume of cone $=48 cm^{3}$
$\Rightarrow \frac{1}{3} \pi r^{2} h=48 \pi cm^{3}$
$\Rightarrow(\frac{1}{3} \pi r^{2} \times 9) cm=48 \pi cm^{3}$
$\Rightarrow r^{2}=16 cm^{2}$
$\Rightarrow r=4 cm$
Diameter of base $=2 r=8 cm$
5. A conical pit of top diameter $3.5 \mathrm{~m}$ is $12 \mathrm{~m}$ deep. What is its capacity in kilolitres?
Show Answer
Solution
Radius ( $r$ ) of pit
$ =(\frac{3.5}{2}) m=1.75 m $
Height $(h)$ of pit $=$ Depth of pit $=12 m$
Volume of pit
$ =\frac{1}{3} \pi r^{2} h $
$=[\frac{1}{3} \times \frac{22}{7} \times(1.75)^{2} \times 12] cm^{3}$
$=38.5 m^{3}$
Thus, capacity of the pit $=(38.5 \times 1)$ kilolitres $=38.5$ kilolitres
6. The volume of a right circular cone is $9856 \mathrm{~cm}^{3}$. If the diameter of the base is $28 \mathrm{~cm}$, find
(i) height of the cone
(ii) slant height of the cone
(iii) curved surface area of the cone
Show Answer
Solution
(i) Radius of cone $=(\frac{28}{2}) cm=14 cm$
Let the height of the cone be $h$. Volume of cone
$=9856 cm^{3}$
$ \begin{aligned} & \Rightarrow \frac{1}{3} \pi r^{2} h=9856 cm^{3} \\ & \Rightarrow[\frac{1}{3} \times \frac{22}{7} \times(14)^{2} \times h] cm^{2}=9856 cm^{3} \end{aligned} $
$h=48 cm$
Therefore, the height of the cone is $48 cm$.
(ii) Slant height (I) of cone $=\sqrt{r^{2}+h^{2}}$
$=[\sqrt{(14)^{2}+(48)^{2}}] cm$
$=[\sqrt{196+2304}] cm$
$=50 cm$
Therefore, the slant height of the cone is $50 cm$.
(iii) $CSA$ of cone $=nrl$
$=(\frac{22}{7} \times 14 \times 50) cm^{2}$
$=2200 cm^{2}$
Therefore, the curved surface area of the cone is $2200 cm^{2}$.
7. A right triangle $\mathrm{ABC}$ with sides $5 \mathrm{~cm}, 12 \mathrm{~cm}$ and $13 \mathrm{~cm}$ is revolved about the side $12 \mathrm{~cm}$. Find the volume of the solid so obtained.
Show Answer
Solution
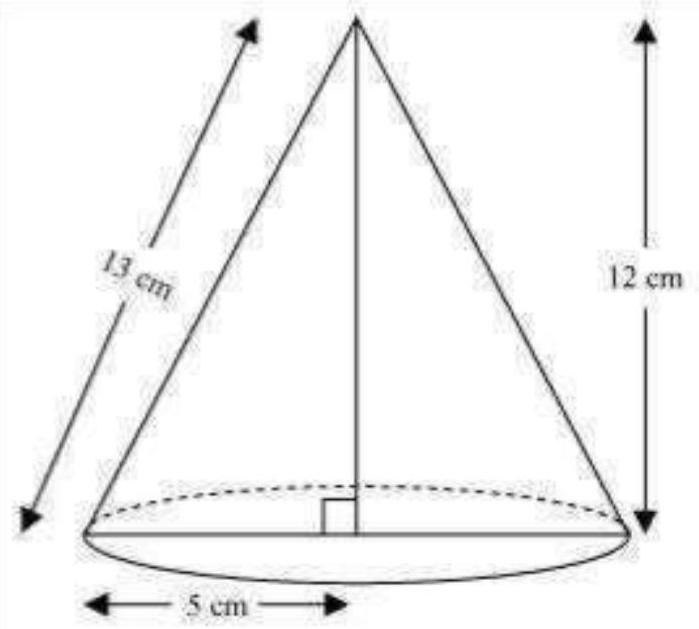
When right-angled $\triangle A B C$ is revolved about its side $12 cm$, a cone with height $(h)$ as 12 $cm$, radius ( $r$ ) as $5 cm$, and slant height (I) $13 cm$ will be formed.
$ =\frac{1}{3} \pi r^{2} h $
Volume of cone
$=[\frac{1}{3} \times \pi \times(5)^{2} \times 12] cm^{3}$
$=100 \pi cm^{3}$
Therefore, the volume of the cone so formed is $100 n cm^{3}$.
8. If the triangle $\mathrm{ABC}$ in the Question 7 above is revolved about the side $5 \mathrm{~cm}$, then find the volume of the solid so obtained. Find also the ratio of the volumes of the two solids obtained in Questions 7 and 8.
Show Answer
Solution
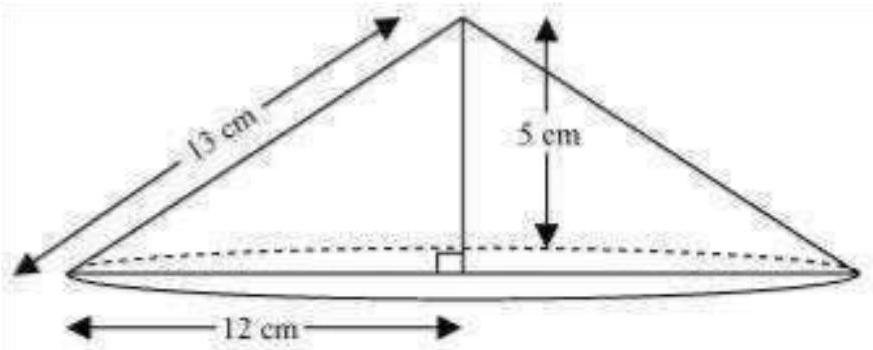
When right-angled $\triangle A B C$ is revolved about its side $5 cm$, a cone will be formed having radius ( $r$ ) as $12 cm$, height ( $h$ ) as $5 cm$, and slant height $(I)$ as $13 cm$.
$ \begin{aligned} & \qquad=\frac{1}{3} \pi r^{2} h \\ & \text{ Volume of cone } \\ & =[\frac{1}{3} \times \pi \times(12)^{2} \times 5] cm^{3} \\ & =240 \pi cm^{3} \\ & \qquad=\frac{100 \pi}{240 \pi} \\ & \text{ Reauired ratio } \\ & =\frac{5}{12}=5: 12 \end{aligned} $
9. A heap of wheat is in the form of a cone whose diameter is $10.5 \mathrm{~m}$ and height is $3 \mathrm{~m}$. Find its volume. The heap is to be covered by canvas to protect it from rain. Find the area of the canvas required.
Show Answer
Solution
Radius ( $r$ ) of heap
$ =(\frac{10.5}{2}) m=5.25 m $
Height ( $h$ ) of heap $=3 m$
Volume of heap
$ =\frac{1}{3} \pi r^{2} h $
$=(\frac{1}{3} \times \frac{22}{7} \times(5.25)^{2} \times 3) m^{3}$
$=86.625 m^{3}$
Therefore, the volume of the heap of wheat is $86.625 m^{3}$.
Area of canvas required $=$ CSA of cone
$ \begin{aligned} & =\pi r l=\pi r \sqrt{r^{2}+h^{2}} \\ & =[\frac{22}{7} \times 5.25 \times \sqrt{(5.25)^{2}+(3)^{2}}] m^{2} \\ & =(\frac{22}{7} \times 5.25 \times 6.05) m^{2} \\ & =99.825 m^{2} \end{aligned} $
Therefore, $99.825 m^{2}$ canvas will be required to protect the heap from rain.
Find the volume of a sphere whose radius is
(i) $7 cm$ (ii) $0.63 m$
Assume $.\pi=\frac{22}{7}]$










