Chapter 07 Comparing Quantities
7.1 PERCENTAGE - ANOTHER WAY OF COMPARING QUANTITIES
| Anita’s Report |
|---|
| Total $320 / 400$ |
| Percentage: 80 |
| Rita’s Report |
|---|
| Total $300 / 360$ |
| Percentage: 83.3 |
Anita said that she has done better as she got 320 marks whereas Rita got only 300. Do you agree with her? Who do you think has done better?
Mansi told them that they cannot decide who has done better by just comparing the total marks obtained because the maximum marks out of which they got the marks are not the same.
She said why don’t you see the Percentages given in your report cards?
Anita’s Percentage was 80 and Rita’s was 83.3. So, this shows Rita has done better. Do you agree?
Percentages are numerators of fractions with denominator 100 and have been used in comparing results. Let us try to understand in detail about it.
7.1.1 Meaning of Percentage
Per cent is derived from Latin word ‘per centum’ meaning ‘per hundred’.
Per cent is represented by the symbol $%$ and means hundredths too. That is $1 %$ means
1 out of hundred or one hundredth. It can be written as: $1 %=\frac{1}{100}=0.01$
To understand this, let us consider the following example.
Rina made a table top of 100 different coloured tiles. She counted yellow, green, red and blue tiles separately and filled the table below. Can you help her complete the table?
| Colour | Number of Tiles |
Rate per Hundred |
Fraction | Written as | Read as |
|---|---|---|---|---|---|
| Yellow | 14 | 14 | $\frac{14}{100}$ | $14 %$ | 14 per cent |
| Green | 26 | 26 | $\frac{26}{100}$ | $26 %$ | 26 per cent |
| Red | 35 | 35 | —– | —– | —— |
| Blue | 25 | —– | —– | —– | —– |
| Total | $\mathbf{1 0 0}$ |
TRY THESE
1. Find the Percentage of children of different heights for the following data.
| Height | Number of Children | In Fraction | In Percentage |
|---|---|---|---|
| $110 ~cm$ | 22 | ||
| $120 ~cm$ | 25 | ||
| $128 ~cm$ | 32 | ||
| $130 ~cm$ | 21 | ||
| Total | $\mathbf{1 0 0}$ |
2. A shop has the following number of shoe pairs of different sizes.
Size $2: 20$
Size $3: 30$
Size $4: 28$
Size $5: 14$
Size $6: 8$
Write this information in tabular form as done earlier and find the Percentage of each shoe size available in the shop.
Percentages when total is not hundred
In all these examples, the total number of items add up to 100. For example, Rina had 100 tiles in all, there were 100 children and 100 shoe pairs. How do we calculate Percentage of an item if the total number of items do not add up to 100 ? In such cases, we need to convert the fraction to an equivalent fraction with denominator 100. Consider the following example. You have a necklace with twenty beads in two colours.
| Colour | Number of Beads |
Fraction | Denominator Hundred | In Percentage |
|---|---|---|---|---|
| Red | 8 | $\frac{8}{20}$ | $\frac{8}{20} \times \frac{100}{100}=\frac{40}{100}$ | $40 %$ |
| Blue | 12 | $\frac{12}{20}$ | $\frac{12}{20} \times \frac{100}{100}=\frac{60}{100}$ | $60 %$ |
| Total | $2 0$ |
Anwar found the Percentage of red beads like this
Out of 20 beads, the number of red beads is 8 . Hence, out of 100 , the number of red beads is $\frac{8}{20} \times 100=40($ out of hundred) $=40$%
Asha does it like this
$ \frac{8}{20}=\frac{8 \times 5}{20 \times 5} $
$=\frac{40}{100}=40$%
We see that these three methods can be used to find the Percentage when the total does not add to give 100. In the method shown in the table, we multiply the fraction by $\frac{100}{100}$. This does not change the value of the fraction. Subsequently, only 100 remains in the denominator.
Anwar has used the unitary method. Asha has multiplied by $\frac{5}{5}$ to get 100 in the denominator. You can use whichever method you find suitable. May be, you can make your own method too.
The method used by Anwar can work for all ratios. Can the method used by Asha also work for all ratios? Anwar says Asha’s method can be used only if you can find a natural number which on multiplication with the denominator gives 100 . Since denominator was 20 , she could multiply it by 5 to get 100 . If the denominator was 6 , she would not have been able to use this method. Do you agree?
TRY THESE
1. A collection of 10 chips with different colours is given .
| Colour | Number | Fraction | Denominator Hundred | In Percentage |
|---|---|---|---|---|
| Green | ||||
| Blue | ||||
| Red | ||||
| Total |
Fill the table and find the percentage of chips of each colour.
2. Mala has a collection of bangles. She has 20 gold bangles and 10 silver bangles. What is the percentage of bangles of each type? Can you put it in the tabular form as done in the above example?
THINK, DISCUSS AND WRITE
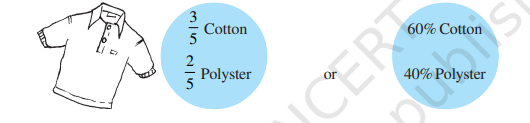
1. Look at the examples below and in each of them, discuss which is better for comparison.
In the atmosphere, $1 g$ of air contains:

2. A shirthas:
7.1.2 Converting Fractional Numbers to Percentage
Fractional numbers can have different denominator. To compare fractional numbers, we need a common denominator and we have seen that it is more convenient to compare if our denominator is 100 . That is, we are converting the fractions to Percentages. Let us try converting different fractional numbers to Percentages.
Example 1 Write $\frac{1}{3}$ as per cent.
Solution
We have, $\frac{1}{3}=\frac{1}{3} \times \frac{100}{100}=\frac{1}{3} \times 100 %$
$ =\frac{100}{3} %=33 \frac{1}{3} % $
Example 2 Out of 25 children in a class, 15 are girls. What is the percentage of girls?
Solution
Out of 25 children, there are 15 girls.
Therefore, percentage of girls $=\frac{15}{25} \times 100=60$. There are $60 %$ girls in the class.
Example 3 Convert $\frac{5}{4}$ to per cent.
Solution
We have, $\frac{5}{4}=\frac{5}{4} \times 100 %=125 %$
From these examples, we find that the percentages related to proper fractions are less than 100 whereas percentages related to improper fractions are more than 100.
THINK, DISCUSS AND WRITE
(i) Can you eat $50 %$ of a cake? Can you eat $100 %$ of a cake?
Can you eat $150 %$ of a cake?
(ii) Can a price of an item go up by $50 %$ ? Can a price of an item go up by $100 %$ ?
Can a price of an item go up by $150 %$ ?
7.1.3 Converting Decimals to Percentage
We have seen how fractions can be converted to per cents. Let us now find how decimals can be converted to per cents.
Example 4 Convert the given decimals to per cents:
(a) 0.75
(b) 0.09
(c) 0.2
Solution
(a) $0.75=0.75 \times 100 %$
(b) $0.09=\frac{9}{100}=9 %$
$ =\frac{75}{100} \times 100 %=75 % $
(c) $0.2=\frac{2}{10} \times 100 %=20 %$
TRY THESE
1. Convert the following to per cents:
(a) $\frac{12}{16}$
(b) 3.5
(c) $\frac{49}{50}$
(d) $\frac{2}{2}$
(e) 0.05
2. (i) Out of 32 students, 8 are absent. What per cent of the students are absent?
(ii) There are 25 radios, 16 of them are out of order. What per cent of radios are out of order?
(iii) A shop has 500 items, out of which 5 are defective. What per cent are defective?
(iv) There are 120 voters, 90 of them voted yes. What per cent voted yes?
7.1.4 Converting Percentages to Fractions or Decimals
We have so far converted fractions and decimals to percentages. We can also do the reverse. That is, given per cents, we can convert them to decimals or fractions. Look at the table, observe and complete it:
| Per cent | $1 $% | $10 $% | $25 $% | $50 $% | $90 $% | $125 $% | $250$% |
|---|---|---|---|---|---|---|---|
| Fraction | $\frac{1}{100}$ | $\frac{10}{100}=\frac{1}{10}$ | |||||
| Decimal | 0.01 | 0.10 |
Make some more such examples and solve them.
Parts always add to give a whole
In the examples for coloured tiles, for the heights of children and for gases in the air, we find that when we add the Percentages we get 100. All the parts that form the whole when added together gives the whole or $100 %$. So, if we are given one part, we can always find out the other part. Suppose,
$30 %$% of a given number of students are boys.
This means that if there were 100 students, 30 out of them would be boys and the remaining would be girls.
Then girls would obviously be $(100-30) %=70 %$.
TRY THESE
1. 35%+_____%=100%,
64%+20%+_____%=100%
45%=100%-_____%
70%=_____%-30%
2. If $65 %$ of students in a class have a bicycle, what per cent of the student do not have bicycles?
3. We have a basket full of apples, oranges and mangoes. If $50 %$ are apples, $30 %$ are oranges, then what per cent are mangoes?
THINK, DISCUSS AND WRITE
Consider the expenditure made on a dress
$20 %$% on embroidery, $50 %$% on cloth, $30 %$% on stitching.
Can you think of more such examples?
7.1.5 Fun with Estimation
Percentages help us to estimate the parts of an area.
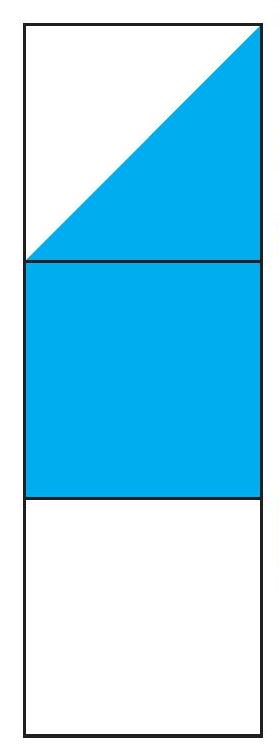
Example 5 What per cent of the adjoining figure is shaded?
Solution
We first find the fraction of the figure that is shaded. From this fraction, the percentage of the shaded part can be found.
You will find that half of the figure is shaded. And, $\frac{1}{2}=\frac{1}{2} \times 100 =50 $%
Thus, $50$% of the figure is shaded.
TRY THESE
What per cent of these figures are shaded?
You can make some more figures yourself and ask your friends to estimate the shaded parts.
7.2 USE OF PERCENTAGES
7.2.1 Interpreting Percentages
We saw how percentages were helpful in comparison. We have also learnt to convert fractional numbers and decimals to percentages. Now, we shall learn how percentages can be used in real life. For this, we start with interpreting the following statements:
—-$5 %$% of the income is saved by Ravi. —– $20 %$% of Meera’s dresses are blue in colour. —-Rekha gets $10 %$% on every book sold by her.
What can you infer from each of these statements?
By $5 %$% we mean 5 parts out of 100 or we write it as $\frac{5}{100}$. It means Ravi is saving ₹ 5 out of every ₹ 100 that he earns. In the same way, interpret the rest of the statements given above.
7.2.2 Converting Percentages to “How Many”
Consider the following examples:
Example 6 A survey of 40 children showed that $25 %$ liked playing football. How many children liked playing football?
Solution
Here, the total number of children are 40 . Out of these, $25 %$ like playing football. Meena and Arun used the following methods to find the number. You can choose either method.
Arun does it like this
Out of 100, 25 like playing football
So out of 40 , number of children who like
playing football $=\frac{25}{100} \times 40=10$
Meena does it like this
$25 %$ of $40=\frac{25}{100} \times 40$
$=10$
Hence, 10 children out of 40 like playing football.
TRY THESE
1. Find:
(a) $50 %$% of 164
(b) $75 %$% of 12
(c) $12 \frac{1}{2} %$% of 64
2. $8 %$% children of a class of 25 like getting wet in the rain. How many children like getting wet in the rain.
Example 7 Rahul bought a sweater and saved $₹ 200$ when a discount of $25 %$% was given. What was the price of the sweater before the discount?
Solution
Rahul has saved ₹ 200 when price of sweater is reduced by $25 %$%. This means that $25 %$% reduction in price is the amount saved by Rahul. Let us see how Mohan and Abdul have found the original cost of the sweater.
Mohan’s solution
$25 %$% of the original price $=₹ 200$
Let the price (in ₹) be $P$
So, $25 %$% of $P=200$ or $\frac{25}{100} \times P=200$
or, $\frac{P}{4}=200$ or $P=200 \times 4$
Therefore, $P=800$
Abdul’s solution
₹ 25 is saved for every ₹ 100
Amount for which ₹ 200 is saved
$ =\frac{100}{25} \times 200=₹ 800 $
Thus both obtained the original price of sweater as ₹ 800 .
TRY THESE
- 9 is $25 %$% of what number?
- $75 %$% of what number is 15 ?
EXERCISE 7.1
1. Convert the given fractional numbers to per cents.
(a) $\frac{1}{8}$
(b) $\frac{5}{4}$
(c) $\frac{3}{40}$
(d) $\frac{2}{7}$
2. Convert the given decimal fractions to per cents.
(a) 0.65
(b) 2.1
(c) 0.02
(d) 12.35
3. Estimate what part of the figures is coloured and hence find the per cent which is coloured.
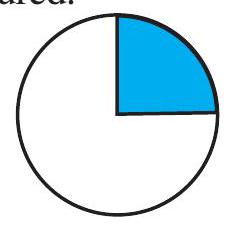
(i)
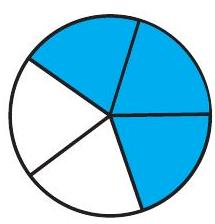
(ii)
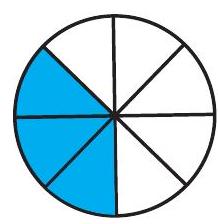
(iii)
4. Find:
(a) $15 %$% of 250
(b) $1 %$% of 1 hour
(c) $20 %$% of ₹ 2500
(d) $75 %$% of $1 kg$
5. Find the whole quantity if
(a) $5 %$% of it is 600 .
(b) $12 %$% of it is ₹ 1080 .
(c) $40 %$% of it is $500 km$.
(d) $70 %$% of it is 14 minutes.
(e) $8 %$% of it is 40 litres.
6. Convert given per cents to decimal fractions and also to fractions in simplest forms:
(a) $25 %$%
(b) $150 %$%
(c) $20 %$%
(d) $5 %$%
7. In a city, $30 %$% are females, $40 %$% are males and remaining are children. What per cent are children?
8. Out of 15,000 voters in a constituency, $60 %$% voted. Find the percentage of voters who did not vote. Can you now find how many actually did not vote?
9. Meeta saves $₹ 4000$ from her salary. If this is $10 %$% of her salary. What is her salary?
10. A local cricket team played 20 matches in one season. It won $25 %$% of them. How many matches did they win?
7.2.3 Ratios to Percents
Sometimes, parts are given to us in the form of ratios and we need to convert those to percentages. Consider the following example:
Example 8 Reena’s mother said, to make idlis, you must take two parts rice and one part urad dal. What percentage of such a mixture would be rice and what percentage would be urad dal?
Solution
In terms of ratio we would write this as Rice : Urad dal $=2: 1$.
Now, $2+1=3$ is the total of all parts. This means $\frac{2}{3}$ part is rice and $\frac{1}{3}$ part is urad dal.
Then, percentage of rice would be $\frac{2}{3} \times 100 $%=$\frac{200}{3}=66 \frac{2}{3} %$%.
Percentage of urad dal would be $\frac{1}{3} \times 100 $%=$\frac{100}{3}=33 \frac{1}{3} %$%.
Example 9 If ₹ 250 is to be divided amongst Ravi, Raju and Roy, so that Ravi gets two parts, Raju three parts and Roy five parts. How much money will each get? What will it be in percentages?
Solution
The parts which the three boys are getting can be written in terms of ratios as $2: 3: 5$. Total of the parts is $2+3+5=10$.
| Amounts received by each | Percentage og money for each |
|---|---|
| $\frac{2}{10} \times ₹ 250=₹ 50$ | $\text{ Ravi gets } \frac{2}{10} \times$ 100 %=20% |
| $\frac{3}{10} \times ₹ 250=₹ 75$ | $\text{ Raju gets } \frac{3}{10} \times$ 100 %=30 |
| $\frac{5}{10} \times ₹ 250=₹ 125$ | $\text{ Roy gets } \frac{5}{10} \times$ 100 %=50 |
TRY THESE
1. Divide 15 sweets between Manu and Sonu so that they get $20 %$% and $80 %$% of them respectively.
2. If angles of a triangle are in the ratio $2: 3: 4$. Find the value of each angle.
7.2.4 Increase or Decrease as Per Cent
There are times when we need to know the increase or decrease in a certain quantity as percentage. For example, if the population of a state increased from 5,50,000 to $6,05,000$. Then the increase in population can be understood better if we say, the population increased by $10 %$.
How do we convert the increase or decrease in a quantity as a percentage of the initial amount? Consider the following example.
Example 10 A school team won 6 games this year against 4 games won last year. What is the per cent increase?
Solution
The increase in the number of wins (or amount of change) $=6-4=2$.
$ \begin{aligned} \text{ Percentage increase } & =\frac{\text{ amount of change }}{\text{ original amount or base }} \times 100 \\ & =\frac{\text{ increase in the number of wins }}{\text{ original number of wins }} \times 100=\frac{2}{4} \times 100=50 \end{aligned} $
Example 11 The number of illiterate persons in a country decreased from 150 lakhs to 100 lakhs in 10 years. What is the percentage of decrease?
Solution
Original amount $=$ the number of illiterate persons initially $=150$ lakhs.
Amount of change $=$ decrease in the number of illiterate persons $=150-100=50$ lakhs Therefore, the percentage of decrease
$ =\frac{\text{ amount of change }}{\text{ original amount }} \times 100=\frac{50}{150} \times 100=33 \frac{1}{3} $
TRY THESE
1. Find Percentage of increase or decrease:
- Price of shirt decreased from ₹ 280 to ₹ 210.
- Marks in a test increased from 20 to 30.
2. My mother says, in her childhood petrol was ₹ 1 a litre. It is ₹ 52 per litre today. By what Percentage has the price gone up?
7.3 PRICES RELATED TO AN ITEM OR BUYING AND SELLIVG
I bought it for ₹ 600

The buying price of any item is known as its cost price. It is written in short as CP. The price at which you sell is known as the selling price or in short SP.
What would you say is better, to you sell the item at a lower price, same price or higher price than your buying price? You can decide whether the sale was profitable or not depending on the $CP$ and $SP$. If $CP<SP$ then you made a profit $=SP-CP$.
$ \begin{aligned} & \text{ If } CP=SP \text{ then you are in a no profit no loss situation. } \\ & \text{ If } CP>SP \text{ then you have a loss }=CP-SP \text{. } \end{aligned} $
Let us try to interpret the statements related to prices of items.

- A toy bought for ₹ 72 is sold at ₹ 80 .
- A T-shirt bought for ₹ 120 is sold at ₹ 100 .
- A cycle bought for ₹ 800 is sold for ₹ 940 .
Let us consider the first statement.
The buying price (or CP) is ₹ 72 and the selling price (or SP) is ₹ 80. This means SP is more than CP. Hence profit made $=SP-CP=₹ 80-₹ 72=₹ 8$
Now try interpreting the remaining statements in a similar way.
7.3.1 Profit or Loss as a Percentage
The profit or loss can be converted to a percentage. It is always calculated on the CP.
For the above examples, we can find the profit $%$ or loss $%$.
Let us consider the example related to the toy. We have $CP=₹ 72, SP=₹ 80$, Profit $=₹ 8$. To find the percentage of profit, Neha and Shekhar have used the following methods.
Neha does it this way
Profit percent=$\frac{\text{Profit}}{CP}\times 100=\frac{8}{72}\times 100$
$=\frac{1}{9}\times 100=11\frac{1}{9}$
Shekhar does it this way
On ₹ 72 the profit is ₹ 8
On ₹ 100, profit=$\frac{8}{72}\times 100$
$=11\frac{1}{9}.$ Thus, profit percent $11\frac{1}{9}$
Thus, the profit is ₹ 8 and profit percent is $11\frac{1}{9}$.
Similarly you can find the loss per cent in the second situation. Here,
$CP=₹ 120,SP=₹ 100.$
Therefore, Loss =$₹ 120- ₹ 100=₹ 20$
Loss percent$=\frac{Loss}{CP}\times 100$
$=\frac{20}{120}\times 100$
$\frac{50}{3}=16\frac{2}{3}$
On ₹ 120, the loss is ₹ 20
$\frac{20}{120}\times 100=\frac{50}{3}=16\frac{2}{3}$
Thus, loss percent is$16\frac{2}{3}$
Try the last case.
Now we see that given any two out of the three quantities related to prices that is, CP, SP, amount of Profit or Loss or their percentage, we can find the rest.
Examiple 12 The cost of a flower vase is ₹ 120 . If the shopkeeper sells it at a loss of $10 %$, find the price at which it is sold.
Solution
We are given that $CP=₹ 120$ and Loss per cent $=10$. We have to find the SP.
Sohan does it like this
Loss of $10 %$ means if CP is ₹ 100 , Loss is ₹ 10
Therefore, SP would be
$₹(100-10)=₹ 90$
When CP is ₹ 100 , SP is ₹ 90 .
Therefore, if CP were ₹ 120 then
$SP=\frac{90}{100} \times 120=₹ 108$
Anandi does it like this
Loss is $10 %$ of the cost price
$ \begin{aligned} & =10 % \text{ of ₹ } 120 \\ & =\frac{10}{100} \times 120=₹ 12 \end{aligned} $
Therefore $ \begin{aligned} SP & =CP-\text{ Loss } \\ & =₹ 120-₹ 12=₹ 108 \end{aligned} $
Thus, by both methods we get the SP as ₹ 108 .
Example 13 Selling price of a toy car is ₹ 540 . If the profit made by shopkeeper is $20 %$, what is the cost price of this toy?
Solution
We are given that $SP=₹ 540$ and the Profit $=20 %$. We need to find the CP.
| Amina does it like this |
|---|
| $20 %$% profit will mean if $CP$ is $₹ 100$, |
| profit is ₹ 20 |
| Therefore, $SP=100+20=120$ |
| Now, when $SP$ is $₹ 120$, |
| then $CP$ is $₹ 100$. |
| Therefore, when $SP$ is ₹ 540 , |
| then $CP=\frac{100}{120} \times 540=₹ 450$ |
| Arun does it like this |
|---|
| Profit $=20 %$% of $CP$ and $SP=CP+$ Profit |
| So, $540=CP+20 %$% of $CP$ |
| $=CP+\frac{20}{100} \times CP=[1+\frac{1}{5}] CP$ |
| $=\frac{6}{5} CP$. Therefore, $540 \times \frac{5}{6}=CP$ |
| or $₹ 450=CP$ |
Thus, by both methods, the cost price is ₹ 450 .
TRY THESE
1. A shopkeeper bought a chair for $₹ 375$ and sold it for $₹ 400$%. Find the gain Percentage.
2. Cost of an item is ₹ 50 . It was sold with a profit of $12 %$%. Find the selling price.
3. An article was sold for ₹ 250 with a profit of $5 %$%. What was its cost price?
4. An item was sold for ₹ 540 at a loss of $5 %$%. What was its cost price?
7.4 CHARGE GIVEN ON BORROWED MONEY OR SIMPLE INTEREST
Sohini said that they were going to buy a new scooter. Mohan asked her whether they had the money to buy it. Sohini said her father was going to take a loan from a bank. The money you borrow is known as sum borrowed or principal.
This money would be used by the borrower for some time before it is returned. For keeping this money for some time the borrower has to pay some extra money to the bank. This is known as Interest.
You can find the amount you have to pay at the end of the year by adding the sum borrowed and the interest. That is, Amount = Principal + Interest.
Interest is generally given in per cent for a period of one year. It is written as say $10 %$% per year or per annum or in short as $10 %$% p.a. (per annum).
$10 %$% p.a. means on every ₹ 100 borrowed, ₹ 10 is the interest you have to pay for one year. Let us take an example and see how this works.
Example 14 Anita takes a loan of ₹ 5,000 at $15 %$% per year as rate of interest. Find the interest she has to pay at the end of one year.
Solution
The sum borrowed $=₹ 5,000$, Rate of interest $=15 %$ per year.
This means if ₹ 100 is borrowed, she has to pay ₹ 15 as interest for one year. If she has borrowed ₹ 5,000 , then the interest she has to pay for one year
$ =₹ \frac{15}{100} \times 5000=₹ 750 $
So, at the end of the year she has to give an amount of ₹ $5,000+₹ 750=₹ 5,750$.
We can write a general relation to find interest for one year. Take $P$ as the principal or sum and $R %$ as Rate per cent per annum.
Now on every ₹ 100 borrowed, the interest paid is ₹ $R$
Therefore, on ₹ $P$ borrowed, the interest paid for one year would be $\frac{R \times P}{100}=\frac{P \times R}{100}$.
7.4.1 Interest for Multiple Years
If the amount is borrowed for more than one year the interest is calculated for the period the money is kept for. For example, if Anita returns the money at the end of two years and the rate of interest is the same then she would have to pay twice the interest i.e., ₹ 750 for the first year and ₹ 750 for the second. This way of calculating interest where principal is not changed is known as simple interest. As the number of years increase the interest also increases. For ₹ 100 borrowed for 3 years at $18 %$, the interest to be paid at the end of 3 years is $18+18+18=3 \times 18=₹ 54$.
We can find the general form for simple interest for more than one year.
We know that on a principal of ₹ $P$ at $R %$ rate of interest per year, the interest paid for one year is $\frac{R \times P}{100}$. Therefore, interest $I$ paid for $T$ years would be
$ \frac{T \times R \times P}{100}=\frac{P \times R \times T}{100} \text{ or } \frac{P R T}{100} $
And amount you have to pay at the end of $T$ years is $A=P+I$
TRY THESE
1. ₹ 10,000 is invested at $5 %$ interest rate p.a. Find the interest at the end of one year.
2. ₹ 3,500 is given at $7 %$ p.a. rate of interest. Find the interest which will be received at the end of two years.
3. ₹ 6,050 is borrowed at $6.5 %$ rate of interest p.a.. Find the interest and the amount to be paid at the end of 3 years.
4. ₹ 7,000 is borrowed at $3.5 %$ rate of interest p.a. borrowed for 2 years. Find the amount to be paid at the end of the second year.
Just as in the case of prices related to items, if you are given any two of the three quantities in the relation $I=\frac{P \times T \times R}{100}$, you could find the remaining quantity.
Example 15 If Manohar pays an interest of ₹ 750 for 2 years on a sum of $₹ 4,500$, find the rate of interest.
| Solution 1 | Solution 2 |
|---|---|
| $P \times T \times R$ | For 2 years, interest paid is $₹ 750$ |
| $1=\frac{100}{10}$ | Therefore, for 1 vear, interest paid $₹ \underline{750}=₹ 375$ |
| Therefore, $750=\frac{4500 \times 2 \times R}{100}$ | On ₹ 4,500 , interest paid is ₹ 375 |
| or $\quad \frac{750}{45 \times 2}=R^{100}$ | Therefore, on ₹ 100 , rate of interest paid |
| Therefore, Rate $=8 \frac{1}{3} %$ | $=\frac{375 \times 100}{4500}=8 \frac{1}{3} %$ |
TRY THESE
1. You have $₹ 2,400$ in your account and the interest rate is $5 %$%. After how many years would you earn ₹ 240 as interest.
2. On a certain sum the interest paid after 3 years is $₹ 450$ at $5 %$% rate of interest per annum. Find the sum.
EXERCISE 7.2
1. Tell what is the profit or loss in the following transactions. Also find profit per cent or loss per cent in each case.
(a) Gardening shears bought for ₹ 250 and sold for ₹ 325 .
(b) A refrigerater bought for ₹ 12,000 and sold at ₹ 13,500 .
(c) A cupboard bought for ₹ 2,500 and sold at ₹ 3,000.
(d) A skirt bought for ₹ 250 and sold at ₹ 150 .
2. Convert each part of the ratio to percentage:
(a) $3: 1$
(b) $2: 3: 5$
(c) $1: 4$
(d) $1: 2: 5$
3. The population of a city decreased from 25,000 to 24,500 . Find the percentage decrease.
4. Arun bought a car for ₹ $3,50,000$. The next year, the price went upto $₹ 3,70,000$. What was the Percentage of price increase?
5. I buy a T.V. for ₹ 10,000 and sell it at a profit of $20 %$%. How much money do I get for it?
6. Juhi sells a washing machine for ₹ 13,500 . She loses $20 %$% in the bargain. What was the price at which she bought it?
7. (i) Chalk contains calcium, carbon and oxygen in the ratio 10:3:12. Find the percentage of carbon in chalk.
(ii) If in a stick of chalk, carbon is $3 g$, what is the weight of the chalk stick?
8. Amina buys a book for ₹ 275 and sells it at a loss of $15 %$%. How much does she sell itfor?
9. Find the amount to be paid at the end of 3 years in each case:
(a) Principal $=₹ 1,200$ at $12 %$% p.a.
(b) Principal $=₹ 7,500$ at $5 %$% p.a.
10. What rate gives ₹ 280 as interest on a sum of ₹ 56,000 in 2 years?
11. If Meena gives an interest of ₹ 45 for one year at $9 %$% rate p.a.. What is the sum she has borrowed?
WHAT HAVE WE DISCUSSED?
1. A way of comparing quantities is percentage. Percentages are numerators of fractions with denominator 100. Per cent means per hundred.
For example $82 %$ marks means 82 marks out of hundred.
2. Fractions can be converted to percentages and vice-versa.
For example, $\frac{1}{4}=\frac{1}{4} \times 100 %$% whereas, 75%$=\frac{75}{100}=\frac{3}{4}$
3. Decimals too can be converted to percentages and vice-versa.
For example, $0.25=0.25 \times 100 $%==25%
4. Percentages are widely used in our daily life,
(a) We have learnt to find exact number when a certain per cent of the total quantity is given.
(b) When parts of a quantity are given to us as ratios, we have seen how to convert them to percentages.
(c) The increase or decrease in a certain quantity can also be expressed as percentage.
(d) The profit or loss incurred in a certain transaction can be expressed in terms of percentages.
(e) While computing interest on an amount borrowed, the rate of interest is given in terms of per cents. For example, ₹ 800 borrowed for 3 years at $12 %$ per annum.










