JEE Main 12 Jan 2019 Morning Question 7
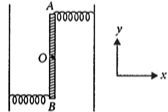
Question: Two light identical springs of spring constant k are attached horizontally at the two ends of a uniform horizontal rod AB of length l and mass m. The rod is pivoted at its centre O and can rotate freely in horizontal plane. The other ends of the two springs are fixed to rigid supports as shown in figure. The rod is gently pushed through a small angle and released. The frequency of resulting oscillation is [JEE Main Online Paper Held On 12-Jan-2019 Morning]
Options:
A) $ \frac{1}{2\pi }\sqrt{\frac{k}{m}} $
B) $ \frac{1}{2\pi }\sqrt{\frac{6k}{m}} $
C) $ \frac{1}{2\pi }\sqrt{\frac{3k}{m}} $
D) $ \frac{1}{2\pi }\sqrt{\frac{2k}{m}} $
Show Answer
Answer:
Correct Answer: B
Solution:
Let the rod be rotated through a small angle $ \theta . $ .
Due to restoring force of the spring, the torque acting on the rod is
$ \tau =( \frac{l}{2} )(kx)+\frac{l}{2}(kx) $ $ =( \frac{l}{2} )( k\frac{l}{2}\theta )+\frac{l}{2}(k)( \frac{l}{2}\theta )=\frac{l^{2}k\theta }{2} $ ….(i)
Also, $ \tau =\frac{ml^{2}}{12}\alpha $ …(ii)
Using equations (i) and (ii),
$ \frac{\alpha ml^{2}}{12}=\frac{l^{2}k\theta }{2}\Rightarrow \frac{\alpha m}{6}=k\theta $ $ \alpha =\frac{6k}{m}\theta ={{\omega }^{2}}\theta $
$ \Rightarrow $ $ \omega =\sqrt{\frac{6k}{m}};T=\frac{\omega }{2\pi }=\frac{1}{2\pi }\sqrt{\frac{6k}{m}} $










