Wave Motion 4 Question 19
18. Two men are walking along a horizontal straight line in the same direction. The main in front walks at a speed $1.0 ms^{-1}$ and the man behind walks at a speed $2.0 ms^{-1}$. A third man is standing at a height $12 m$ above the same horizontal line such that all three men are in a vertical plane. The two walking men are blowing identical whistles which emit a sound of frequency $1430 Hz$. The speed of sound in air $330 ms^{-1}$. At the instant, when the moving men are $10 m$ apart, the stationary man is equidistant from them. The frequency of beats in $Hz$, heard by the stationary man at this instant, is ………
(2018 Adv.)
Show Answer
Answer:
Correct Answer: 18. 5
Solution:
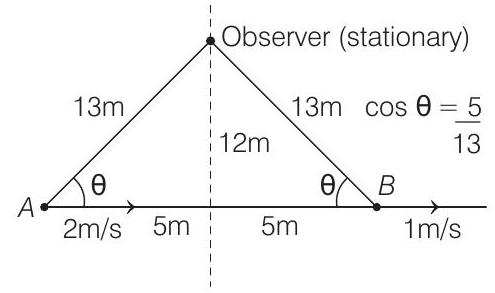
$$ \begin{aligned} f _A & =1430\left[\frac{330}{330-2 \cos \theta}\right] \\ & =1430\left[\frac{1}{1-\frac{2 \cos \theta}{330}}\right] \approx 1430\left[1+\frac{2 \cos \theta}{330}\right] \end{aligned} $$
[from binomial expansion]
$$ f _B=1430\left[\frac{330}{330+1 \cos \theta}\right] \approx 1430\left[1-\frac{\cos \theta}{330}\right] $$
$$ \begin{aligned} \text { Beat frequency } & =f _A-f _B=1430\left[\frac{3 \cos \theta}{330}\right]=13 \cos \theta \\ & =13\left(\frac{5}{13}\right)=5.00 Hz \end{aligned} $$






