Wave Motion 2 Question 15
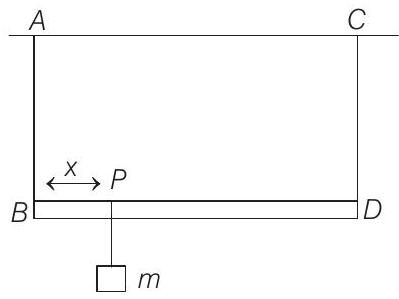
15. A massless $\operatorname{rod} B D$ is suspended by two identical massless strings $A B$ and $C D$ of equal lengths. A block of mass $m$ is suspended from point $P$ such that $B P$ is equal to $x$. If the fundamental frequency of the left wire is twice the fundamental frequency of right wire, then the value of $x$ is
(2006)
(a) $l / 5$
(b) $l / 4$
(c) $4 l / 5$
(d) $3 l / 4$
Show Answer
Answer:
Correct Answer: 15. (a)
Solution:
- $f \propto v \propto \sqrt{T} \Rightarrow f _{A B}=2 f _{C D}$
$\therefore \quad T _{A B}=4 T _{C D}$
Further
$$ \Sigma \tau _p=0 $$
$\begin{array}{rlrl}\therefore & T _{A B}(x) & =T _{C D}(l-x) \ \text { or } & 4 x & =l-x \ \text { or }\end{array} \quad\left(\right.$ as $\left.T _{A B}=4 T _{C D}\right)$
or
$$ x=l / 5 $$






