SATHEE: Simple Harmonic Motion 4 Question 8

Simple Harmonic Motion 4 Question 8

8. A uniform rod of length $L$ and mass $M$ is pivoted at the centre. Its two ends are attached to two springs of equal spring constants $k$ . The springs are fixed to rigid supports as shown in the figure, and rod is free to oscillate in the horizontal plane.

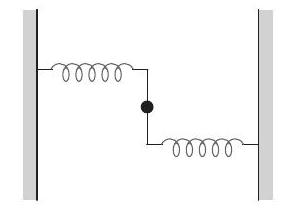
The rod is gently pushed through a small angle $θ$ in one direction and released. The frequency of oscillation is (2009)

(a) $\frac{1}{2 π} \sqrt{\frac{2 k}{M}}$

(b) $\frac{1}{2 π} \sqrt{\frac{k}{M}}$

(d) $\frac{1}{2 π} \sqrt{\frac{24 k}{M}}$

Show Answer

Answer:

Correct Answer: 8. (c)

Solution:

$x = \frac{L}{2} θ$ ,

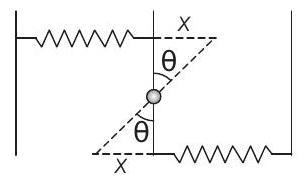
Restoring torque $= - (2 k x) \cdot \frac{L}{2}$

$\begin{aligned} α & = - \frac{k L (L / 2 θ)}{I} = - \frac{k L^{2} / 2}{M L^{2} / 12} \cdot θ = - \frac{6 k}{M} θ \\ ∴ f & = \frac{1}{2 π} \sqrt{| \frac{α}{θ} |} = \frac{1}{2 π} \sqrt{\frac{6 k}{M}} \end{aligned}$

Simple Harmonic Motion 4 Question 8

8. A uniform rod of length $L$ and mass $M$ is pivoted at the centre. Its two ends are attached to two springs of equal spring constants $k$ . The springs are fixed to rigid supports as shown in the figure, and rod is free to oscillate in the horizontal plane.

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Simple Harmonic Motion 4 Question 8

8. A uniform rod of length L and mass M is pivoted at the centre. Its two ends are attached to two springs of equal spring constants k. The springs are fixed to rigid supports as shown in the figure, and rod is free to oscillate in the horizontal plane.

Answer:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu

8. A uniform rod of length $L$ and mass $M$ is pivoted at the centre. Its two ends are attached to two springs of equal spring constants $k$ . The springs are fixed to rigid supports as shown in the figure, and rod is free to oscillate in the horizontal plane.