Simple Harmonic Motion 4 Question 4
4. A block of mass $m$ lying on a smooth horizontal surface is attached to a spring (of negligible mass) of spring constant $k$. The other end of the spring is fixed as shown in the figure. The block is initially at rest in its equilibrium position. If now the block is pulled with a constant force $F$, the maximum speed of the block is
(2019 Main, 09 Jan I)
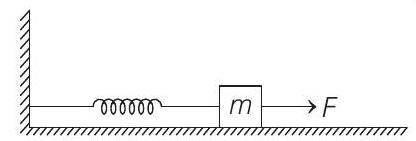
(a) $\frac{\pi F}{\sqrt{m k}}$
(b) $\frac{F}{\sqrt{m k}}$
(c) $\frac{2 F}{\sqrt{m k}}$
(d) $\frac{F}{\pi \sqrt{m k}}$
Show Answer
Answer:
Correct Answer: 4. (b)
Solution:
- In a spring-block system, when a block is pulled with a constant force $F$, then its speed is maximum at the mean position. Also, it’s acceleration will be zero.
In that case, force on the system is given as,
$$ F=k x $$
where, $x$ is the extension produced in the spring.
$$ \text { or } \quad x=\frac{F}{k} $$
Now we know that, for a system vibrating at its mean position, its maximum velocity is given as,
$$ v _{\max }=A \omega $$
where, $A$ is the amplitude and $\omega$ is the angular velocity.
Since, the block is at its mean position.
$$ \text { So, } \begin{aligned} A & =x=\frac{F}{k} \\ v _{\max } & =\frac{F}{k} \sqrt{\frac{k}{m}} \quad \because \omega=\sqrt{\frac{k}{m}}=\frac{F}{\sqrt{k m}} \end{aligned} $$
Alternate Method
According to the work-energy theorem, net work done $=$ change in the kinetic energy
Here, net work done $=$ work done due to external force $\left(W _{\text {ext }}\right)+$ work done due to the spring $\left(W _{\text {spr }}\right)$.
$$ \begin{aligned} & \text { As, } \quad W _{\text {ext }}=F \cdot x \\ & \text { and } \quad W _{spr}=\frac{-1}{2} k x^{2} \\ & \Rightarrow \quad \Delta KE=F \cdot x+-\frac{1}{2} k x^{2} \\ & (\Delta KE) _f-(\Delta KE) _i=F \cdot x-\frac{1}{2} k x^{2} \\ & \Rightarrow \quad \frac{1}{2} m v _{\max }^{2}-\frac{1}{2} m(0)^{2}=F \cdot \frac{F}{k}-\frac{1}{2} k \frac{F}{k}^{2} \\ & \Rightarrow \quad \frac{1}{2} m v _{\max }^{2}=\frac{F^{2}}{k}-\frac{F^{2}}{2 k}=\frac{F^{2}}{2 k} \\ & \text { or } \quad v _{\max }^{2}=\frac{F^{2}}{km} \\ & \Rightarrow \quad v _{\max }=F / \sqrt{km} \end{aligned} $$
