Optics 6 Question 3
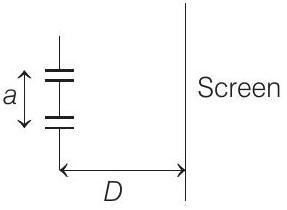
4. The figure shows a Young’s double slit experimental setup. It is observed that when a thin transparent sheet of thickness $t$ and refractive index $\mu$ is put in front of one of the slits, the central maximum gets shifted by a distance equal to $n$ fringe widths. If the wavelength of light used is $\lambda, t$ will be
(a) $\frac{2 n D \lambda}{a(\mu-1)}$
(b) $\frac{2 D \lambda}{a(\mu-1)}$
(c) $\frac{D \lambda}{a(\mu-1)}$
(d) $\frac{n D \lambda}{a(\mu-1)}$
Show Answer
Answer:
Correct Answer: 4. (*)
Solution:
- Path difference introduced by a slab of thickness $t$ and refractive index $\mu$ is given by
$$ \Delta=(\mu-1) t $$
Position of the fringe is $x=\frac{\Delta D}{d}=\frac{(\mu-1) t D}{d}$
Also, fringe width is given by
$$ \beta=\frac{\lambda D}{d} $$
According to the question,
$$ \begin{aligned} & n \beta & =x \\ \Rightarrow \quad & \frac{n \lambda D}{d} & =(\mu-1) t \frac{D}{d} \\ \Rightarrow n \lambda=(\mu-1) t & \text { or } t & =\frac{n \lambda}{(\mu-1)} \end{aligned} $$
Alternate Solution
Path difference, $\Delta=\frac{x d}{D}=(\mu-1) t$
$$ \text { or } \quad t=\frac{x d}{(\mu-1) D} $$
$$ \begin{aligned} & \text { Also, } \quad \beta=\frac{\lambda D}{d} \Rightarrow \frac{d}{D}=\frac{\lambda}{\beta} \\ & \therefore \quad t=\frac{x \lambda}{(\mu-1) \beta}=\frac{n \lambda D \times \lambda}{d(\mu-1) \beta} \quad[\because x=n \beta] \\ & \Rightarrow \quad t=\frac{n \lambda}{(\mu-1)} \end{aligned} $$
So, no option is correct.
