Optics 4 Question 18
19. A monochromatic beam of light is incident at $60^{\circ}$ on one face of an equilateral prism of refractive index $n$ and emerges from the opposite face making an angle $\theta(n)$ with the normal (see figure). For $n=\sqrt{3}$ the value of $\theta$ is $60^{\circ}$ and $\frac{d \theta}{d n}=m$. The value of $m$ is
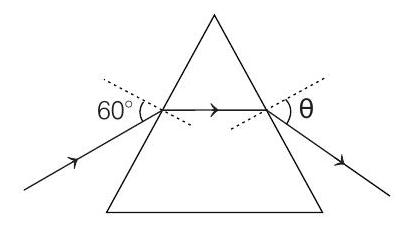
(2015 Adv.)
Show Answer
Answer:
Correct Answer: 19. (2)
Solution:
- Applying Snell’s law at $M$ and $N$,
$$ \begin{aligned} \sin 60^{\circ} & =n \sin r \cdots(i)\\ \sin \theta & =n \sin (60-r)\cdots(ii) \end{aligned} $$
Differentiating we get
$$ \begin{aligned} & \cos \theta \frac{d \theta}{d n}=-n \cos (60-r) \frac{d r}{d n} \\ &+\sin (60-r) \end{aligned} $$
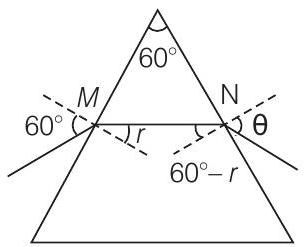
Differentiating Eq. (i),
$$ \begin{aligned} & n \cos r \frac{d r}{d n}+\sin r=0 \\ & \text { or } \quad \frac{d r}{d n}=-\frac{\sin r}{n \cos r}=\frac{-\tan r}{n} \\ & \Rightarrow \quad \cos \theta \frac{d \theta}{d n}=-n \cos (60-r) \frac{-\tan r}{n}+\sin (60-r) \\ & \frac{d \theta}{d n}=\frac{1}{\cos \theta}[\cos (60-r) \tan r+\sin (60-r)] \end{aligned} $$
Form Eq. (i), $\quad r=30^{\circ}$ for $n=\sqrt{3}$
$\Rightarrow \frac{d \theta}{d n}=\frac{1}{\cos 60}(\cos 30 \times \tan 30+\sin 30)=2 \Big(\frac{1}{2}+\frac{1}{2}\Big)=2$






