Kinematics 2 Question 4
6. From a tower of height
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 6. (c)
Solution:
- Time taken to reach the maximum height,
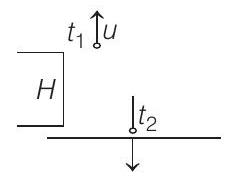
If
[Given]






