Electrostatics 6 Question 6
6. Capacitor $C_{1}$ of capacitance $1 \mu \mathrm{F}$ and capacitor $C_{2}$ of capacitance $2 \mu \mathrm{F}$ are separately charged fully by a common battery. The two capacitors are then separately allowed to discharge through equal resistors at time $t=0 .$
$(1989,2 \mathrm{M})$
(a) The current in each of the two discharging circuits is zero at $t=0$
(b) The currents in the two discharging circuits at $t=0$ are equal but not zero
(c) The currents in the two discharging circuits at $t=0$ are unequal
(d) Capacitor $C_{1}$, loses $50 %$ of its initial charge sooner than $\mathrm{C}_{2}$ loses $50 %$ of its initial charge
Show Answer
Answer:
Correct Answer: 6. (b,d)
Solution:
- The discharging current in the circuit is,
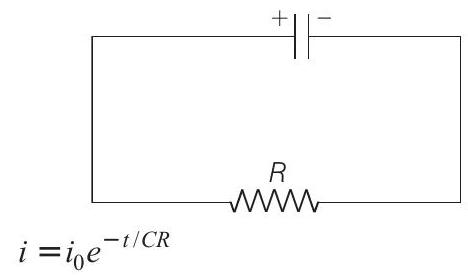
Here, $i_{0}=$ initial current $=\frac{V}{R}$
Here, $V$ is the potential with which capacitor was charged.
Since, $V$ and $R$ for both the capacitors are same, initial discharging current will be same but non-zero.
$\therefore$ Correct option is (b).
Further, $\tau_{c}=C R \Rightarrow C_{1}<C_{2}$ or $\tau_{C_{1}}<\tau_{C_{2}}$ or $C_{1}$ loses its $50 %$ of initial charge sooner than $C_{2}$.
$\therefore$ Option (d) is also correct .






