Current Electricity 2 Question 29
29. In the circuit shown in figure $E _1=3 V, E _2=2 V$,
$E _3=1 V$ and $R=r _1=r _2=r _3=1 \Omega$.
$(1981,6 M)$
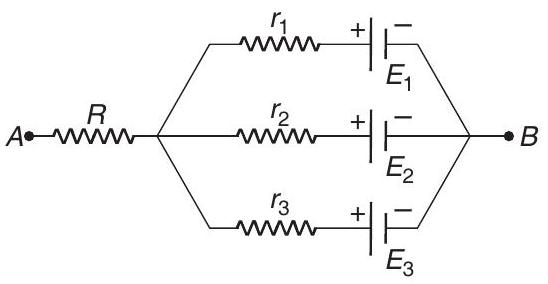
(a) Find the potential difference between the points $A$ and $B$ and the currents through each branch.
(b) If $r _2$ is short-circuited and the point $A$ is connected to point $B$, find the currents through $E _1, E _2, E _3$ and the resistor $R$.
Show Answer
Solution:
- (a) Equivalent emf of three batteries would be
$$ E _{eq}=\frac{\sum(E / r)}{\Sigma(1 / r)}=\frac{(3 / 1+2 / 1+1 / 1)}{(1 / 1+1 / 1+1 / 1)}=2 V $$
Further $r _1, r _2$ and $r _3$ each are of $1 \Omega$. Therefore, internal resistance of the equivalent battery will be $\frac{1}{3} \Omega$ as all three are in parallel.
The equivalent circuit is therefore shown in the given figure.
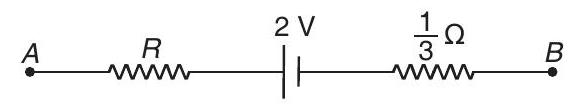
Since, no current is taken from the battery.
$$ \begin{aligned} & \qquad V _{A B}=2 V(\text { From } V=E-i r) \\ & \text { Further, } V _{A B}+V _A-V _B=E _1-i _1 r _1 \\ & \therefore \quad i _1=\frac{V _B-V _A+E _1}{r _1}=\frac{-2+3}{1}=1 A \\ & \text { Similarly, } i _2=\frac{V _B-V _A+E _2}{r _2}=\frac{-2+2}{1}=0 \\ & \text { and } \quad i _3=\frac{V _B-V _A+E _3}{r _3}=\frac{-2+1}{1}=-1 A \end{aligned} $$
(b) $r _2$ is short circuited means resistance of this branch becomes zero. Making a closed circuit with a battery and resistance $R$. Applying Kirchhoff’s second law in three loops so formed.
$$ 3-i _1-\left(i _1+i _2+i _3\right)=0 $$
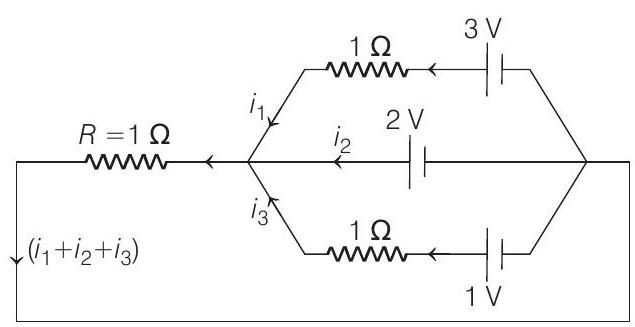
$$ \begin{aligned} 2-\left(i _1+i _2+i _3\right) & =0 \\ 1-i _3-\left(i _1+i _2+i _3\right) & =0 \end{aligned} $$
From Eq. (ii) $\quad i _1+i _2+i _3=2 A$
$\therefore \quad$ Substituting in Eq. (i), we get, $i _1=1 A$
Substituting in Eq. (iii) we get, $i _3=-1 A$
$$ \therefore \quad i _2=2 A $$






