Vectors 2 Question 3
3. If the length of the perpendicular from the point $(\beta, 0, \beta)(\beta \neq 0)$ to the line, $\frac{x}{1}=\frac{y-1}{0}=\frac{z+1}{-1}$ is $\sqrt{\frac{3}{2}}$, then $\beta$ is equal to
(2019 Main, 10 April +I)
(a) 2
(b) -2
(c) -1
(d) 1
Show Answer
Answer:
Correct Answer: 3. (c)
Solution:
- Equation of given line is
$ \frac{x}{1}=\frac{y-1}{0}=\frac{z+1}{-1} $
Now, one of the point on line is $P(0,1,-1)$ and the given point is $Q(\beta, 0, \beta)$.
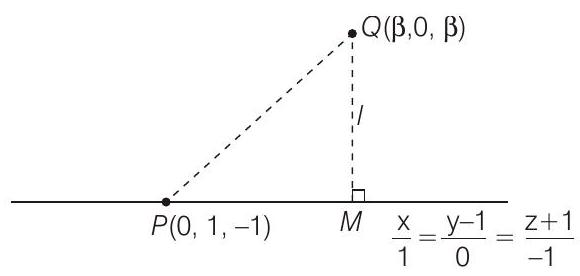
From the figure, the length of the perpendicular
$ \begin{array}{ll} & Q M=l=\sqrt{\frac{3}{2}} \\ \Rightarrow & \frac{|\mathbf{P Q} \times \mathbf{P M}|}{|\mathbf{P M}|}=\sqrt{\frac{3}{2}} \\ \because \quad & \mathbf{P Q}=\beta \hat{\mathbf{i}}-\hat{\mathbf{j}}+(\beta+1) \hat{\mathbf{k}} \end{array} $
and $\mathbf{P M}=$ a vector along given line $(i)=\hat{\mathbf{i}}-\hat{\mathbf{k}}$ So, $\mathbf{P Q} \times \mathbf{P M}=\left|\begin{array}{ccc}\hat{\mathbf{i}} & \hat{\mathbf{j}} & \hat{\mathbf{k}} \ \beta & -1 & \beta+1 \ 1 & 0 & -1\end{array}\right|$
$ =\hat{\mathbf{i}}-\hat{\mathbf{j}}(-\beta-\beta-1)+\hat{\mathbf{k}}=\hat{\mathbf{i}}+(2 \beta+1) \hat{\mathbf{j}}+\hat{\mathbf{k}} $
Now, $\quad \frac{|PQ \times PM|}{|PM|}=\frac{\sqrt{1+(2 \beta+1)^{2}+1}}{\sqrt{2}}$
From Eqs. (ii) and (iii), we get
$ \begin{aligned} & \sqrt{\frac{1+(2 \beta+1)^{2}+1}{2}}=\sqrt{\frac{3}{2}} \Rightarrow \frac{1+(2 \beta+1)^{2}+1}{2}=\frac{3}{2} \\ \Rightarrow & \quad(2 \beta+1)^{2}=1 \Rightarrow 2 \beta+1= \pm 1 \\ \Rightarrow & 2 \beta+1=1 \text { or } 2 \beta+1=-1 \Rightarrow \beta=0 \text { or } \beta=-1 \end{aligned} $
