SATHEE: Straight Line and Pair of Straight Lines 1 Question 22

Straight Line and Pair of Straight Lines 1 Question 22

22. The $x$ -coordinate of the incentre of the triangle that has the coordinates of mid-points of its sides as $(0, 1), (1, 1)$ and $(1, 0)$ is

(2013 Main)

(a) $2 + \sqrt{2}$

(b) $2 - \sqrt{2}$

(c) $1 + \sqrt{2}$

(d) $1 - \sqrt{2}$

Show Answer

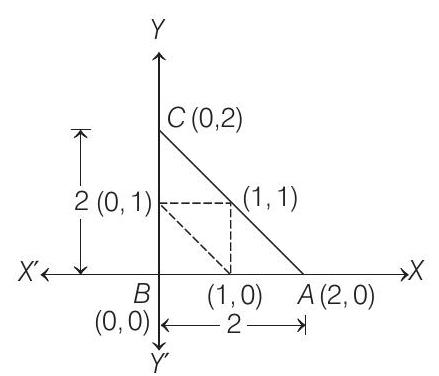
Solution:

Given mid-points of a triangle are $(0, 1), (1, 1)$ and $(1, 0)$ . Plotting these points on a graph paper and make a triangle.

So, the sides of a triangle will be 2,2 and $\sqrt{2^{2} + 2^{2}}$ i.e. $2 \sqrt{2}$ .

$x$ -coordinate of incentre $= \frac{2 \times 0 + 2 \sqrt{2} \cdot 0 + 2 \cdot 2}{2 + 2 + 2 \sqrt{2}}$

$= \frac{2}{2 + \sqrt{2}} \times \frac{2 - \sqrt{2}}{2 - \sqrt{2}} = 2 - \sqrt{2}$

Privacy Policy

Powered by Prutor@IITK

sathee@iitk.ac.in

Media coverage of SATHEE

Play Store

App Store

Ask SATHEE

Welcome to SATHEE !
Select from 'Menu' to explore our services, or ask SATHEE to get started. Let's embark on this journey of growth together! 🌐📚🚀🎓

I'm relatively new and can sometimes make mistakes.
If you notice any error, such as an incorrect solution, please use the thumbs down icon to aid my learning.
To begin your journey now, click on "I understand".

Straight Line and Pair of Straight Lines 1 Question 22

22. The x-coordinate of the incentre of the triangle that has the coordinates of mid-points of its sides as (0,1),(1,1) and (1,0) is

Solution:

Engineering Preparation

Medical Preparation

NCERT Books & Solutions

Important Resources

Other Resources

Syllabus

Test Platform

Sample Mock Test

Mentorship

NCERT Exercise Video Solution

Menu

22. The $x$ -coordinate of the incentre of the triangle that has the coordinates of mid-points of its sides as $(0, 1), (1, 1)$ and $(1, 0)$ is