Hyperbola 1 Question 17
18.
Let $H: \frac{x^{2}}{a^{2}}-\frac{y^{2}}{b^{2}}=1$, where $a>b>0$, be a hyperbola in the $X Y$-plane whose conjugate axis $L M$ subtends an angle of $60^{\circ}$ at one of its vertices $N$. Let the area of the $\triangle L M N$ be $4 \sqrt{3}$.
| List-I | List-II | ||
|---|---|---|---|
| P. | The length of the conjugate axis of $H$ is |
1. | 8 |
| Q. | The eccentricity of $H$ is | 2. | $\frac{4}{\sqrt{3}}$ |
| R. | The distance between the foci of $H$ is |
3. | $\frac{2}{\sqrt{3}}$ |
| S. | The length of the latus rectum of $H$ is |
4. | 4 |
The correct option is
(a) $P \rightarrow 4 ; Q \rightarrow 2 ; R \rightarrow 1 ; S \rightarrow 3$
(b) $P \rightarrow 4 ; Q \rightarrow 3 ; R \rightarrow 1 ; S \rightarrow 2$
(c) $P \rightarrow 4 ; Q \rightarrow 1 ; R \rightarrow 3 ; S \rightarrow 2$
(d) $P \rightarrow 3 ; Q \rightarrow 4 ; R \rightarrow 2 ; S \rightarrow 1$
(2018 Adv.)
Show Answer
Answer:
Correct Answer: 18. (b)
Solution:
- We have,
Equation of hyperbola
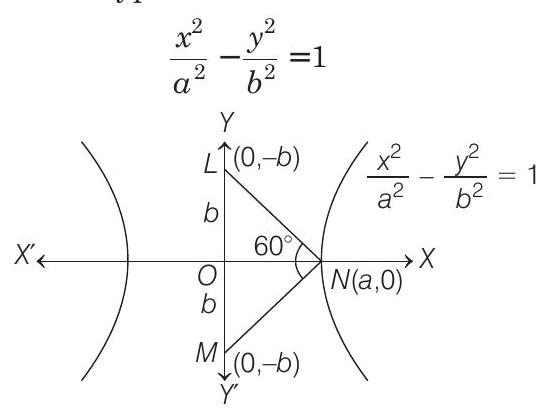
It is given,
$ \angle L N M=60^{\circ} $
and $\quad$ Area of $\triangle L M N=4 \sqrt{3}$
Now, $\triangle L N M$ is an equilateral triangle whose sides is $2 b$
$\because \triangle L O N \cong \triangle M O L ; $
$\therefore \quad \angle N L O=\angle N M O=60^{\circ}$
$\therefore$ Area of $\triangle L M N=\frac{\sqrt{3}}{4}(2 b)^{2}$
$\Rightarrow 4 \sqrt{3}=\sqrt{3} b^{2} \Rightarrow b=2$
Also, area of $\triangle L M N=\frac{1}{2} a(2 b)=a b$
$\Rightarrow \quad 4 \sqrt{3}=a(2) \quad \Rightarrow \quad a=2 \sqrt{3}$
(P) Length of conjugate axis $=2 b=2(2)=4$
(Q) Eccentricity $(e)=\sqrt{1+\frac{b^{2}}{a^{2}}}=\sqrt{1+\frac{4}{12}}=\frac{4}{2 \sqrt{3}}=\frac{2}{\sqrt{3}}$
(R) Distance between the foci $=2 a e=2 \times 2 \sqrt{3} \times \frac{2}{\sqrt{3}}=8$
(S) The length of latusrectum $=\frac{2 b^{2}}{a}=\frac{2(4)}{2 \sqrt{3}}=\frac{4}{\sqrt{3}}$
$P \rightarrow 4 ; Q \rightarrow 3 ; R \rightarrow 1 ; S \rightarrow 2$
