Definite Integration Question 21
Question 21
- If ’ $f$ ’ is a continuous function with $\int_{0}^{x} f(t) d t \rightarrow \infty$ as $|x| \rightarrow \infty$, then show that every line $y=m x$ intersects the curve $y^{2}+\int_{0}^{x} f(t) d t=2$
$(1991,2 M)$
Show Answer
Solution:
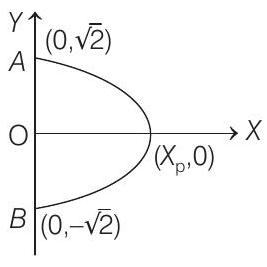
- Since, $f$ is continuous function and $\int_{0}^{x} f(t) d t \rightarrow \infty$, as $|x| \rightarrow \infty$. To show that every line $y=m x$ intersects the curve $y^{2}+\int_{0}^{x} f(t) d t=2$
At $x=0, y= \pm \sqrt{2}$
Hence, $(0, \sqrt{2}),(0,-\sqrt{2})$ are the point of intersection of the curve with the $Y$-axis. As $x \rightarrow \infty, \int_{0}^{x} f(t) d t \rightarrow \infty$ for a particular $x$ (say $x_{n}$ ), then $\int_{0}^{x} f(t) d t=2$ and for this value of $x, y=0$
The curve is symmetrical about $X$-axis.
Thus, we have that there must be some $x$, such that $f\left(x_{n}\right)=2$.
Thus, $y=m x$ intersects this closed curve for all values of $m$.
