Circle 1 Question 4
4. A square is inscribed in the circle $x^{2}+y^{2}-6 x+8 y-103=0$ with its sides parallel to the coordinate axes. Then, the distance of the vertex of this square which is nearest to the origin is
(2019 Main, 11 Jan II)
(a) 6
(b) 13
(c) $\sqrt{41}$
(d) $\sqrt{137}$
Show Answer
Answer:
Correct Answer: 4. (c)
Solution:
- Given equation of circle is $x^{2}+y^{2}-6 x+8 y-103=0$, which can be written as $(x-3)^{2}+(y+4)^{2}=128=(8 \sqrt{2})^{2}$
$\therefore$ Centre $=(3,-4)$ and radius $=8 \sqrt{2}$
Now, according to given information, we have the following figure.
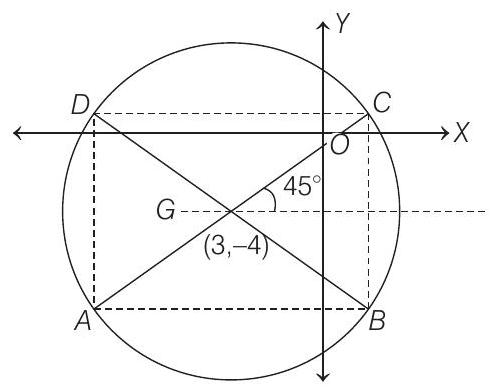
For the coordinates of $A$ and $C$.
Consider, $\frac{x-3}{\frac{1}{\sqrt{2}}}=\frac{y+4}{\frac{1}{\sqrt{2}}}= \pm 8 \sqrt{2}$
[using distance (parametric) form of line,
$ \left.\frac{x-x _1}{\cos \theta}=\frac{y-y _1}{\sin \theta}=r\right] $
$\Rightarrow x=3 \pm 8, y=-4 \pm 8$
$\therefore \quad A(-5,-12)$ and $C(11,4)$
Similarly, for the coordinates of $B$ and $D$, consider
$ \begin{aligned} & \frac{x-3}{-\frac{1}{\sqrt{2}}}=\frac{y+4}{\frac{1}{\sqrt{2}}}= \pm 8 \sqrt{2} \quad\left[\text { in this case, } \theta=135^{\circ}\right. \\ & \Rightarrow \quad x=3 \mp 8, y=-4 \pm 8 \\ & \therefore \quad B(11,-12) \text { and } D(-5,4) \\ & O B=\sqrt{121+144}=\sqrt{265} \\ & O C=\sqrt{121+16}=\sqrt{137} \\ & O D=\sqrt{25+16}=\sqrt{41} \end{aligned} $
