Area Question 32
Question 32
- Find the area bounded by the curves
Show Answer
Solution:
- The region bounded by the curves
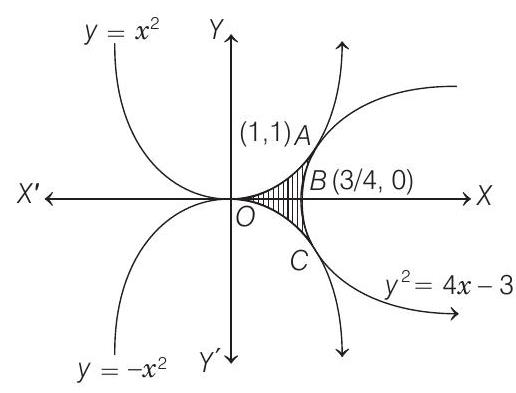
$$ \begin{aligned} & =2{\frac{x^{3}}{3}}{0}^{1}-{\frac{(4 x-3)^{3 / 2}}{3 \cdot 4 / 2}}{3 / 4}^{1} \ & =2 \frac{1}{3}-\frac{1}{6} \ & =1 \cdot \frac{1}{6}=\frac{1}{3} \mathrm{sq} \text { unit } \end{aligned} $$






