SATHEE: Area Question 2

Area Question 2

Question 2

If the area (in sq units) of the region $Missing or unrecognized delimiter for \left$ is $a \sqrt{2} + b$ , then $a - b$ is equal to

(2019 Main, 12 April I) (a) $\frac{10}{3}$ (b) 6 (c) $\frac{8}{3}$ (d) $- \frac{2}{3}$

Show Answer

Solution:

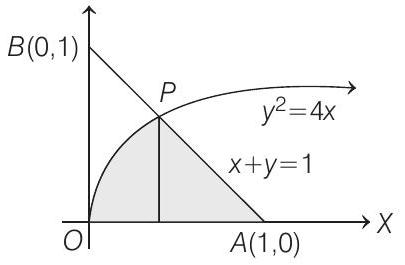
Given region is $Missing or unrecognized delimiter for \left$

Now, for point $P$ , put value of $y = 1 - x$ to $y^{2} = 4 x$ , we get $(1 - x)^{2} = 4 x \Rightarrow x^{2} + 1 - 2 x = 4 x$

$\begin{aligned} \Rightarrow & x^{2} - 6 x + 1 = 0 \Rightarrow x & = \frac{6 \pm \sqrt{36 - 4}}{2} & = 3 \pm 2 \sqrt{2} . \end{aligned}$

Since, $x$ -coordinate of $P$ less than $x$ -coordinate of point $A (1, 0)$ .

$∴ x = 3 - 2 \sqrt{2}$

Now, required area

$= \int_{0}^{3 - 2 \sqrt{2}} 2 \sqrt{x} d x + \int_{3 - 2 \sqrt{2}}^{1} (1 - x) d x$

$=2\left|\frac{x^{3 / 2}}{3 / 2}\right|{0}^{3-2 \sqrt{2}}+x-{\frac{x^{2}}{2}}{3-2 \sqrt{2}}$

$= \frac{4}{3} (3 - 2 \sqrt{2})^{32} + 1 - \frac{1}{2} - (3 - 2 \sqrt{2}) + \frac{(3 - 2 \sqrt{2})^{2}}{2}$

$= \frac{4}{3} {[(\sqrt{2} - 1)^{2}]}^{3 / 2} + \frac{1}{2} - 3 + 2 \sqrt{2} + \frac{1}{2} (9 + 8 - 12 \sqrt{2})$

$= \frac{4}{3} (\sqrt{2} - 1)^{3} - \frac{5}{2} + 2 \sqrt{2} + \frac{17}{2} - 6 \sqrt{2}$

$= \frac{4}{3} (2 \sqrt{2} - 3 (2) + 3 (\sqrt{2}) - 1) - 4 \sqrt{2} + 6$

$= \frac{4}{3} (5 \sqrt{2} - 7) - 4 \sqrt{2} + 6 = \frac{8 \sqrt{2}}{3} - \frac{10}{3}$

$= a \sqrt{2} + b$

So, on comparing $a = \frac{8}{3}$ and $b = - \frac{10}{3}$

(given)

$∴ a - b = \frac{8}{3} + \frac{10}{3} = 6$

Engineering Preparation

Mathematics 11th

Mathematics 12th

JEE Previous Year Questions

JEE PYQ - Chapterwise

JEE Tutorial Sessions

Medical Preparation

NEET Previous Year Questions

NEET PYQ - Chapterwise

NEET Tutorial Sessions

NCERT Books & Solutions

NCERT Books for JEE

NCERT Books for NEET

NCERT Solutions for JEE

NCERT Solutions for NEET

NCERT Exemplar for JEE

NCERT Exemplar for NEET

NCERT Books for 9th-10th

NCERT Exemplar for 9th-10th

Important Resources

Physics Formulas

Chemistry Formulas

Mathematics Formulas

Useful Articles

Sample Mock Tests

Other Resources

Educational Games

CBSE Board Physics Previous Year Questions Chapterwise

Bank PO Previous Year Questions Chapterwise

For 10th and 12th Board Exams

Syllabus

Test Platform

JEE / Engineering Test

NEET / Medical Test

Sample Mock Test

Mock Test for JEE

Additional problems chapterwise

Mentorship

Mentorship for JEE

Mentorship for NEET

NCERT Exercise Video Solution

NCERT Chapter Video Solution

© 2024 Copyright SATHEE

Privacy Policy

Powered by Prutor@IITK

sathee@iitk.ac.in

Media coverage of SATHEE

goolge

Play Store

goolge

App Store

instagram

youtube

Ask SATHEE

Welcome to SATHEE !
Select from 'Menu' to explore our services, or ask SATHEE to get started. Let's embark on this journey of growth together! 🌐📚🚀🎓

I'm relatively new and can sometimes make mistakes.
If you notice any error, such as an incorrect solution, please use the thumbs down icon to aid my learning.
To begin your journey now, click on "I understand".