Area Question 11
Question 11
- The area (in sq units) of the region bounded by the curve $x^{2}=4 y$ and the straight line $x=4 y-2$ is (a) $\frac{7}{8}$ (b) $\frac{9}{8}$ (c) $\frac{5}{4}$ (d) $\frac{3}{4}$
Show Answer
Solution:
- Given equation of curve is $x^{2}=4 y$, which represent a parabola with vertex $(0,0)$ and it open upward.
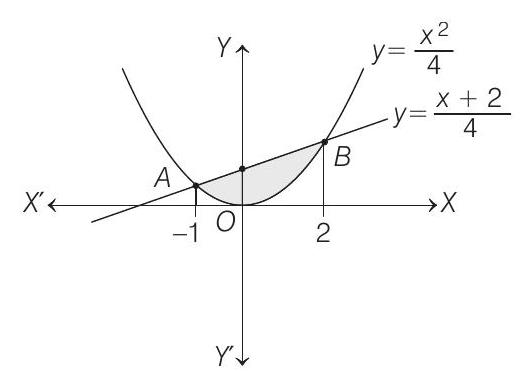
Now, let us find the points of intersection of $x^{2}=4 y$ and $4 y=x+2$
For this consider, $x^{2}=x+2$
$$ \begin{array}{lr} \Rightarrow & x^{2}-x-2=0 \ \Rightarrow & (x-2)(x+1)=0 \ \Rightarrow x=-1, x=2 \end{array} $$
When $x=-1$, then $y=\frac{1}{4}$
and when $x=2$, then $y=1$
Thus, the points of intersection are $A-1, \frac{1}{4}$ and $B(2,1)$.
Now, required area $=$ area of shaded region
$$ \begin{aligned} & =\int_{-1}^{2}{y \text { (line) }-y \text { (parabola) }} d x \ & =\int_{-1}^{2} \frac{x+2}{4}-\frac{x^{2}}{4} d x=\frac{1}{4} \frac{x^{2}}{2}+2 x-{\frac{x^{3}}{3}}_{-1}^{2} \ & =\frac{1}{4} 2+4-\frac{8}{3}-\frac{1}{2}-2+\frac{1}{3} \ & =\frac{1}{4} 8-\frac{1}{2}-3=\frac{1}{4} 5-\frac{1}{2}=\frac{9}{8} \text { sq units. } \end{aligned} $$
