Solution

(i) We have,

$ \begin{aligned} A & =\lbrace x: x \in R, 2 x+11=15\rbrace \\ \therefore 2 x+11 & =15 \\ 2 x & =15-11 \Rightarrow 2 x=4 \\ x & =2 \\ A & =\lbrace 2\rbrace \\ \text{(ii) We have}, B & =\lbrace x \mid x^{2}=x, x \in R\rbrace \\ x^{2} & =x \\ \Rightarrow x^{2}-x & =0 \Rightarrow x(x-1)=0 \\ \Rightarrow x & =0,1 \\ \Rightarrow B & =\lbrace 0,1\rbrace \end{aligned} $

(iii) We have, $C=\lbrace x \mid x$ is a positive factor of prime number $p\rbrace$.

Since, positive factors of a prime number are 1 and the number itself.

$ \therefore \quad C=\lbrace1, p\rbrace $

Solution

(i) We have,

$ \begin{matrix} \text { We have, } & D =\lbrace t \mid t^{3}=t, t \in R\rbrace \\ \therefore & t^{3} =t \\ \Rightarrow & t^{3}-t =0 \Rightarrow t(t^{2}-1)=0 \\ \Rightarrow & t(t-1)(t+1) =0 \Rightarrow t=0,1,-1 \\ \therefore & D =\lbrace-1,0,1\rbrace \\ \end{matrix} $

$ \begin{aligned} & \begin{matrix} \therefore & t^{3}=t \\ \Rightarrow & t^{3}-t=0 \Rightarrow t(t^{2}-1)=0 \end{matrix} \\ & \therefore \quad D=\lbrace-1,0,1\rbrace \end{aligned} $

(ii)

$ \begin{aligned} & \text { We have, } E =\lbrace w \lvert, \frac{w-2}{w+3}=3., w \in R\rbrace \\ & \therefore \quad \frac{w-2}{w+3}=3 \\ & \Rightarrow \quad w-2=3 w+9 \Rightarrow w-3 w=9+2 \\ & \Rightarrow \quad-2 w=11 \quad \Rightarrow \quad w=\frac{-11}{2} \\ & \therefore \quad E=\lbrace \frac{-11}{2} \rbrace \end{aligned} $

(iii) We have,

$ F=\lbrace x \mid x^{4}-5 x^{2}+6=0, x \in R\rbrace $

$ \therefore x^{4}-5 x^{2}+6 =0 $

$ \Rightarrow x^{4}-3 x^{2}-2 x^{2}+6 =0 $

$ \Rightarrow x^{2}(x^{2}-3)-2(x^{2}-3) =0 $

$ \Rightarrow (x^{2}-3)(x^{2}-2) =0 $

$ \Rightarrow x = \pm \sqrt{3}, \pm \sqrt{2} $

$ \therefore F =\lbrace -\sqrt{3},-\sqrt{2}, \sqrt{2}, \sqrt{3}\rbrace $

Note in roaster form, the order in which elements are listed is immaterial. Thus, we can also write $F=\lbrace-\sqrt{3}, \sqrt{2},-\sqrt{2}, \sqrt{3}\rbrace$.

Solution

$Y=\lbrace x \mid x.$ is a positive factor of the number $2^{p-1}(2^{p}-1)$, where $2^{p}-1$ is a prime number $\rbrace$. So, the factor of $2^{p-1}$ are $1,2,2^{2}, 2^{3}, \ldots, 2^{p-1}$.

$ \therefore \quad Y=\lbrace1,2,2^{2}, 2^{3}, \ldots, 2^{p-1}, 2^{p}-1\rbrace $

Solution

(i) Since, the factors of 35 are $1,5,7$ and 35 . So, statement (i) is true.

(ii) Since, the factors of 128 are 1, 2, 4, 8, 16, 32, 64 and 128.

$ \begin{aligned} \therefore \quad \text { Sum of factors } & =1+2+4+8+16+32+64+128 \\ & =255 \neq 2 \times 128 \end{aligned} $

So, statement (ii) is false. (iii) We have,

$ x^{4}-5 x^{3}+2 x^{2}-112 x+6=0 $

$\therefore$ For $x=3$,

$\Rightarrow \quad 81-135+18-336+6=0$

$\Rightarrow$ $ -346=0 $

which is not true.

Hence, statement (iii) is true.

(iv) $\because$ $ 496=2^{4} \times 31 $

So, the factors of 496 are $1,2,4,8,16,31,62,124,248$ and 496.

$\therefore \quad$ Sum of factors $=1+2+4+8+16+31+62+124+248+496$

$ =992=2(496) $

So, $496 \in\lbrace y \mid$ the sum of all the positive factor of $y$ is $2 y\rbrace$.

Hence, statement (iv) is false.

Solution

Given, $\quad L=\lbrace1,2,3,4\rbrace, M=\lbrace3,4,5,6\rbrace$ and $N=\lbrace1,3,5\rbrace$

$\therefore \quad M \cup N=\lbrace1,3,4,5,6\rbrace$

$ L-(M \cup N)=\lbrace2\rbrace $

Now,

$ L-M=\lbrace1,2\rbrace, L-N=\lbrace2,4\rbrace $

$\therefore \quad(L-M) \cap(L-N)=\lbrace2\rbrace$

Hence, $\quad L-(M \cup N)=(L-M) \cap(L-N)$.

Solution

(i) Let $ x \in A $

$\Rightarrow \quad x \in A$ or $x \in B \Rightarrow x \in A \cup B$

Hence, $\subset A \cup B$

(ii) If

$ \begin{matrix} A \subset B \\ x \in A \cup B \end{matrix} $

Let

$\Rightarrow$ $ x \in A \text { or } x \in B \Rightarrow x \in B $

$\Rightarrow$ $A \cup B \subset B$

But $B \subset A \cup B$

From Eqs. (i) and (ii),

$\Rightarrow A \cup B = B$

If $\quad A \cup B = B$

Let $\quad y \in A$

$\Rightarrow \quad y \in A \cup B \Rightarrow y \in B$

$\Rightarrow A \subset B$

Hence, $A \subset B \Rightarrow A \cup B = B$

(iii) Let $\Rightarrow x \in A \cap B$

$\Rightarrow x \in A \text{and} x \in B \Rightarrow x \in A$

Hence, $A \cap B \subset A$

Solution

We have,

$ N=\lbrace1,2,3,4, \ldots, 100\rbrace $

(i) Required subset $=\lbrace2,4,6,8, \ldots, 100\rbrace$

(ii) Required subset $=\lbrace1,4,9,16,25,36,49,64,81,100\rbrace$

Solution

Given,

$ X=\lbrace1,2,3\rbrace $

(i) $\lbrace4 n \mid n \in X\rbrace=\lbrace4,8,12\rbrace$

(ii) $\lbrace n+6 \mid n \in X\rbrace=\lbrace7,8,9\rbrace$

(iii) $\frac{n}{2} \lvert, n \in X=\frac{1}{2}., 1, \frac{3}{2}$

(iv) $\lbrace n-1 \mid n \in X\rbrace=\lbrace0,1,2\rbrace$

Solution

Given,

$ Y=\lbrace 1,2,3, \ldots, 10\rbrace $

(i) $\lbrace a: a \in Y.$ and $.a^{2} \notin Y\rbrace=\lbrace4,5,6,7,8,9,10\rbrace$

(ii) $\lbrace a: a+1=6, a \in Y\rbrace=\lbrace5\rbrace$

(iii) is less than 6 and $a \in Y=\lbrace1,2,3,4,5\rbrace$,

Solution

Solution

Solution

Let $\quad x \in(A-B) \cap(A-C)$

$\Rightarrow \quad x \in(A-B)$ and $x \in(A-C)$

$\Rightarrow \quad(x \in A$ and $x \notin B)$ and $(x \in A$ and $x \notin C)$

$\Rightarrow \quad x \in A$ and $(x \notin B$ and $x \notin C)$

$\Rightarrow \quad x \in A$ and $x \notin(B \cup C)$

$\Rightarrow \quad x \in A-(B \cup C)$

$\Rightarrow \quad(A-B) \cap(A-C) \subset A-(B \cup C)$

Now, let $\quad y \in A-(B \cup C)$

$\Rightarrow$ $y \in A$ and $y \notin(B \cup C)$

$\Rightarrow \quad y \in A$ and $(y \notin B$ and $y \notin C)$

$\Rightarrow \quad(y \in A$ and $y \notin B)$ and $(y \in A$ and $y \notin C)$

$\Rightarrow \quad y \in(A-B)$ and $y \in(A-C)$

$\Rightarrow \quad y \in(A-B) \cap(A-C)$

$\Rightarrow \quad A-(B \cup C) \subset(A-B) \cap(A-C)$

From Eqs. (i) and (ii),

$A-(B \cup C)=(A-B) \cap(A-C)$

Solution

$ \begin{aligned} LHS & =(A-B) \cup(A \cap B) \\ & =[(A-B) \cup A] \cap[(A-B) \cup B] \\ & =A \cap(A \cup B)=A=RHS \end{aligned} $

Hence, given statement is true.

Solution

See the Venn diagrams given below, where shaded portions are representing $A-(B-C)$ and $(A-B)-C$ respectively.

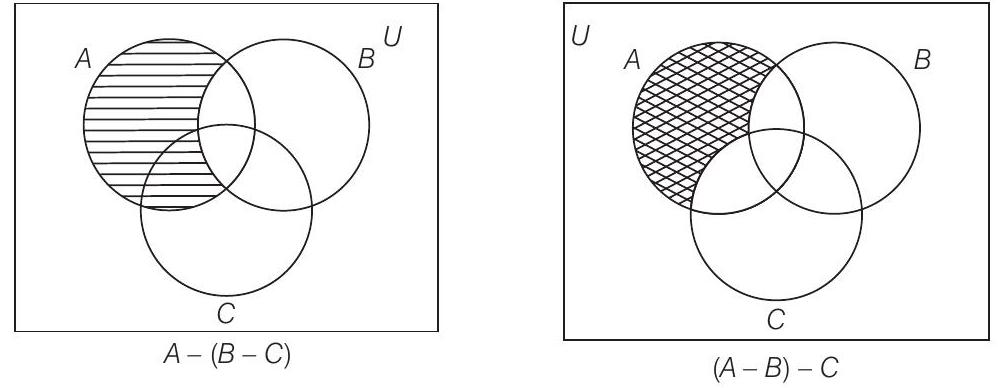
Clearly, $A-(B-C)\neq (A-B)-C$

Hence, given statement is false.

Solution

Let

$x \in A \cap C$

$\Rightarrow \quad x \in A$ and $x \in C$

$\Rightarrow \quad x \in B$ and $x \in C \quad [\because A \subset B]$

$\Rightarrow \quad x \in(B \cap C) \Rightarrow(A \cap C) \subset(B \cap C)$

Hence, given statement is true.

Solution

Let

$x \in A \cup C$

$\Rightarrow$ $x \in A$ and $x \in C$

$\Rightarrow \quad x \in B$ and $x \in C \quad[\because A \subset B]$

$\Rightarrow \quad x \in B \cup C \Rightarrow A \cup C \subset B \cup C$

Hence, given statement is true.

Solution

Let $x \in A \cup B$

$\Rightarrow$ $x \in A$ and $x \in B$

$\Rightarrow \quad x \in C$ and $x \in C\quad$ $[\because A \subset C$ and $B \subset C]$

$\Rightarrow$ $x \in C \Rightarrow A \cup B \subset C$

Hence, given statement is true.

Solution

$LHS=A \cup(B-A)=A \cup(B \cap A^{\prime})$

$[\because A-B=A \cap B^{\prime}]$

$ \begin{matrix} =(A \cup B) \cap(A \cup A^{\prime})=(A \cup B) \cap U & {[\because A \cup A^{\prime}=\cup]} \\ =A \cup B=R H S & {[\because A \cap U=A]} \end{matrix} $

Solution

$ \begin{aligned} LHS & =A-(A-B)=A-(A \cap B^{\prime}) \\ & =A \cap(A \cap B^{\prime})^{\prime}=A \cap[A^{\prime} \cup(B^{\prime})^{\prime}] \\ & =A \cap(A^{\prime} \cup B) \\ & =(A \cap A^{\prime}) \cup(A \cap B)=\varphi \cup(A \cap B) \\ & =A \cap B=RHS \end{aligned} $

Solution

$ \begin{aligned} LHS & =A-(A \cap B)=A \cap(A \cap B)^{\prime} [\because A-B=A \cap B^{\prime}]\\ & =A \cap(A^{\prime} \cup B^{\prime}) [\because (A \cap B)^{\prime}=A^{\prime} \cup B^{\prime}] \\ & =(A \cap A^{\prime}) \cup(A \cap B^{\prime})=\phi \cup(A \cap B^{\prime}) [\because(A^{\prime})^{\prime}=A]\\ & =A \cap B^{\prime} \\ & =A-B=RHS \end{aligned} $

Solution

$ \begin{aligned} LHS & =(A \cup B)-B=(A \cup B) \cap B^{\prime}\quad [\because A-B=A \cap B^{\prime}] \\ & =(A \cap B^{\prime}) \cup(B \cap B^{\prime})=(A \cap B^{\prime}) \cup \phi \quad [\because B \cap B^{\prime}=\phi] \\ & =A \cap B^{\prime} \quad [\because A \cup \phi=A] \\ & =A-B=RHS \end{aligned} $

Solution

Since

$ \begin{aligned} T=\lbrace x \lvert, \frac{x+5}{x-7}-5. & =\frac{4 x-40}{13-x} \rbrace\\ \because \frac{x+5}{x-7}-5 & =\frac{4 x-40}{13-x} \end{aligned} $

$ \begin{aligned} & \Rightarrow \quad \frac{x+5-5(x-7)}{x-7}=\frac{4 x-40}{13-x} \\ & \Rightarrow \quad \frac{x+5-5 x+35}{x-7}=\frac{4 x-40}{13-x} \\ & \Rightarrow \quad \frac{-4 x+40}{x-7}=\frac{4 x-40}{13-x} \\ & \Rightarrow \quad-(4 x-40)(13-x)=(4 x-40)(x-7) \\ & \Rightarrow \quad(4 x-40)(x-7)+(4 x-40)(13-x)=0 \\ & \Rightarrow \quad(4 x-40)(x-7+13-x)=0 \\ & \Rightarrow \quad 4(x-10) 6=0 \\ & \Rightarrow \quad 24(x-10)=0 \\ & \Rightarrow \quad x=10 \\ & \therefore \quad T=\lbrace10\rbrace \end{aligned} $

Hence, $T$ is not an empty set.

Solution

Let

$\Rightarrow x \in A \cap (B \cup C)$

$\Rightarrow x \in A$ and $x \in(B \cup C)$

$\Rightarrow x \in A$ and $(x \in B$ or $x \in C)$

$\Rightarrow (x \in A$ and $x \in B)$ or $(x \in A$ and $x \in C)$

$\Rightarrow x \in A \cap B$ or $x \in A \cap C$

$x \in(A \cap B) \cup(A \cap C)$

$\Rightarrow A \cap(B \cup C) \subset(A \cap B) \cup(A \cap C)$

Again, let

$\Rightarrow y \in(A \cap B) \cup(A \cap C)$

$\Rightarrow y \in(A \cap B)$ or $y \in(A \cap C)$

$\Rightarrow (y \in A$ and $y \in B)$ or $(y \in A$ and $y \in C)$

$\Rightarrow y \in A$ and $(y \in B$ or $y \in C)$

$\Rightarrow y \in A$ and $y \in B \cup C$

$\Rightarrow y \in A \cap(B \cup C)$

$\Rightarrow (A \cap B) \cup(A \cap C) \subset A \cap(B \cup C)$

From Eqs. (i) and (ii)

$A \cap(B \cup C)=(A \cap B) \cup(A \cap C)$

Solution

Let $M$ be the set of students who passed in Mathematics, $E$ be the set of students who passed in English and $S$ be the set of students who passed in Science.

Then, $ n(u)=100 $

$ \begin{aligned} n(E)=15, n(M) & =12, n(S)=8, n(E \cap M)=6, n(M \cap S)=7 \\ n(E \cap S) & =4, \text { and } n(E \cap M \cap S)=4 \end{aligned} $

$ \because \quad n(E)=15 $

$\Rightarrow a+b+e+f = 15$

and $n(M)=12$

$\Rightarrow b+c+e+d=12$

On substituting the values of $d, e$ and $f$ in Eq. (iii), we get

$ 3+4+0+g=8 $

$\Rightarrow \quad g=1$

On substituting the value of $b, e$ and $d$ in Eq. (ii), we get

$ 2+c+4+3=12 $

$\Rightarrow c=13$

On substituting $b, e$, and $f$ in Eq. (i), we get

$ a+2+4+0=15 $

$\Rightarrow$ $ a=9 $

(i) Number of students who passed in English and Mathematics but not in Science

$ =b=2 $

(ii) Number of students who passed in Mathematics and Science but not in English

$ =d=3 $

(iii) Number of students who passed in Mathematics only $=c=3$

(iv) Number of students who passed in more than one subject

$ \begin{aligned} & =b+e+d+f \\ & =2+4+3+0=9 \end{aligned} $

Alternate Method

Let $E$ denotes the set of student who passed in English. $M$ denotes the set of students who passed in Mathematics. $S$ denotes the set of students who passed in Science.

Now,

$ \begin{aligned} n(U) & =100, n(E)=15, n(m)=12, n(S)=8 \\ n(E \cap M) & =6, n(M \cap S)=7 \\ n(E \cap S) & =4, n(E \cap M \cap S)=4 \end{aligned} $

(i) Number of students passed in English and Mathematics but not in Science

$ \text { i.e., } \quad \begin{aligned} n(E \cap M \cap S^{\prime}) & =n(E \cap M)-n(E \cap M \cap S) \quad[\because A \cap B^{\prime}=A-(A \cap B)] \\ & =6-4=2 \end{aligned} $

(ii) Number of students passed in Mathematics and Science but not in English.

$ \text { i.e., } \quad n(M \cap S \cap E^{\prime})=n(M \cap S)-n(M \cap S \cap E) $

$ =7-4=3 $

(iii) Number of students passed in mathematics only

$ \text { i.e., } \quad \begin{aligned} n(M \cap S^{\prime} \cap E^{\prime}) & =n(M)-n(M \cap S)-n(M \cap E)+n(M \cap S \cap E) \\ & =12-7-6+4=3 \end{aligned} $

(iv) Number of students passed in more than one subject only

$ \text { i.e., } \quad \begin{aligned} n(E \cap M)+n(M & \cap S)+n(E \cap S)-3 n(E \cap M \cap S)+n(E \cap M \cap S) \\ & =6+7+4-4 \times 3+4 \\ & =17-12+4=5+4=9 \end{aligned} $

Solution

Let $C$ be the set of students who play cricket and $T$ be the set of students who play tennis. Then

$ n(U)=60, n(C)=25, n(T)=20 \text {, and } n(C \cap T)=10 $

$\therefore$ $ \begin{aligned} n(C \cup T) & =n(C)+n(T)-n(C \cap T) \\ & =25+20-10=35 \end{aligned} $

$\therefore \quad$ Number of students who play neither $=n(U)-n(C \cup T)$

$ =60-35=25 $

Solution

Let $M$ be the set of students who study Mathematics, $P$ be the set of students who study Physics and $C$ be the set of students who study Chemistry.

Then, $n(U)=200,n(M)=120,n(P)=90$

$ \begin{matrix} n(C) =70, n(M \cap P)=40, n(P \cap C)=30, \\ \\ n(C \cap M) =50, n(M^{\prime} \cap P^{\prime} \cap C^{\prime})=20, \\ \\ n(U)-n(M \cup P \cup C) =20, \\ \\ \because n(M \cup P \cup C) =200-20=180 \\ \\ \Rightarrow n(M \cup P \cup C) =n(M)+n(P)+n(C)-n(C \cap M)+n(M \cap P \cap C) \\ \\ \Rightarrow 180 =120+90+70-40-30-50+n(M \cap P \cap C) \\ \\ \Rightarrow n(M \cap P \cap C) =180-160=20 \end{matrix} $

So, the number of students who study all the three subjects is 20 .

Solution

Let $A$ be the set of families which buy newspaper $A, B$ be the set of families which buy newspaper $B$ and $C$ be the set of families which buy newspaper $C$.

Then,

$ n(U) =10000, n(A)=40$% n(B)=20% and n(C)=10%

$ n(A \cap B) =5$ %

$ n(B \cap C) =3 $%

$ n(A \cap C) =4 $ %

$ n(A \cap B \cap C) =2 $%

(i) Number of families which buy newspaper $A$ only

$ =n(A)-n(A \cap B)-n(A \cap C)+n(A \cap B \cap C) $

=(40-5-4+2)%=33 %

$ 10000 \times 33 / 100 =3300 $

(ii) Number of families which buy none of $A, B$ and $C$

$ =n(U)-n(A \cup B \cup C) $

$ =n(U)-[n(A)+n(B)+n(C)-n(A \cap B)-n(B \cap C) $

=100-[40+20+10-5-3-4+2] =100-60 %=40 %

$ =10000 \times \frac{40}{100}=4000 $

Solution

Let $F$ be the set of students who study French, $E$ be the set of students who study English and $S$ be the set of students who study Sanskrit.

Then,

$ \begin{aligned} n(U)=50, n(F) & =17, n(E)=13, \text { and } n(S)=15, \\ n(F \cap E) & =9, n(E \cap S)=4, n(F \cap S)=5, \\ n(F \cap E \cap S) & =3 \end{aligned} $

$ \because \quad n(F)=17 $

$ \begin{matrix} \Rightarrow & a+b+e+f =17 \\ \Rightarrow & n(E) =13 \\ \Rightarrow & b+c+d+e =13 \\ \Rightarrow & n(S) =15 \\ \Rightarrow & d+e+f+g =15 \\ \Rightarrow & n(F \cap E) =9 \\ & b+e =9 \\ & n(E \cap S) =4 \end{matrix} $

$ \begin{aligned} \Rightarrow & e+d =4 \\ \Rightarrow & n(F \cap S) =5 \\ \Rightarrow & n(F \cap E \cap S) =3 \\ \Rightarrow & e =3 \end{aligned} $

From Eqs. (vi) and (vii), $f=2$

From Eqs. (v)and (vii), $d=1$

From Eqs. (iv) and (vii), $b=6$

On substituting the values of $e, f$ and $d$ in Eq. (iii), we get

$ \begin{aligned} 1+3+2+g & =15 \\ \Rightarrow g & =9 \end{aligned} $

On substituting the values of $b, d$ ande in E

(ii), we get

$ \begin{aligned} 6+c+1+3 & =13 \\ c & =3 \end{aligned} $

On substituting the values of $b, e$ and $f$ in E

(i), we get

$ a+6+3+2=17 $

$ \Rightarrow \quad a=6 $

(i) Number of students who study French only, $a=6$

(ii) Number of students who study English only, $c=3$

(iii) Number of students who study Sanskrit only, $g=9$

(iv) Number of students who study English and Sanskrit but not French, $d=1$

(v) Number of students who study French and Sanskrit but not English, $f=2$

(vi) Number of students who study French and English but not Sanskrit, $b=6$

(vii) Number of students who study atleast one of the three languages

$ \begin{aligned} & =a+b+c+d+e+f+g \\ & =6+6+3+1+3+2+9=30 \end{aligned} $

(viii) Number of students who study none of three languages $=$ Total students - Students who study atleast one of the three languages

$ =50-30=20 $

Solution

(c) If elements are not repeated, then number of elements in $A_1 \cup A_2 \cup A_3, \ldots \cup A_{30}$ is $30 \times 5$.

But each element is used 10 times, so

$ S=\frac{30 \times 5}{10}=15 $

If elements in $B_1, B_2, \ldots, B_{n}$ are not repeated, then total number of elements is $3 n$ but each element is repeated 9 times, so

$ \begin{aligned} & S=\frac{3 n}{9} \Rightarrow 15=\frac{3 n}{9} \\ \therefore \quad & n=45 \end{aligned} $

Solution

(a) Since, number of subsets of a set containing melements is 112 more than the subsets of the set containing $n$ elements.

$ \because 2^{m}-2^{n} =112 $

$ \Rightarrow 2^{n} \cdot(2^{m-n}-1) =2^{4} \cdot 7 $

$ \Rightarrow 2^{n}=2^{4} \text { and } 2^{m-n}-1 =7 $

$ \Rightarrow n=4 \text { and } 2^{m-n} =8 $

$ \Rightarrow 2^{m-n} =2^{3} \Rightarrow m-n=3 $

$ \Rightarrow m-4 =3 \Rightarrow m=4+3 $

$ \therefore m =7 $

Solution

(b) We know that, $(A \cap B)^{\prime}=(A^{\prime} \cup B^{\prime})$ and $(A^{\prime})^{\prime}=A$

$ \begin{aligned} \therefore \quad & =(A \cap B^{\prime})^{\prime} \cup(B \cap C) \\ & =[A^{\prime} \cup(B^{\prime})^{\prime}] \cup(B \cap C) \\ & =(A^{\prime} \cup B) \cup(B \cap C)=A^{\prime} \cup B \end{aligned} $

Solution

(d) Every rectangle, rhombus, square in a plane is a parallelogram but every trapezium is not a parallelogram.

So, $F_1$ is either of $F_1, F_2, F_3$ and $F_4$.

$ \therefore \quad F_1=F_2 \cup F_3 \cup F_4 \cup F_1 $

Solution

(c) The given sets can be represented in Venn diagram as shown below

It is clear from the diagram that, $S \cup T \cup C=S$.

Solution

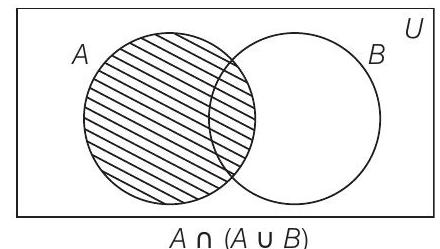
(d) Since, $R$ be the set of points inside the rectangle.

$\therefore R=\lbrace(x, y): 0<x<a$ and $0<y<b\rbrace$

Solution

(b) Let $H$ be the set of persons who read Hindi and $E$ be the set of persons who read English.

Then, $n(U)=840, n(H)=450, n(E)=300, n(H \cap E)=200$

Number of persons who read neither $=n(H^{\prime} \cap F^{\prime})$

$=n(H \cup E)^{\prime}$

$=n(U)-n(H \cup E)$

$=840-[n(H)+n(E)-n(H \cap E)]$

$=840-(450+300-200)$

$=840-550=290$

Solution

(a)

$ \begin{aligned} & X=\lbrace8^{n}-7 n-1 \mid n \in N\rbrace=\lbrace0,49,490, \ldots\rbrace \\ & Y=\lbrace49 n-49 \mid n \in N\rbrace=\lbrace0,49,98,147, \ldots\rbrace \end{aligned} $

Clearly, every elements of $X$ is in $Y$ but every element of $Y$ is not in $X$.

$ \therefore \quad X \subset Y $

Solution

(c) Let $A$ be the set of percentage of those people who watch a news channel and $B$ be the set of percentage of those people who watch another channel.

$ n(A)=63, n(B) =76, \text { and } n(A \cap B)=x $

$ \because n(A \cup B) \leq 100 $

$ \Rightarrow n(A)+n(B)-n(A \cap B) \leq 100 $

$ \Rightarrow 63+76-x \leq 100 \Rightarrow 139-x \leq 100 $

$ \Rightarrow 139-100 \leq x \Rightarrow 39 \leq x $

$ \Rightarrow n(A) =63 $

$ \therefore x(A \cap B) \leq n(A) \Rightarrow x \leq 63 39 \leq x \leq 63 $

Solution

(c) Let $x \in R$

$ \begin{matrix} \text { We know that, } & -x \neq \frac{1}{x} \\ \therefore & A \cap B=\phi \end{matrix} $

Solution

(a) $\because$ $ A \cap(A \cup B)=A $

Solution

(b)

$ \begin{matrix} A^{\prime} \cup[(A \cup B) \cap B] \quad[\because A \cap(B \cup C)=(A \cap B) \cup(A \cap C)] \\ =A^{\prime} \cup[(A \cap B^{\prime}) \cup(B \cap B^{\prime})] \\ =A^{\prime} \cup[(A \cap B^{\prime}) \cup \phi]=A^{\prime} \cup(A \cap B^{\prime}) \\ =(A^{\prime} \cup A) \cap(A^{\prime} \cup B^{\prime}) \\ =A^{\prime} \cup B^{\prime}=N \cap(A^{\prime} \cup B^{\prime}) \\ =A^{\prime} \cup B^{\prime}=(A \cap B)^{\prime} & \\ =\phi=N & {[\because A \cap B=\phi]} \end{matrix} $

Solution

(d) $\because \quad S=\lbrace x \mid x$ is a positive multiple of 3 less than 100$\rbrace$

$ \therefore \quad n(S)=33 $

and $P=\lbrace x \mid x$ is a prime number less than 20$\rbrace$

$ \begin{aligned} n(P) & =8 \\ n(S)+n(P) & =33+8=41 \end{aligned} $

Solution

(c)

$ \begin{aligned} X \cap(X \cup Y)^{\prime} & =X \cap(X^{\prime} \cap Y^{\prime}) \\ & =(X \cap X^{\prime}) \cap(X \cap Y^{\prime}) \\ & =\phi \cap(X \cap Y^{\prime})=\phi \end{aligned} $

$ [\because(A \cup B)^{\prime}=A^{\prime} \cap B^{\prime}] $

$[\because \phi \cap A=\phi]$

Solution

The set $\lbrace x \in R: 1 \leq x<2\rbrace$ can be written as $(1,2)$.

Solution

$\therefore$ $ \begin{aligned} A & =\phi \Rightarrow n(A)=0 \\ n\lbrace P(A)\rbrace & =2^{n(A)}=2^{0}=1 \end{aligned} $

So, number of element in $P(A)$ is 1 .

Solution

If $A$ and $B$ are two finite sets such that $A \subset B$, then $n(A \cup B)=n(B)$.

Solution

If $A$ and $B$ are any two sets, then $A-B=A \cap B^{`}$

Solution

$\therefore A=\lbrace1,2\rbrace$

So, the subsets of $A$ are $\phi\lbrace1\rbrace,\lbrace2\rbrace$ and $\lbrace1,2\rbrace$.

$\therefore \quad P(A)=\lbrace\phi,\lbrace1\rbrace,\lbrace2\rbrace,\lbrace1,2\rbrace\rbrace$

Solution

Universal set for $A, B$ and $C$ is given by $U=\lbrace0,1,2,3,4,5,6,8\rbrace$

Solution

If

$U=\lbrace1,2,3,4,5, \ldots, 10\rbrace$,

$A=\lbrace1,2,3,5\rbrace, B=\lbrace2,4,6,7\rbrace$ and $C=\lbrace2,3,4,8\rbrace$

$\therefore \quad B \cup C=\lbrace2,3,4,6,7,8\rbrace$

(i) $(B \cup C)^{\prime}=U-(B \cup C)=\lbrace1,5,9,10\rbrace$

(ii) $C-A=\lbrace4,8\rbrace$

$\therefore \quad(C-A)^{\prime}=U-(C-A)=\lbrace1,2,3,5,6,7,9,10\rbrace$

Solution

$A-(A \cap B)=A-B=A \cap B^{\prime}$

Solution

(i) $[(A^{\prime} \cup B^{\prime})-A]^{\prime}=[(A^{\prime} \cup B^{\prime}) \cap A^{\prime}]^{\prime}$

$[\because A-B=A \cap B^{\prime}]$

$=[(A \cap B)^{\prime} \cap A^{\prime}]^{\prime}$ $[\because(A \cap B)^{\prime}=A^{\prime} \cup B^{\prime}]$

$=[(A \cap B)^{\prime}]^{\prime} \cup(A^{\prime})^{\prime}=(A \cap B) \cup A$

$=A$

(ii) $[B^{\prime} \cup(B^{\prime}-A)]^{\prime}=[B^{\prime} \cup(B^{\prime} \cap A^{\prime})]^{\prime}$

$[\because A-B=A \cap B^{\prime}]$

$=B^{\prime} \cup(B \cup A^{\prime}]^{\prime}.$

$[\because A^{\prime} \cap B^{\prime}=(A \cup B)^{\prime}]$

$=(B^{\prime})^{\prime} \cap[(B \cup A)^{\prime}]^{\prime}$ $[\because(A \cup B)^{\prime}=A^{\prime} \cap B^{\prime}]$

$=B \cap(B \cup A)$

$[\because(A^{\prime})^{\prime}=A]$

$=B$

(iii) $(A-B)-(B-C)=(A \cap B^{\prime})-(B \cap C^{\prime})$

$=(A \cap B^{\prime}) \cap(B \cap C^{\prime})^{\prime}$

$=(A \cap B^{\prime}) \cap[B^{\prime} \cup(C^{\prime})^{\prime}]$

$=(A \cap B^{\prime}) \cap(B^{\prime} \cup C)$

$=[A \cap(B^{\prime} \cup C)] \cap[B^{\prime} \cap(B^{\prime} \cup C)]$

$=[A \cap(B^{\prime} \cup C)] \cap B^{\prime}$

$=(A \cap B^{\prime}) \cap[(B^{\prime} \cup C) \cap B^{\prime}]$

$=(A \cap B^{\prime}) \cap B^{\prime}=A \cap B^{\prime}=A-B$

Alternate Method

It is clear from the diagram, $(A-B)-(B-C)=A-B$.

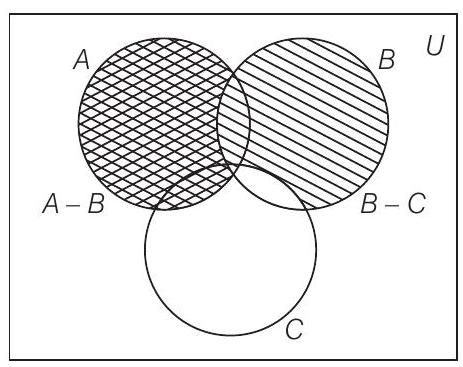
(iv) $(A-B) \cap(C-B)$ $\Rightarrow$ $\Rightarrow$ $(A \cap B^{\prime}) \cap(C \cap B^{\prime})$ $[\because A-B=A \cap B^{\prime}]$ $\Rightarrow \quad(A \cap C)-B$ $[\because A \cap B^{\prime}=A-B]$

(v) $A \times B \cap C=(A \times B) \cap(A \times C)$

(vi) $A \times(B \cup C)=(A \times B) \cup(A \times C)$

Hence, the correct matches are

(i) $\rightarrow$ (b),

(ii) $\rightarrow$ (c),

(iii) $\rightarrow(a)$,

(iv) $\rightarrow$ (f),

(v) $\rightarrow(d)$,

(vi) $\rightarrow(e)$

Solution

True

Since, every set is the subset of itself.

Therefore, for any set $A, A \subset A$.

Solution

False

$ \begin{aligned} & M=\lbrace1,2,3,4,5,6,7,8,9\rbrace \\ & B=\lbrace1,2,3,4,5,6,7,8,9\rbrace \end{aligned} $

Since, every elements of $B$ is also in $M$

$ \therefore \quad B \subset M $

Solution

False

Solution

True

Since, every integer is also a rational number, then $Z \subset Q$

where, $Z$ is the set of integer and $Q$ is the set of rational number.

$\therefore$ $Q \cup Z=Q$

Solution

True

$ \begin{aligned} & R=\lbrace x \in Z \mid x \text { is divisible by } 2\rbrace=\lbrace\ldots-6,-4,-2,0,2,4,6, \ldots\rbrace \\ & T=\lbrace x \in Z \mid x \text { is divisible by } 6\rbrace=\lbrace\ldots,-12,-6,0,6,12, \ldots\rbrace \end{aligned} $

Thus, this every elements of $T$ is also in $R$

$\therefore \quad T \subset R$

Solution

False

$ \begin{aligned} A=\lbrace0,1,2\rbrace, \text { and } B & =\lbrace x \in R \mid 0 \leq x \leq 2\rbrace \\ n(A) & =3 \end{aligned} $

So, $A$ is finite. Since, there are infinite real numbers from 0 to 2 . So, $B$ is infinite.

$\therefore \quad A \neq B$