Work Power and Energy 5 Question 6
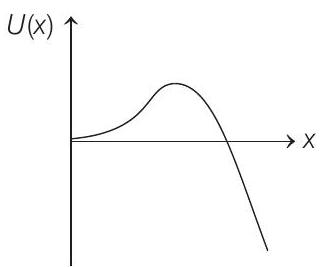
8. A particle, which is constrained to move along $x$-axis, is subjected to a force in the same direction which varies with the distance $x$ of the particle from the origin as $F(x)$ $=-k x+a x^{3}$. Here, $k$ and $a$ are positive constants. For $x \geq 0$, the functional form of the potential energy $U(x)$ of the particle is (a)
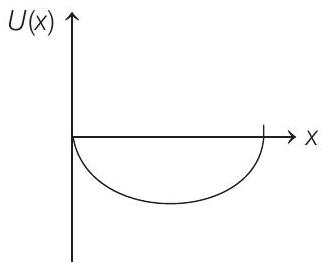
(c)
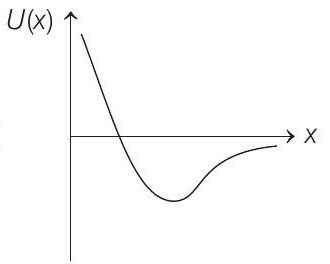
(b)
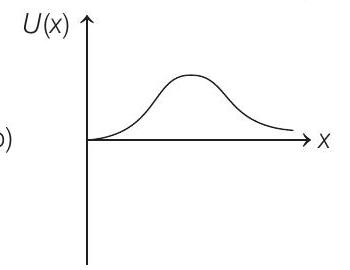
(d)
Show Answer
Answer:
Correct Answer: 8. (d)
Solution:
- $\quad F=-\frac{d U}{d x}$
$$ \therefore \quad d U=-F \cdot d x $$
or $\quad U(x)=-\int _0^{x}\left(-k x+a x^{3}\right) d x$
$$ U(x)=\frac{k x^{2}}{2}-\frac{a x^{4}}{4} $$
$$ \begin{aligned} & U(x)=0 \text { at } x=0 \text { and } x=\sqrt{\frac{2 k}{a}} \\ & U(x)=\text { negative for } x>\sqrt{\frac{2 k}{a}} \end{aligned} $$
From the given function, we can see that $F=0$ at $x=0$ i.e. slope of $U-x$ graph is zero at $x=0$.
