Work Power and Energy 3 Question 1
1. A point particle of mass $m$, moves along the uniformly rough track $P Q R$ as shown in the figure. The coefficient of friction, between the particle and the rough track equals $\mu$. The particle is released from rest, from the point $P$ and it comes to rest at a point $R$. The energies lost by the ball, over the parts $P Q$ and $Q R$ of the track, are equal to each other, and no energy is lost when particle changes direction from $P Q$ to $Q R$. The values of the coefficient of friction $\mu$ and the distance $x$ $(=Q R)$, are respectively close to
(2016 Main)
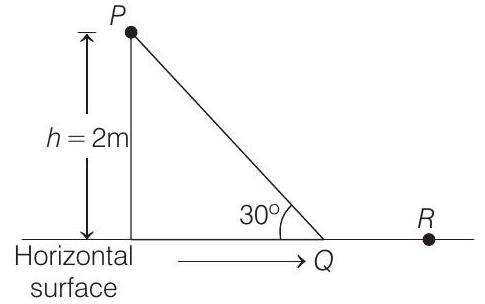
(a) 0.2 and $6.5 m$
(b) 0.2 and $3.5 m$
(c) 0.29 and $3.5 m$
(d) 0.29 and $6.5 m$
The block $B$ is displaced towards wall 1 by a small distance $x$ and released. The block returns and moves a maximum distance $y$ towards wall 2. Displacements $x$ and $y$ are measured with respect to the equilibrium position of the block $B$. The ratio $\frac{y}{x}$ is
$(2008,3 M)$
(a) 4
(b) 2
(c) $\frac{1}{2}$
(d) $\frac{1}{4}$
Show Answer
Answer:
Correct Answer: 1. (c)
Solution:
- As energy loss is same, thus
$$ \begin{array}{rlrl} \mu m g \cos \theta \cdot(P Q) & =\mu m g \cdot(Q R) \\ \therefore \quad & Q R & =(P Q) \cos \theta \\ \Rightarrow \quad & Q R & =4 \times \frac{\sqrt{3}}{2} \\ & =2 \sqrt{3} \approx 3.5 m \end{array} $$
Further,decrease in potential energy $=$ loss due to friction
$\therefore \quad m g h=(\mu m g \cos \theta) d _1+(\mu m g) d _2$
$$ \begin{array}{rlrl} & m \times 10 \times 2 & =\mu \times m \times 10 \times \frac{\sqrt{3}}{2} \times 4 \\ & & +\mu \times m \times 10 \times 2 \sqrt{3} \\ \Rightarrow & 4 \sqrt{3} \mu & =2 \quad \mu & =\frac{1}{2 \sqrt{3}}=0.288=0.29 \end{array} $$
