Wave Motion 4 Question 29
27. A source of sound is moving along a circular path of radius
(1990, 7M)
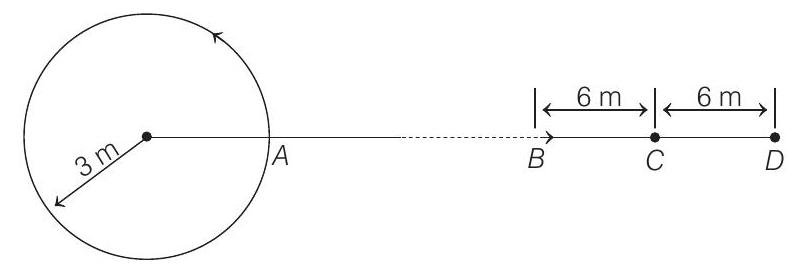
Show Answer
Answer:
Correct Answer: 27. 5
Solution:
- Angular frequency of detector
Since, angular frequency of source of sound and of detector are equal, their time periods will also be equal.
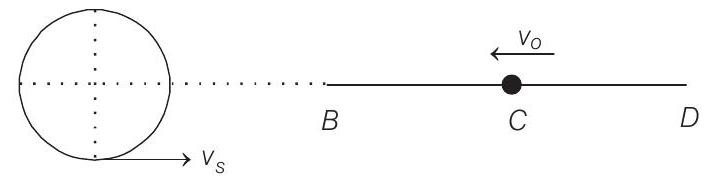
Maximum frequency will be heard in the position shown in figure. Since, the detector is far away from the source, we can use,
Here,
(given)
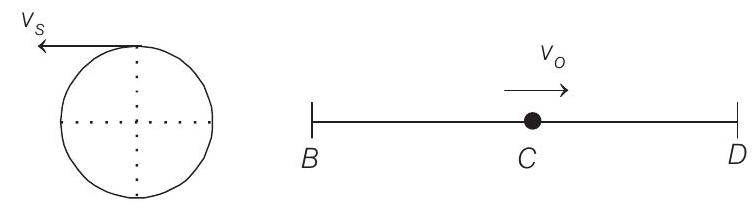
Minimum frequency will be heard in the condition shown in figure. The minimum frequency will be






