Wave Motion 2 Question 5
5. A resonance tube is old and has jagged end. It is still used in the laboratory to determine velocity of sound in air. A tuning fork of frequency
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 5. (a)
Solution:
- Key idea To overcome the error occured in measurement of resonant length. We introduce end correction factor
In first resonance, length of air coloumn
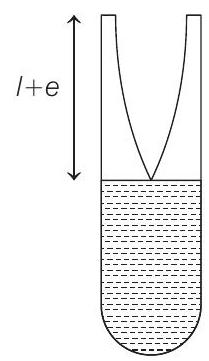
So,
So, speed of sound is
And in second case,
Dividing both Eqs. (i) and (ii), we get
Substituting value of
Speed of sound






