SATHEE: Wave Motion 1 Question 4

Wave Motion 1 Question 4

4. A heavy ball of mass $M$ is suspended from the ceiling of a car by a light string of mass $m (m « M)$ . When the car is at rest, the speed of transverse waves in the string is $60 m s^{- 1}$ . When the car has acceleration $a$ , the wave speed increases to $60.5 m s^{- 1}$ . The value of $a$ , in terms of gravitational acceleration $g$ is closest to

(2019 Main, 9 Jan I)

(a) $\frac{g}{20}$

(b) $\frac{g}{5}$

(d) $\frac{g}{10}$

Show Answer

Answer:

Correct Answer: 4. (b)

Solution:

When the car is at rest, then the situation can be shown in the figure below.

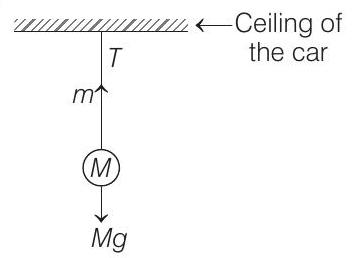
Since, $m « M$ , then we can neglect the mass of the string. So, the initial velocity of the wave in the string can be given as,

$v = \sqrt{\frac{T}{μ}} = \sqrt{\frac{M g}{\frac{M}{l}}} = 60 m / s$

where, $T$ is the tension in the string and $μ$ is the mass of the block per unit length $(l)$ .

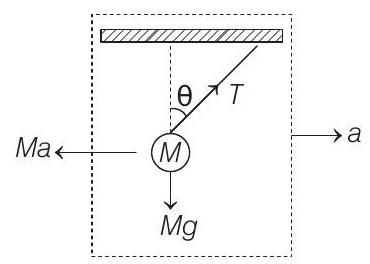
Now, when the car has acceleration ’ $a$ ‘, then the situation can be shown in the figure given below,

Resolving the components of ’ $T$ ’ along $X$ -and $Y$ -axis, we get

$\begin{aligned} T \cos θ & = M g \\ T \sin θ & = M a \end{aligned}$

Squaring both sides of Eqs. (ii) and (iii) and adding them, we get

$\begin{array}{cc} T^{2} (\sin^{2} θ + \cos^{2} θ) = M^{2} (g^{2} + a^{2}) \\ \Rightarrow T = M {(g^{2} + a^{2})}^{\frac{1}{2}} \end{array}$

Now, the velocity of the wave in the string would be given as,

$v^{'} = \sqrt{\frac{T}{μ}} = \sqrt{\frac{M {(g^{2} + a^{2})}^{\frac{1}{2}}}{\frac{M}{l}}} = 60.5 m / s$

Dividing Eq. (i) and Eq. (v), we get

$\frac{v}{v^{'}} = \frac{\sqrt{\frac{\frac{M g}{l}}{l}}}{\sqrt{\frac{M {(g^{2} + a^{2})}^{\frac{1}{2}}}{\frac{M}{l}}}} = \sqrt{\frac{g}{{(g^{2} + a^{2})}^{\frac{1}{2}}}} = \frac{60}{60.5}$

$\frac{60.5}{60} = \sqrt{\frac{{(g^{2} + a^{2})}^{\frac{1}{2}}}{g}}$

Squaring both the sides, we get

$\begin{aligned} \frac{(60.5)^{2}}{(60)^{2}} & = \frac{{(g^{2} + a^{2})}^{\frac{1}{2}}}{g} {(1 + \frac{0.5}{60})}^{2} & = \sqrt{1 + {(\frac{a}{g})}^{2}} \end{aligned}$

Using binomial expansion,

$(1 + x)^{n} = 1 + \frac{n x}{1!} + \dots$

On both sides, we get

$\begin{matrix} 1 + 2 \times \frac{0.5}{60} = 1 + \frac{1}{2} \cdot \frac{a^{2}}{g^{2}} \\ \Rightarrow 1 + \frac{1}{60} = 1 + \frac{1}{2} \cdot \frac{a^{2}}{g^{2}} or \frac{a}{g} = \frac{1}{\sqrt{30}} \\ or a = \frac{g}{\sqrt{30}} = \frac{g}{5.4} ≃ \frac{g}{5} \end{matrix}$