Simple Harmonic Motion 4 Question 13
13. A spring block system is resting on a frictionless floor as shown in the figure. The spring constant is $2.0 Nm^{-1}$ and $1 kg \stackrel{2 m s^{-1}}{\longrightarrow} 2 kg-mmm$ the mass of the block is $2.0 kg$. Ignore the mass of the spring. Initially, the spring is in an unstretched condition. Another block of mass $1.0 kg$ moving with a speed of $2.0 ms^{-1}$ collides elastically with the first block. The collision is such that the $2.0 kg$ block does not hit the wall. The distance, in metres, between the two blocks when the spring returns to its unstretched position for the first time after the collision is
(2018 Adv.)
Analytical & Descriptive Questions
Show Answer
Answer:
Correct Answer: 13. (2.09)
Solution:
- Just Before Collision,
$$ 1 kg \stackrel{2 m / s}{\longrightarrow} \quad 2 kg \text {, } $$
Just After Collision
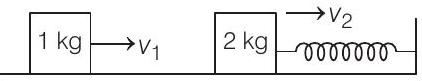
Let velocities of $1 kg$ and $2 kg$ blocks just after collision be $v _1$ and $v _2$ respectively.
From momentum conservation principle,
$$ 1 \times 2=1 v _1+2 v _2 $$
Collision is elastic. Hence $e=1$ or relative velocity of separation $=$ relation velocity of approach.
From Eqs. (i) and (ii),
$$ v _2-v _1=2 $$
$$ v _2=\frac{4}{3} m / s, v _1=\frac{-2}{3} m / s $$
$2 kg$ block will perform SHM after collision,
$$ \begin{gathered} t=\frac{T}{2}=\pi \sqrt{\frac{m}{k}}=3.14 s \\ \text { Distance }=\left|v _1\right| t=\frac{2}{3} \times 3.14=2.093=2.09 m \end{gathered} $$
