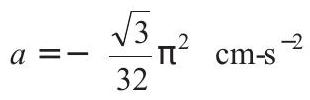Simple Harmonic Motion 2 Question 2
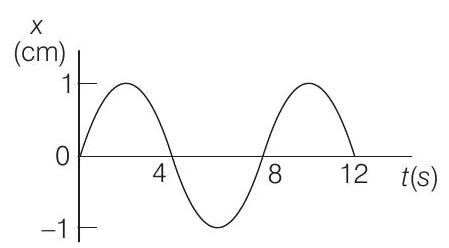
2. The $x$ - $t$ graph of a particle undergoing simple harmonic motion is shown below. The acceleration of the particle at $t=4 / 3 s$ is
(a) $\frac{\sqrt{3}}{32} \pi^{2} cms^{-2}$
(b) $\frac{-\pi^{2}}{32} cms^{-2}$
(c) $\frac{\pi^{2}}{32} cms^{-2}$
(d) $-\frac{\sqrt{3}}{32} \pi^{2} cms^{-2}$
Show Answer
Answer:
Correct Answer: 2. (d)
Solution:
- $T=8 s, \omega=\frac{2 \pi}{T}=\frac{\pi}{4} \operatorname{rads}^{-1}$
$$ \begin{aligned} & \Rightarrow \quad x=A \sin \omega t \\ & \therefore \quad a=-\omega^{2} x=-\frac{\pi^{2}}{16} \sin \frac{\pi}{4} t \end{aligned} $$
Substituting $\quad t=\frac{4}{3} s$, we get
