Rotation 5 Question 30
42. A carpet of mass
Show Answer
Answer:
Correct Answer: 42.
Solution:
- Let
From conservation of mechanical energy :
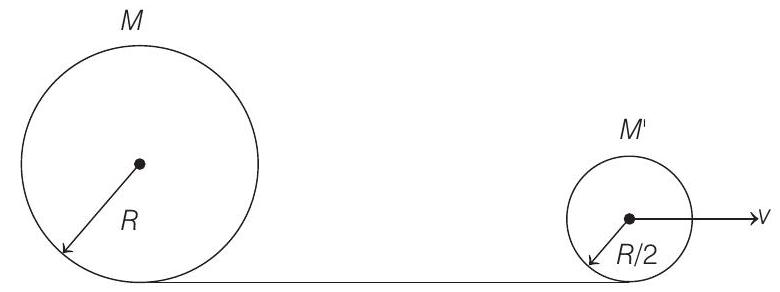
or
or






