Rotation 5 Question 21
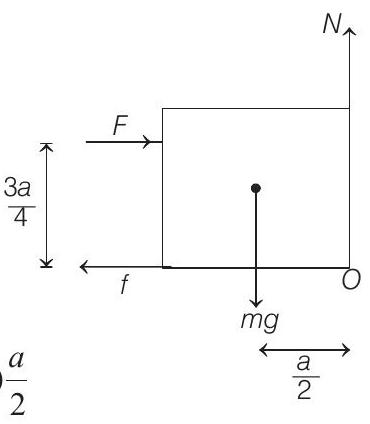
33. A uniform cube of side $a$ and mass $m$ rests on a rough horizontal table. A horizontal force $F$ is applied normal to one of the faces at a point that is directly above the centre of the face, at a height $3 a / 4$ above the base. The minimum value of $F$ for which the cube begins to tip about the edge is (Assume that the cube does not slide).
$(1984,2 M)$
True / False
Show Answer
Answer:
Correct Answer: 33. $\frac{2}{3} m g$
Solution:
- Taking moments about point $O$ Moments of $N$ (normal reaction) and $f$ (force of friction) are zero. In critical case normal reaction will pass through $O$. To tip about the edge, moment of $F$ should be greater than moment of $m g$. Or,
$$ F \quad \frac{3 a}{4}>(m g) \frac{a}{2} $$
$$ \text { or } \quad F>\frac{2}{3} m g $$
