Rotation 5 Question 1
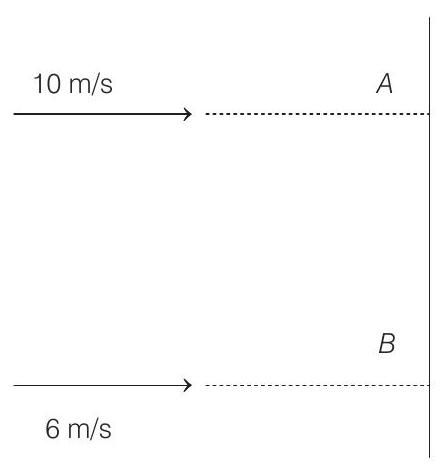
10. A thin uniform bar lies on a frictionless horizontal surface and is free to move in any way on the surface. Its mass is
(1991)
(a)
(c)
(b)
(d)
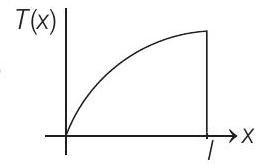
2 A rectangular solid box of length
(a) 0.02
(b) 0.3
(c) 0.5
(2019 Main, 8 April II)
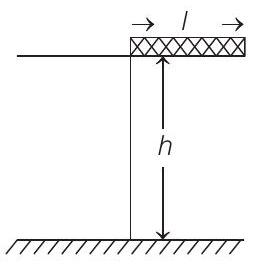
3 A straight rod of length
(2019 Main, 12 Jan I)
(a)
(b) Gm
(c)
(d)
4 The magnitude of torque on a particle of mass
(Main 2019, 11 Jan II)
(a)
(b)
(c)
(d)
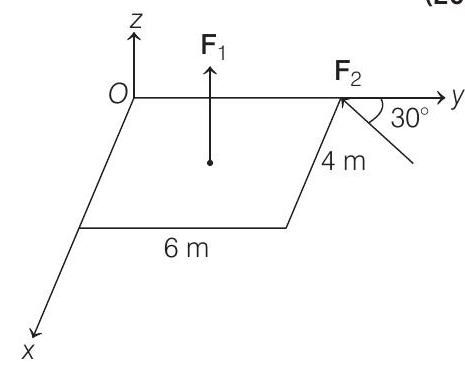
5 A slab is subjected to two forces
(2019 Main, 11 Jan I)
(a)
(b)
(c)
(d)
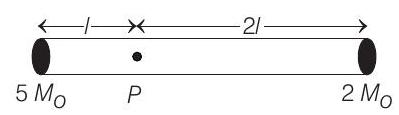
6 A rigid massless rod of length
(2019 Main, 10 Jan II)
(a)
(b)
(c)
(d)
7 To mop-clean a floor, a cleaning machine presses a circular mop of radius
(a)
(b)
(c)
(d)
8 A rod of length
(2019 Main, 09 Jan II)
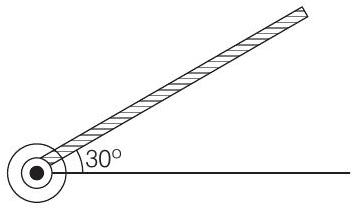
(a)
(b)
(c)
(d)
9 Two masses
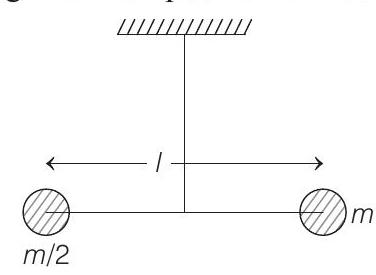
(a)
(b)
(c)
(d)
Show Answer
Answer:
Correct Answer: 10. (a)
Solution:
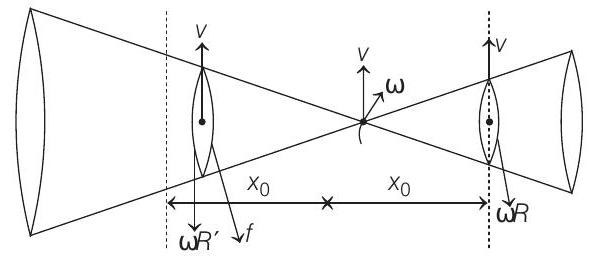
At distance
Distance less than
Initially, there is pure rolling at both the contacts. As the cone moves forward, slipping at
Thus, the cone will start turning towards left. As it moves, further slipping at






