Rotation 4 Question 2
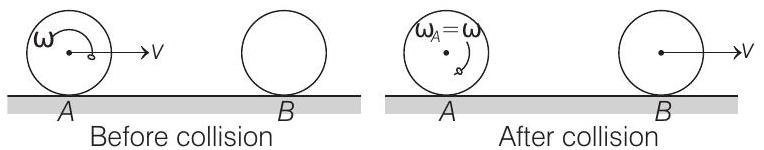
3. A smooth sphere $A$ is moving on a frictionless horizontal plane with angular velocity $\omega$ and centre of mass velocity $v$. It collides elastically and head on with an identical sphere $B$ at rest. Neglect friction everywhere. After the collision their angular speeds are $\omega _A$ and $\omega _B$ respectively. Then,
(1999, 2M)
(a) $\omega _A<\omega _B$
(b) $\omega _A=\omega _B$
(c) $\omega _A=\omega$
(d) $\omega _B=\omega$
Show Answer
Answer:
Correct Answer: 3. (c)
Solution:
- Since, it is head on elastic collision between two identical spheres, they will exchange their linear velocities, i.e., $A$ comes to rest and $B$ starts moving with linear velocity $v$. As there is no friction anywhere, torque on both the spheres about their centre of mass is zero and their angular velocities remain unchanged. Therefore,
$$ \omega _A=\omega \text { and } \omega _B=0 \text {. } $$
