Rotation 3 Question 21
27. A boy is pushing a ring of mass
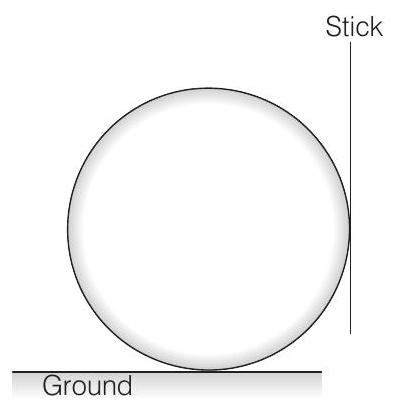coefficient of friction between the stick and the ring is
(2011)
Analytical & Descriptive Questions
Show Answer
Answer:
Correct Answer: 27. 3.6
Solution:
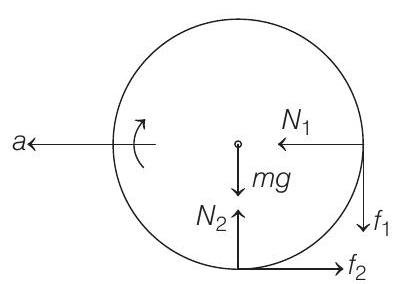
There is no slipping between ring and ground. Hence
Solving above four equations we get,
