Rotation 2 Question 24
24. A particle is projected at time
Show Answer
Answer:
Correct Answer: 24.
Solution:
- In terms of
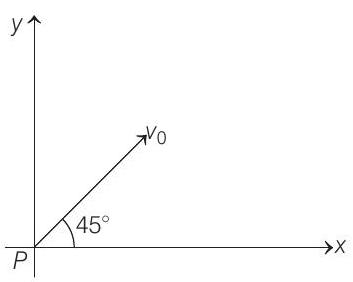
Now, angular momentum about point
Thus, magnitude of angular momentum is






