SATHEE: Rotation 1 Question 13

Rotation 1 Question 13

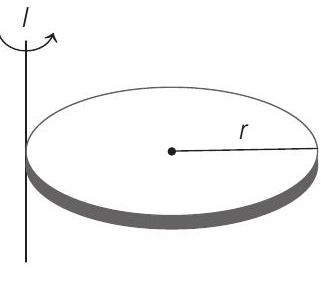16. A solid sphere of radius $R$ has moment of inertia $I$ about its geometrical axis. It is melted into a disc of radius $r$ and thickness $t$ . If it’s moment of inertia about the tangential axis (which is perpendicular to plane of the disc),

is also equal to $I$ , then the value of $r$ is equal to

(a) $\frac{2}{\sqrt{15}} R$

(b) $\frac{2}{\sqrt{5}} R$

(d) $\frac{\sqrt{3}}{\sqrt{15}} R$

Show Answer

Answer:

Correct Answer: 16. (a)

Solution:

$\frac{2}{5} M R^{2} = \frac{1}{2} M r^{2} + M r^{2}$

or $\frac{2}{5} M R^{2} = \frac{3}{2} M r^{2}$

$∴ r = \frac{2}{\sqrt{15}} R$

$\begin{aligned} I & = \frac{m a^{2}}{6} = \frac{\frac{2}{\sqrt{3} π} M \frac{2}{\sqrt{3}} R^{2}}{σ} \\ = \frac{4 M R^{2}}{9 \sqrt{3} π} \end{aligned}$

पिछला

अगला

Rotation 1 Question 13

16. A solid sphere of radius $R$ has moment of inertia $I$ about its geometrical axis. It is melted into a disc of radius $r$ and thickness $t$ . If it’s moment of inertia about the tangential axis (which is perpendicular to plane of the disc),

Answer:

इंजीनियरिंग की तैयारी

चिकित्सा तैयारी

एनसीईआरटी पुस्तकें और समाधान

महत्वपूर्ण संसाधन

अन्य संसाधन

पाठ्यक्रम

परीक्षा मंच

नमूना मॉक टेस्ट

सदस्यता

एनसीईआरटी व्यायाम वीडियो समाधान

Rotation 1 Question 13

16. A solid sphere of radius R has moment of inertia I about its geometrical axis. It is melted into a disc of radius r and thickness t. If it’s moment of inertia about the tangential axis (which is perpendicular to plane of the disc),

Answer:

इंजीनियरिंग की तैयारी

चिकित्सा तैयारी

एनसीईआरटी पुस्तकें और समाधान

महत्वपूर्ण संसाधन

अन्य संसाधन

पाठ्यक्रम

परीक्षा मंच

नमूना मॉक टेस्ट

सदस्यता

एनसीईआरटी व्यायाम वीडियो समाधान

Menu

16. A solid sphere of radius $R$ has moment of inertia $I$ about its geometrical axis. It is melted into a disc of radius $r$ and thickness $t$ . If it’s moment of inertia about the tangential axis (which is perpendicular to plane of the disc),