Optics 7 Question 39
40. The refractive indices of the crown glass for blue and red light are 1.51 and 1.49 respectively and those of the flint glass are 1.77 and 1.73 respectively. An isosceles prism of angle $6^{\circ}$ is made of crown glass. A beam of white light is incident at a small angle on this prism. The other flint glass isosceles prism is combined with the crown glass prism such that there is no deviation of the incident light.
(a) Determine the angle of the flint glass prism.
(b) Calculate the net dispersion of the combined system.
$(2001,5 M)$
Show Answer
Solution:
- (a) When angle of prism is small and angle of incidence is also small, the deviation is given by $\delta=(\mu-1) A$.
Net deviation by the two prisms is zero. So,
$$ \delta _1+\delta _2=0 $$
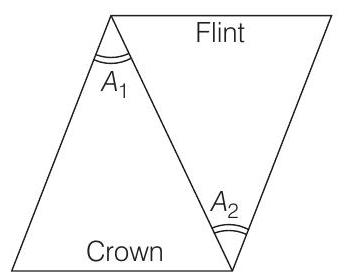
or $\quad\left(\mu _1-1\right) A _1+\left(\mu _2-1\right) A _2=0$
Here, $\mu _1$ and $\mu _2$ are the refractive indices for crown and flint glasses respectively.
Hence,
$$ \begin{aligned} & \mu _1=\frac{1.51+1.49}{2}=1.5 \\ & \mu _2=\frac{1.77+1.73}{2}=1.75 \end{aligned} $$
and
$A _1=$ Angle of prism for crown glass $=6^{\circ}$
Substituting the values in Eq. (i), we get
$$ (1.5-1)\left(6^{\circ}\right)+(1.75-1) A _2=0 $$
This gives $A _2=-4^{\circ}$
Hence, angle of flint glass prism is $4^{\circ}$ (Negative sign shows that flint glass prism is inverted with respect to the crown glass prism.)
(b) Net dispersion due to the two prisms is
$$ \begin{aligned} & =\left(\mu _{b _1}-\mu _{r _1}\right) A _1+\left(\mu _{b _2}-\mu _{r _2}\right) A _2 \\ & =(1.51-1.49)\left(6^{\circ}\right)+(1.77-1.73)\left(-4^{\circ}\right)=-0.04^{\circ} \end{aligned} $$
$\therefore$ Net dispersion is $-0.04^{\circ}$
