Optics 7 Question 18
18. Two thin convex lenses of focal lengths
(1993)
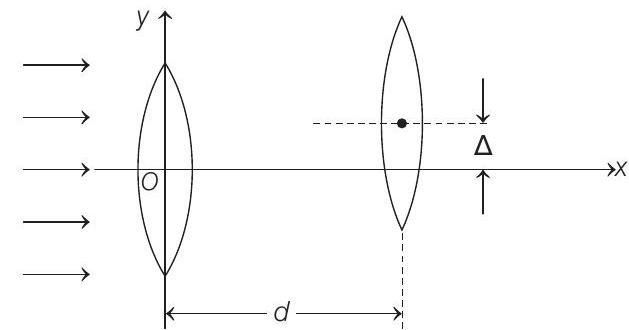
Taking the origin of coordinates,
(1993; 2M)
(a)
(b)
(c)
(d)
Numerical Value
Show Answer
Solution:
- From the first lens parallel beam of light is focused at its focus i.e. at a distance
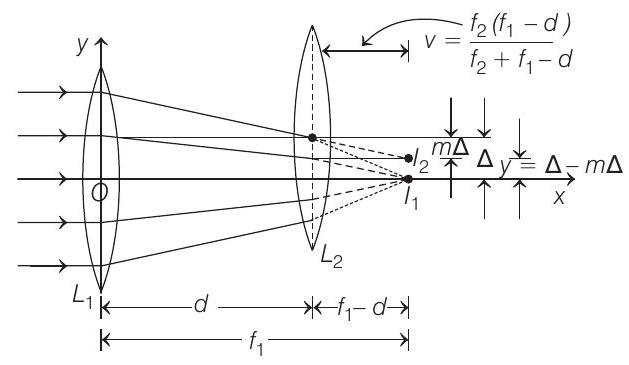
Therefore,
Linear magnification for
Therefore, second image will be formed at a distance of
Therefore,






