Optics 7 Question 12
12. A biconvex lens of focal length
(2010)
(a) virtual and at a distance of
(b) real and at a distance of
(c) virtual and at a distance of
(d) real and at a distance of
Show Answer
Solution:
- Object is placed at distance
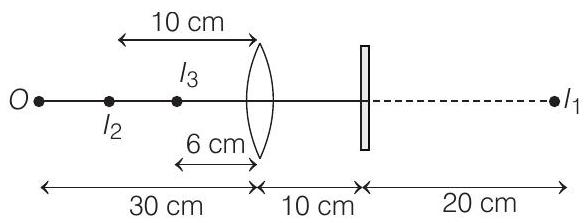
Now applying lens formula
Therefore, the final image is at distance
This is because ray of light is travelling from right to left.






