Optics 7 Question 10
10. Diameter of a plano-convex lens is $6 cm$ and thickness at the centre is $3 mm$. If speed of light in material of lens is $2 \times 10^{8}$ $m / s$, the focal length of the lens is
(2013 Main)
(a) $15 cm$
(b) $20 cm$
(c) $30 cm$
(d) $10 cm$
Show Answer
Solution:
- By Pythagoras theorem
$$ R^{2}=(3)^{2}+(R-0.3)^{2} \Rightarrow R \approx 15 cm $$
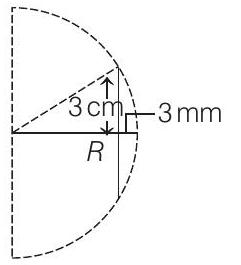
Refractive index of material of lens $\mu=\frac{c}{v}$
Here $c=$ speed of light in vacuum $=3 \times 10^{8} m / s$
$v=$ speed of light in material of lens $=2 \times 10^{8} m / s$
$$ =\frac{3 \times 10^{8}}{2 \times 10^{8}}=\frac{3}{2} $$
From lens maker’s formula
$$ \frac{1}{f}=(\mu-1) \frac{1}{R _1}-\frac{1}{R _2} $$
Here, $R _1=R$ and $R _2=\infty$ (For plane surface)
$$ \begin{aligned} & & \frac{1}{f}=\frac{3}{2}-1 \quad \frac{1}{15} \\ \Rightarrow & f & =30 cm \end{aligned} $$
