Optics 6 Question 50
52. A coherent parallel beam of microwaves of wavelength
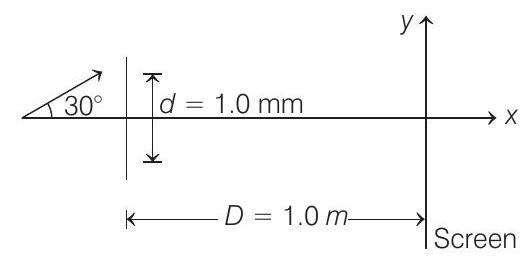 measured on a screen placed parallel to the plane of the slits at a distance of
measured on a screen placed parallel to the plane of the slits at a distance of
(a) If the incident beam falls normally on the double slit apparatus, find the
(b) If the incident beam makes an angle of
Show Answer
Answer:
Correct Answer: 52. (a)
Solution:
- Given,
(a) When the incident beam falls normally :
Path difference between the two rays
For minimum intensity,
or
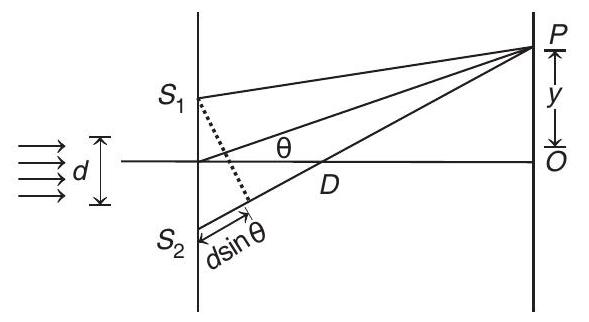
As
So,
When
or
So, the position of minima will be
And as minima can be on either side of centre
Therefore there will be four minimas at positions
(b) When
So, there is already a path difference of
Position of central maximum Central maximum is defined as a point where net path difference is zero. So,
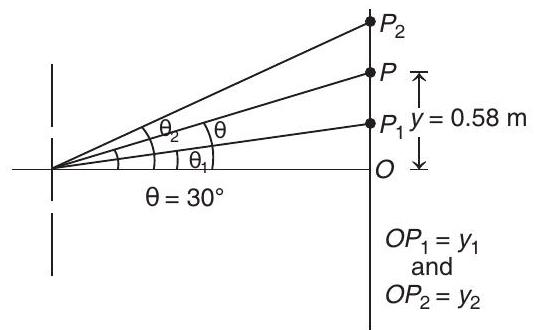
At point
Above point
Below point
Now, let
or
Similarly by for
Therefore,
NOTE In this problem






