Optics 3 Question 39
39. A plano-convex lens has a thickness of
(1984, 6M)
Show Answer
Answer:
Correct Answer: 39.
Solution:
- Refer figure (a)
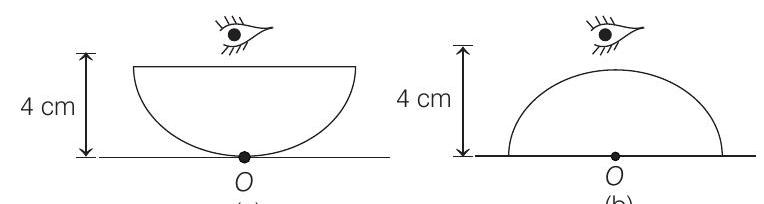
(a)
(b)
In this case refraction of the rays starting from
Refer figure (b) In this case refraction takes place from a spherical surface. Hence, applying
Now, to find the focal length we will use the lens Maker’s formula






