SATHEE: Optics 2 Question 28

Optics 2 Question 28

27. The $x - y$ plane is the boundary between two transparent media. Medium-1 with $z \geq 0$ has a refractive index $\sqrt{2}$ and medium-2 with $z \leq 0$ has a refractive index $\sqrt{3}$ . A ray of light in medium-1 given by vector $A = 6 \sqrt{3} \hat{i} + 8 \sqrt{3} \hat{j} - 10 \hat{k}$ is incident on the plane of separation. Find the unit vector in the direction of the refracted ray in medium-2.

(1999, 10 M)

Show Answer

Answer:

Correct Answer: 27. $\frac{1}{5 \sqrt{2}} (3 \hat{i} + 4 \hat{j} - 5 \hat{k})$

Solution:

Incident ray $A = 6 \sqrt{3} \hat{i} + 8 \sqrt{3} \hat{j} - 10 \hat{k}$

$\begin{aligned} = (6 \sqrt{3} \hat{i} + 8 \sqrt{3} \hat{j}) + (- 10 \hat{k}) \\ = Q O + P Q (As shown in figure) \end{aligned}$

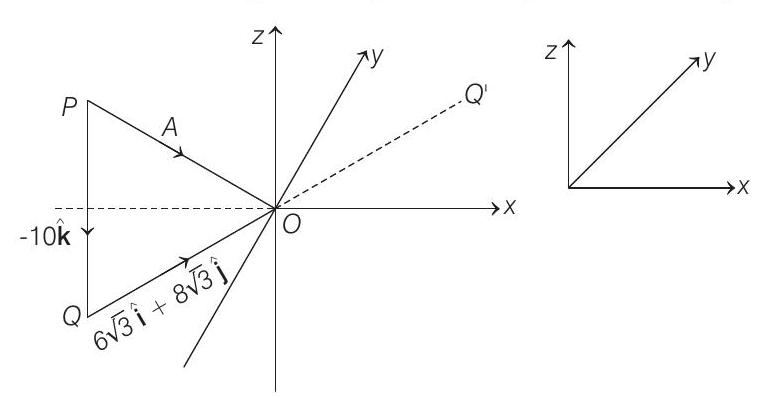
Note that $Q O$ is lying on $x - y$ plane.

Now, $Q Q^{'}$ and $Z$ -axis are mutually perpendicular. Hence, we can show them in two-dimensional figure as below.

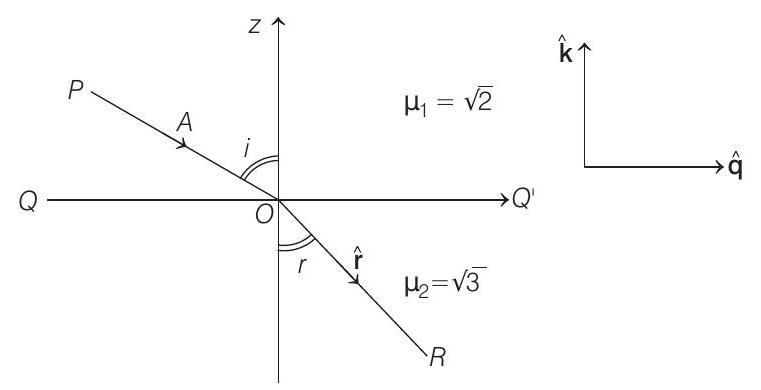
Vector A makes an angle $i$ with $z$ -axis, given by

$\begin{aligned} i = \cos^{- 1} \frac{10}{\sqrt{(10)^{2} + (6 \sqrt{3})^{2} + (8 \sqrt{3})^{2}}} = \cos^{- 1} \frac{1}{2} \\ i = 60^{\circ} \end{aligned}$

Unit vector in the direction of $Q O Q^{'}$ will be

$\begin{matrix} \hat{q} = \frac{6 \sqrt{3} \hat{i} + 8 \sqrt{3} \hat{j}}{\sqrt{(6 \sqrt{3})^{2} + (8 \sqrt{3})^{2}}} \\ = \frac{1}{5} (3 \hat{i} + 4 \hat{j}) \end{matrix}$

Snell’s law gives

$\begin{aligned} \frac{\sqrt{3}}{\sqrt{2}} & = \frac{\sin i}{\sin r} = \frac{\sin 60^{\circ}}{\sin r} \\ ∴ & \sin r & = \frac{\sqrt{3} / 2}{\sqrt{3} / \sqrt{2}} = \frac{1}{\sqrt{2}} \\ ∴ & r & = 45^{\circ} \end{aligned}$

Now, we have to find a unit vector in refracted ray’s direction $O R$ . Say it is $\hat{r}$ whose magnitude is 1 . Thus,

$\begin{aligned} \hat{r} & = (1 \sin r) \hat{q} - (1 \cos r) \hat{k} \\ = \frac{1}{\sqrt{2}} [\hat{q} - \hat{k}] = \frac{1}{\sqrt{2}} \frac{1}{5} (3 \hat{i} + 4 \hat{j}) - \hat{k} \\ \hat{r} & = \frac{1}{5 \sqrt{2}} (3 \hat{i} + 4 \hat{j} - 5 \hat{k}) . \end{aligned}$