Optics 2 Question 25
25. A monochromatic light is travelling in a medium of refractive index $n=1.6$. It enters a stack of glass layers from the bottom side at an angle $\theta=30^{\circ}$. The interfaces of the glass layers are parallel to each other. The refractive indices of different glass layers are monotonically decreasing as $n _m=n-m \Delta n$, where $n _m$ is the refractive index of the $m$ th slab and $\Delta n=0.1$ (see the figure). The ray is refracted out parallel to the interface between the $(m-1)$ th and $m$ th slabs from the right side of the stack. What is the value of $m$ ?
(2017 Adv.)
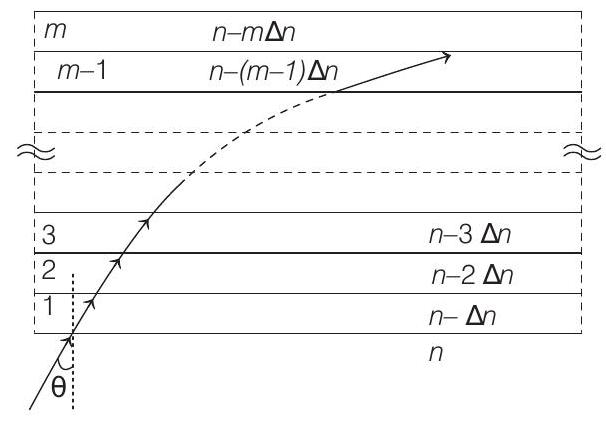
Analytical & Descriptive Questions
Show Answer
Answer:
Correct Answer: 25. 8
Solution:
- But this value of refractive index is not possible.
$$ \begin{aligned} & 1.6 \sin \theta=(n-m \Delta n) \sin 90^{\circ} \\ & 1.6 \sin \theta=n-m \Delta n \end{aligned} $$
$$ \begin{aligned}
