Modern Physics 7 Question 81
11. In a line of sight radio communication, a distance of about 50
(Radius of the earth
(a)
(b)
(c)
(d)
(Main 2019, 8 April II)
Show Answer
Solution:
- Key Idea In line of sight communication, distance
Here in figure,
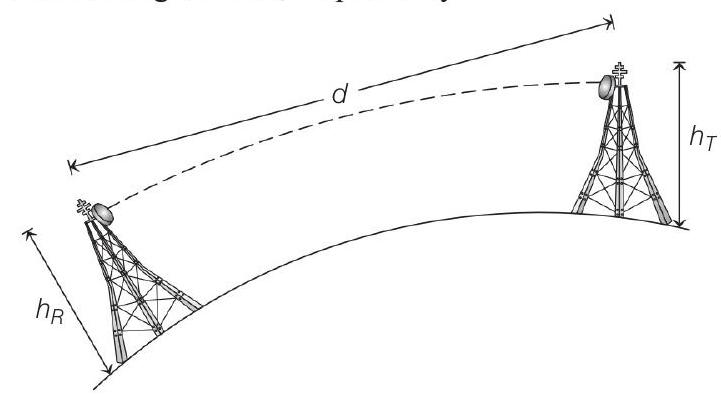
Given,
Then, distance between transmitting and
receiving antenna, i.e.






